- #1
knowLittle
- 312
- 3
Homework Statement
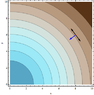
An artificial hill has altitude given by the function A(x,y)=300e^-(x^2 +y^2)/100 where the positive y-axis points north and the positive x-axis points east.
a.)What would be the instantaneous rate of change of her altitude if she walks precisely northwest, starting from the point (8,6)?
b.) Which way should she start walking to go downhill most rapidly from the point (8,6) and what would the rate of change of her altitude be if she walked in that direction?
c.) Which ways could she start walking to stay at a constant altitude, starting from (8,6)?
(Note that of course she is in a location with 3 coordinates, so I'm specifying only her x and y coordinates in this problem.)
Could you review all my answers?
Homework Equations
Directional derivatives.
The Attempt at a Solution
Problem 3a., the "instantaneous rate of change of her altitude if she walks precisely northwest, starting from the point (8,6)?" can be interpreted as find the directional derivative of A(x,y) at (8,6) in the direction of u= -cos(pi/4)i +sin(pi/4)j, since Northwest would be 135 degrees.
Ax (8,6)=-6xe^(-x^2 -y^2)/100 =-48e^-1
Ay(8,6)=-6ye^(-x^2 -y^2)/100=-36e^-1
D_u A(x,y)=(-48e^-1)(-cos(pi/4)i)+(-36e^-1)(sint(pi/4)j ), D as in directional derivative.
I found that D= 3.121560451
Problem 3b.)
Which way should she start walking to go downhill most rapidly from the point (8,6)?
Downhill most rapidly from (8,6) means the -|gradA|
gradA(x,y)=Ax(x,y)i+Ay(x,y)j
gradA(x,y)=-48e^(-1)i -36e^(-1)j, she should start walking in this direction to go uphill most rapidly and -gradA(x,y) to go downhill most rapidly.
Namely,
48e^(-1)i +36e^(-1)j
what would the rate of change of her altitude be if she walked in that direction?
Rate of change of her altitude would be |gradA(x,y)|=22.07276647
Problem c.)
Which ways could she start walking to stay at a constant altitude, starting from (8,6)?
(Note that of course she is in a location with 3 coordinates, so I'm specifying only her x and y coordinates in this problem.)
Domain of x and y are all reals, but z=A(x,y) can only be [0,300]
So there are only upwards<110.363824, 300]
and downwards from <110.36824, 0] ways.
Thank you