- #1
Transstars
- 3
- 0
Let's say there is two tubes(cylinders with no tops or bottoms) with charges ##q_1## and ##q_2##, radii ##b_1## and ##b_2##, lengths ##l_1## and ##l_2##. These tubes are located along the axis of each other's surfaces like in this figure:
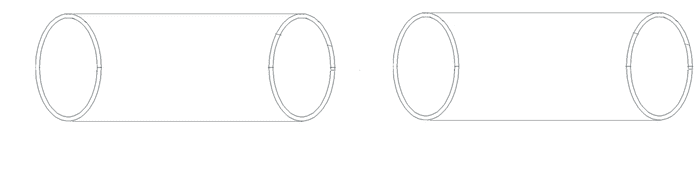
I know I have to use Coulomb's law rather than Gauss law since the tubes are along the axis of each other's surfaces.The question is how can I calculate the force between these two tubes? If I have to integrate something please show me how to do it since I have never taken a course on calculus.
Thanks in advance.
P.S: I asked this question on another platform and I got that answer but I don't know how to use integral so I would be grateful if someone could make the equations that is mentioned in the answer below.
" 1. Start with the force of two point charges: you know this equation
$$F=\frac{Q_1Q_2}{4\pi\epsilon_0 r^2}$$
2. Integrate this force over an infinitesimally thin ring of charge: now you have the force of a ring on an off-axis point (hint: you only need the axial component - the radial components will cancel due to symmetry in the next step). The distance $R$ will be a function of position along the ring (since the point charge is off-axis)
3. Integrate over all possible points that constitute a second ring: now you have the force of one ring on another; with the two rings on the same axis, the force will be along that axis. This is easy since the axial force is the same everywhere (so no difficult integration needed - just multiply by $2\pi$ and take account of the "charge per unit length").
4. Integrate over the length of the first cylinder: now you have the force of a cylinder on a ring. This is a bit harder - you are in essence integrating the force over a series of rings of variable (axial) distance
5. Integrate over the second cylinder: this is the sum of the force between a cylinder and a series of rings of different distance to the cylinder.
Note that your expression for the on-axis force of the cylinder is not terribly helpful since the charges of the second cylinder are off-axis."
I know I have to use Coulomb's law rather than Gauss law since the tubes are along the axis of each other's surfaces.The question is how can I calculate the force between these two tubes? If I have to integrate something please show me how to do it since I have never taken a course on calculus.
Thanks in advance.
P.S: I asked this question on another platform and I got that answer but I don't know how to use integral so I would be grateful if someone could make the equations that is mentioned in the answer below.
" 1. Start with the force of two point charges: you know this equation
$$F=\frac{Q_1Q_2}{4\pi\epsilon_0 r^2}$$
2. Integrate this force over an infinitesimally thin ring of charge: now you have the force of a ring on an off-axis point (hint: you only need the axial component - the radial components will cancel due to symmetry in the next step). The distance $R$ will be a function of position along the ring (since the point charge is off-axis)
3. Integrate over all possible points that constitute a second ring: now you have the force of one ring on another; with the two rings on the same axis, the force will be along that axis. This is easy since the axial force is the same everywhere (so no difficult integration needed - just multiply by $2\pi$ and take account of the "charge per unit length").
4. Integrate over the length of the first cylinder: now you have the force of a cylinder on a ring. This is a bit harder - you are in essence integrating the force over a series of rings of variable (axial) distance
5. Integrate over the second cylinder: this is the sum of the force between a cylinder and a series of rings of different distance to the cylinder.
Note that your expression for the on-axis force of the cylinder is not terribly helpful since the charges of the second cylinder are off-axis."
Last edited by a moderator: