How can the end of a rod accelerate faster than g?

[Happiness]

A uniform rod rests on supports at its ends. The right support is quickly removed.

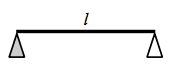
The atom on the right end will accelerate faster than $g$ at $1.5g$. How is this possible forces-wise? The only forces acting on the atom is its own weight and the interatomic forces of attraction, which acts horizontally (left) at the instant the right support is removed. So the net force on the atom doesn't seem to be able to make it accelerate faster than g.

 

[Doc Al]

The only forces acting on the atom is its own weight and the interatomic forces of attraction, which acts horizontally (left)

Why do you think those interatomic forces act horizontally?

 

[Doc Al]

Try this: Imagine a small piece of the rod that is just above the right support. What forces act on it?

 

[Happiness]

Why do you think those interatomic forces act horizontally?

Because the rod is horizontal at first.

Suppose we picture the rod to be made of N horizontal rows of atoms. We may group each vertical column of N atoms as a "super-atom". Then these super-atoms are horizontally next one another. So the forces between them are assumed to be horizontal.

 

[Doc Al]

Because the rod is horizontal at first.

Suppose we picture the rod to be made of N horizontal rows of atoms. We may group each vertical column of N atoms as a "super-atom". Then these super-atoms are horizontally next one another. So the forces between them are assumed to be horizontal.

Just because they are arranged horizontally does not mean that the forces are horizontal. (See the question in my last post.)

 

[Happiness]

Try this: Imagine a small piece of the rod that is just above the right support. What forces act on it?

Suppose the rod is two-atom thick. Let $M$ and $m$ be the masses of the rod and of each atom respectively. Before the right support is removed, there is an upward force of $\frac{1}{2}Mg$ acting on the rightmost column of atoms. The total weight of this column is $2mg$. Thus to keep it in equilibrium, the adjacent column must be acting on it a downward force of $F=\frac{1}{2}Mg-2mg$. When the right support is removed, the net force acting on it will be $F+2mg=\frac{1}{2}Mg$. Its acceleration will thus be $\frac{Mg}{4m}$. But this is not necessarily $1.5g$. What's wrong?

 

[Doc Al]

The point of that exercise was not to solve for the acceleration, but just to point out that one section of the rod must be exerting vertical forces on the other.

To solve for the acceleration of the end of the rod we'd just examine the torque on the rod. (The downward force on that end piece would change once the support is removed.)

 

[Dale]

So the forces between them are assumed to be horizontal.

This is not correct.

You are thinking of a rope. An ideal rope can only support tension, meaning a force along the rope. However a rod can support shear stress as well, meaning forces perpendicular to the rod.

The end of the rod falls down faster than g because there is a downward shear force as well as the downward gravitational force.