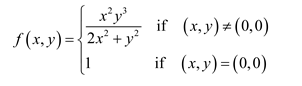How can we determine the set of points at which a function is continuous?

[ineedhelpnow]

im lost. can someone explain how to do this problem?
determine the set of points at which the function is continuous
View attachment 3360

 

[alyafey22]

Is it true

$$\lim_{(x,y)\to (0,0)} \frac{x^2y^3}{2x^2+y^2} = 1$$

 

[ineedhelpnow]

i am not sure

- - - Updated - - -

i think its not

 

[alyafey22]

i am not sure

Any path through The origin should result in $1$. Try the path $y=x$. Does it result in $1$ ?

 

[ineedhelpnow]

no.

 

[ineedhelpnow]

i got 0. is that wrong?

 

[MarkFL]

i got 0. is that wrong?

W|A can tell you if you are wrong or not, but how did you obtain this value? (Thinking)

 

[ineedhelpnow]

did f(x,x) since i put in x for y and then i simplified and took the limit of that as it approaches 0 (plugged it into my calc). i got 0. unless i did something wrong.

 

[alyafey22]

did f(x,x) since i put in x for y and then i simplified and took the limit of that as it approaches 0 (plugged it into my calc). i got 0. unless i did something wrong.

So the value of the limit is not equal to the value of the function, what can you conclude ?

 

[ineedhelpnow]

thats what i don't understand. my book uses this delta epsilon stuff for it and i was never properly taught the delta epsilon proof in calc 1 so i don't understand this problem at all. and sometimes my teacher uses the squeeze theorem for these problems which i also don't know how to use that well. i know what they both say but i don't get how to use them.

 

[Dethrone]

Squeeze theorem is simple.

Say you have:
$$\lim_{{x}\to{0}}x^2\sin\left({\frac{1}{x^2}}\right)$$

We know that $-1\le\sin\left({x}\right)<1$, and so you just want to manipulate it so that function mirrors the one given...

$$-1\le \sin\left({\frac{1}{x}}\right)\le1$$
$$-x^2\le x^2 \sin\left({\frac{1}{x}}\right)\le x^2$$

We know that as $x$ approaches 0, then the outer functions of the inequality also goes to zero. Because $x^2\sin\left({\frac{1}{x}}\right)$ is always between the two outer functions, it must also go to zero (which doesn't necessary mean that it is defined at 0)

 

[ineedhelpnow]

i get the idea of it but like how would i implement it in a problem like this. i know this problem may not be that difficult but my instructor wants proof for the solution to this problem.

 

[alyafey22]

i get the idea of it but like how would i implement it in a problem like this. i know this problem may not be that difficult but my instructor wants proof for the solution to this problem.

There is nothing to prove. The only discontinuity can happen at the origin and since the value of the function is not equal to the limit at the origin, the function is not continuous at that particular point.

 

[ineedhelpnow]

im looking at how it was done with the squeeze theorem but it makes no sense.

 

[Nono713]

If you really need to use deltas and epsilons, you could proceed by showing (in the language of deltas and epsilons, of course) that despite the fact that we can make $(x, y)$ arbitrarily close to $(0, 0)$ (by making it equal $(x, x)$ as $x$ tends to zero), the distance from $f((x, x))$ to $f((0, 0)) = 1$ as $x \to 0$ does not get arbitrarily small, since the latter equals one while the former converges to zero. And as a result the function fails to be continuous at $(0, 0)$ by definition of epsilon-delta continuity.

Does that make it clearer?​

 

[Dethrone]

I'll have an attempt at this problem now, most likely for future readers. I will first prove that the limit exists by using two methods, the first being an epsilon delta proof and the second with squeeze theorem. Then, since we find that the limit at the origin does not equal to value of the function, it does not satisfy the conditions of being continuous at the origin.

The squeeze theorem is quite straightforward to apply most of the time using the property $x^2\le x^2+y^2$ or $y^2\le x^2+y^2$.
We have $$\lim_{{(x,y)}\to{(0,0)}}\frac{x^2y^3}{2x^2+y^2}$$, and we can deduce the following:
$$0\le \left| \frac{x^2y^3}{2x^2+y^2} \right|< \frac{x^2\left| y^3 \right|}{x^2+y^2} \le \frac{(x^2+y^2)\left| y^3 \right|}{x^2+y^2}=\left| y^3 \right|$$
As we take the limit as $(x,y)$ tends to $(0,0)$ on the right most inequality, by the squeeze theorem, $$\lim_{{(x,y)}\to{(0,0)}}\frac{x^2y^3}{2x^2+y^2}$$ must also equal zero. From here, we can say that the given piecewise function is not continuous at $(0,0)$Alternatively, we can use an epsilon delta proof.
For all $\epsilon>0$, there exists a $\delta$ such that whenever $\left| \text{x}-\text{a} \right|<\delta$, $\left| f(\text{x})-L \right|<\epsilon$, where $\text{x}=(x,y), \text{a}=(a,b)$, both vectors. In other words, whenever $\sqrt{(x-a)^2+(y-b)^2}<\delta, \left| f(x,y)-L \right|<\epsilon$.
In this problem, we have $\sqrt{x^2+y^2}<\delta$, $$\left| \frac{x^2y^3}{2x^2+y^2} \right|<\epsilon$$.

From above, we have shown that $$\left| \frac{x^2y^3}{2x^2+y^2} \right|<\left| y \right|^3=\left(x^2+y^2\right)^{3/2}$$. Set $\delta = \epsilon ^{1/3}$. So for any $\epsilon$, we have:

$\sqrt{x^2+y^2}<\delta =\epsilon ^{1/3}$
$(x^2+y^2)^{3/2}<\epsilon$
$$\frac{x^2y^3}{2x^2+y^2} <\epsilon$$, as required.
The limit exists and equals $0$, which is not equal to the function evaluated at $(0,0)$, therefore the function is not continuous at the origin.