Interpretation of the Heisenberg picture in QM

[Sonderval]

I was always a bit puzzled by the Heisenberg picture (not mathematically, I'm fine with that, but conceptually) - if a "state" describes a system, how can it not be time-dependent, if the system changes?
I just found an alternative way of looking at it which seems to make sense to me, but I'm not sure whether it really is correct. Here is the operator evolution equation (stolen from Wikipedia).

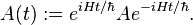
This will always be applied to some state |ψ> (so that the time evolution of the Schrödinger picture

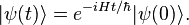
is recovered).
|ψ(0)> is the initial state of the system (and is the same in both pictures). So would it be acceptable to say that in the Heisenberg picture, I use the operators to contain the time evolution of the system and always apply them to the initial state? So |ψ> actually never enters the equation as a state at any time except at t=0 and acts only as initial conditions.

In other words: In the Schrödinger picture we have some initial condition (or initial state) and look at how this state evolves. In the Heisenberg picture, we plug the mathematics of the evolution into the operators and can then apply them always to the initial conditions. So it is not so much that the state does not change in time, but that we never have to look at the state at any time except t=0.

 

[vanhees71]

The point of the freedom to choose a picture is the following. You have states, represented by normalized state kets $|\psi \rangle$ (representing the corresponding ray in Hilbert space, which are the true representants of pure states), and (generalized) common eigenvectors of a complete set of compatible observables $|u(k) \rangle$, where $k$ is a label consisting of several discrete and/or continuous subsets of $\mathbb{R}^n$, where $n$ is the number of compatible observables determining the eigenstates uniquely. Neither the state kets nor the eigenvectors taken for themselves are observable but only the probabilities defined according to Born's Rule,
$$P_{\psi}(k)=|\langle u(k)|\psi \rangle|^2.$$
Accordingly you can pretty freely choose how the time dependence is distributed on the state kets and the eigenvectors of operators representing observables. This freedom boils down to a determination of the time dependence up to an arbitrary time-dependent unitary transformation.

The "extreme choices" are the Schrödinger picture: The entire time evolution is put to the state kets:
$$|\psi_S(t) \rangle=\exp(-\mathrm{i} \hat{H} t) |\psi_S(0) \rangle, \quad \hat{A}_j^{(S)}(t) = \hat{A}_j^{(S)}(0), \quad |u_S(k,t)=u_S(k,0)$$
and the Heisenberg picture: The entire time evolution is put to the eigenvectors
$$|\psi_H(t) \rangle=|\psi_H(0) \rangle, \quad \hat{A}_j^{(H)}(t)=\exp(\mathrm{i} \hat{H} t) \hat{A}_j^{(H)}(0) \exp(-\mathrm{i} \hat{H} t), \quad |u_H(k,t) \rangle=\exp(\mathrm{i} \hat{H} t) |u_H(k,0) \rangle.$$
Assuming that the operators and vectors coincide at $t=0$ (which you always can reach by a static unitary transformation) you have
$$|\psi_S(t) \rangle=\exp(-\mathrm{i} \hat{H} t) |\psi_H(t) \rangle \; \Rightarrow \; \hat{U}_{SH}(t)=\exp(-\mathrm{i} \hat{H} t).$$
Indeed that's consistent with
$$\hat{A}_S(t)=\hat{A}_H(0)=\hat{U}_{SH}(t) \hat{A}_H(t) \hat{A}_H(t) \hat{U}^{-1}_{SH}(t), \quad |u_S(k,t) \rangle=|u_H(k,0) \rangle=\hat{U}_{SH}(t) |u_H(t).$$
Sometimes a mixed picture of the time evolution is of advantaged. That's usually called a Dirac picture. A commonly used special case is the interaction picture in scattering theory.

 

[blue_leaf77]

Heisenberg and Schroedinger pictures in describing time evolution are physically equivalent, both stand on the same footing that is a time-independent Hamiltonian. The Heisenberg picture was originally invented as an effort to form a closer connection to classical mechanics. In classical mechanics, we don't talk about evolution of states (well, the state analogous to that in QM is not present in CM to begin with), instead, we talk about evolving observables such as position, momenta, angular momenta, etc, that is physical quantities as a function of time. Physical observables in QM are represented as operators, therefore if we allow operators to evolve in time like they do in CM, we might find a similar (at least mathematical) relations in QM. This approach is called Heisenberg picture.

So would it be acceptable to say that in the Heisenberg picture, I use the operators to contain the time evolution of the system and always apply them to the initial state?

That's indeed how Heisenberg picture was constructed.

So it is not so much that the state does not change in time, but that we never have to look at the state at any time except t=0.

Again, Schroedinger and Heisenberg picture differ from each other only in the way you view the definitions of states and operators. There is no urgent need to always associate a state to the reality, let your system evolve in time and you define the state associated with that system as the "agent" carrying the time information while the observables remain unchanged, then you are working in Schroedinger picture. On the other hand, if you define the state to be stationary and the operators to be the time-evolution "agent", then you are in the Heisenberg picture. It's just a matter of how you define your tools for your work. So in Heisenberg picture, again state is stationary because it's defined so.

One thing you may have to pay attention is that how the different definition in both pictures affect the definition of base kets. That's the set of vectors you will use to expand arbitrary state.

 

[zonde]

In other words: In the Schrödinger picture we have some initial condition (or initial state) and look at how this state evolves. In the Heisenberg picture, we plug the mathematics of the evolution into the operators and can then apply them always to the initial conditions. So it is not so much that the state does not change in time, but that we never have to look at the state at any time except t=0.

Not sure that it's always enough to look at t=0.
Say we have two different optical modes that produce interference. They can have different path length so that either they starting space-time points or ending space-time points are not the same. Usually you would chose that ending point is the same and that means that two modes will have to have different "time" due to different starting points.

 

[vanhees71]

It's not true that there are no states in classical theory. The "pure state" is a point in 6n-dimensional phase space (for a system of n point particles) and a mixed state is a probability density function $f(t;q,p)$ with $(q,p) \in \mathbb{R}^{6n}$.

 

[blue_leaf77]

The "pure state" is a point in 6n-dimensional phase space (for a system of n point particles) and a mixed state is a probability density function $f(t;q,p)$ with $(q,p) \in \mathbb{R}^{6n}$.

I'm not very familiar with phase space formulation in classical mechanics, but I can only imagine that this classical state is a collection of position coordinates and canonical momenta, which are a function of time, and which are none other than the quantities whose analog in QM are the observable operators.

 

[Sonderval]

@blue_leaf77
Thanks for the explanations.

There is no urgent need to always associate a state to the reality

That's exactly the point I am uncomfortable with intuitively (not mathematically, as I said) - I've often read a formulation like "In the Heisenberg pictures, the states are frozen in time".
In a sense, I feel that operators are something that you apply to a system, so the system should be there regardless of whether I operate on it or not. As an example, if I consider a wave packet that is traveling from A to B (in the Schrödinger picture) and I measure its position at different times (for example by trying to capture it in a box), intuitively it makes sense to me that the packet actually changes, whereas I find it difficult to say that the wave packet is always in its initial state but it is the box I use to capture it with that evolves over time. That's why I came up with the different interpretation of the state as an initial condition only - the position operator thena ctually consists of two parts: One to time-evolve the initial state to the current, the other to act on the current state (and then I need as athird part the hermitian conjugate on the left to be able to evaluate expectation values etc. relative to the initial state).
Possibly this is a consequence of me regarding the wave function as a kind of physical object (see also my answer to vanhees below).

@zonde
Sorry, I don't think I understand your example (not knowing much about optical modes). In any system it should be possible to formulate it in a way that starts from an initial condition at t=0 (or t=-∞), shouldn't it?

@vanhees
Thanks a lot for bringing that up. So would it make sense to you to formulate classical mechanics in a way where the point in phase space always stays at the same position and where the observables are the only things that evolve?

Actually, I looked at some original papers by Heisenberg, Jordan and Born yesterday - they never actually use the state in their equations and only calculate on the operators (as it is also frequently done in QFT).

 

[blue_leaf77]

As an example, if I consider a wave packet that is traveling from A to B (in the Schrödinger picture) and I measure its position at different times (for example by trying to capture it in a box), intuitively it makes sense to me that the packet actually changes, whereas I find it difficult to say that the wave packet is always in its initial state but it is the box I use to capture it with that evolves over time.

When you are talking about measurement, it makes more sense to say things in terms of probability of finding rather than the states or operators alone. Let's say you detector is slim enough with thickness $\Delta x$, the probability to find the particle having the wavepacket $|\psi\rangle$in question at $x=x'$ would be $\int_{x'}^{x'+\Delta x} |\langle x|\hat{U}(t)|\psi \rangle|^2 dx$, this quantity is a function of time. (don't worry both pictures will lead to this same expression of probability). So it's not that your box detector will be moving during the measurement, rather it's the probability to find that particle in a fixed position that is evolving in time. However, be carefull when conducting such thought experiment, as by placing a detector you have actually measured thing, and as consequence the wavepacket may have changed to another form. Therefore once you get the reading of the probability out of your detector, you shouldn't use that experiment again for measuring the probability at later times. Either perform another identical measurement or prepare initially identical wavepackets and do different measurements in parallel.

 

[Sonderval]

@blue_leaf77

the probability to find that particle in a fixed position that is evolving in time.

Yes - and it seems counterintuitive to me (and my question is all about the intuition/mental image; we do agree on the maths) to say that the probability evolves because it is the operator that changes over time.
In other words:
Standard wording to explain Heisenberg picture is "Operators evolve over time and act on a state that is frozen in time."
My preferred wording: "Operators act on the initial state that is evolved over time; the time evolutiuon is then made part of the operator to keep the maths simpler."

 

[Jilang]

Your intuition is leading you to identify the state with the particle and the measurement with the operator. As has been stated it doesn't have to be this way around. If it helps you might switch this around mentally by considering that it is the particle that operates on the measuring equipment.

 

[ChrisVer]

What difference do you find between me telling you: to get the vector $\begin{pmatrix} a \\ b \end{pmatrix}$ you have to multiply $\begin{pmatrix} 1 \\ 1 \end{pmatrix}$ with $\begin{pmatrix} a & 0 \\ 0 & b \end{pmatrix}$ or if I tell you that you have to multiply $\begin{pmatrix} a \\ b \end{pmatrix}$ with $\begin{pmatrix} 1 & 0 \\ 0 & 1 \end{pmatrix}$?

In the first case I took out the $a,b$ parameters from the vector and put them into the operator (in matrix representation here) , and in the second case I kept those parameters in the vector and left the operator independent of them...you could make $a,b$ dependent on $t$...that's a simple example I could think of...

 

[naima]

you can identify a state with the projection on this state. You see then that both pictures are on equal footing

 

[Sonderval]

@Jilang

If it helps you might switch this around mentally by considering that it is the particle that operates on the measuring equipment.

But we do not take the time evolution of the measuring equipment into account - it will have its own Hamiltonian which is different from that of the electron.

@ChrisVer and naima
As I said, I have no problem to see that the two pictures are mathematically equivalent, my problem is solely with the phrasing that "the state vector does not change". Probably this is just due to me identifying "state vector" to much with "state" (in the sense where a "state" of a system describes that system at a given time). My question is whether there is any physical reason that my alternative way of looking at the Heisenberg picture is wrong.

 

[ChrisVer]

The state of the QM system is not an observable (it's a multidimensional vector), so I don't understand why you are trying to apply a physical picture on that...The thing is that this "picture" think is just a unitary transformation/redefinition of your state...
What you actually care about are average values of observables, something that does not depend on the picture.

 

[vanhees71]

I'm not very familiar with phase space formulation in classical mechanics, but I can only imagine that this classical state is a collection of position coordinates and canonical momenta, which are a function of time, and which are none other than the quantities whose analog in QM are the observable operators.

This is a bit more subtle in my opinion. Indeed in classical mechanics the notion of "state" and "observable" is not as clearly separated as in quantum theory, because in classical mechanics indeed the state of the system is completely given by a point in phase space, and all observables then are functions of the phase-space variables (configuration space coordinates and their canonical momenta). So if you know the state of the system in classical mechanics completely, you know the positions and momenta (or equivalently the positions and generalized velocities) and thus the values of all possible observables.

This is not so in quantum theory, and the mathematical objects are quite far from what are observables. I'd never say the observables are self-adjoint operators in Hilbert space or the physical system is the statistical operator (as descriptor of the state). It's way more indirect: An observable is, both from the point of view classical as quantum theory, defined as a precise measurement process (or an equivalence class of such measurement processes) of this observable. A state is (an equivalence class) of preparation procedures, i.e., a description of how to prepare a system in a given state. Then the operators and Hilbert-space vectors are the mathematical way to express the probabilities for the outcome of measurements provided the system is prepared in some state. I don't see a direct connection between the abstract mathematical objects of the theory and what's called the observables and states of the systems experimentalists deal with. In other words, I'm a proponent of the minimal statistical interpretation and a strictly epistemic meaning of the quantum-theoretical notion of a system's state. I never could make sense out of more ontological interpretations, particularly when it comes to the collapse postulates which are quite unavoidable if you give an ontological interpretation to states, but than you open all the cans (or better said barrels!) of worms a la EPR!

 

[Jilang]

@Jilang

But we do not take the time evolution of the measuring equipment into account - it will have its own Hamiltonian which is different from that of the electron.

Is there normally such an evolution? How would you take it into account in the other picture? If there were such a time dependence that would introduce a second operator in the H picture which you could think of as operating on the equipment I suppose.

 

[blue_leaf77]

So if you know the state of the system in classical mechanics completely, you know the positions and momenta (or equivalently the positions and generalized velocities) and thus the values of all possible observables.

I guess the same is also true in QM, given a state of a system, we will know all possible values of any observable, provided we know how the state is represented in the space spanned by the eigenvectors of the observable in question.

 

[ChrisVer]

how would you know the positions and momenta in QM?
You would have to set up some uncertainty in their values, so their evolution in the phase-space would look like the evolution of a hypersurface (and not a point)

 

[blue_leaf77]

You would have to set up some uncertainty in their values

Indeed, we wouldn't get only one value of an observable with 100% probability.

 

[Sonderval]

@Jilang
If you consider the electron as measuring the equipment, then the time evolution operator should contain the Hamiltonian describing the equipment - so the two concepts are not equivalent (i.e., you cannot describe the standard equation for an expectation variable of an observable in the Heisenberg picture as "the electron measuring the equipment", because then the state vector of the equipment and its Hamiltonian should be involved.)

@vanhees71
Yes, if you use this minimalist interpretation and do not consider the wave function (or state) as a "real" object, then of course it does not matter either way - the state is an abstract entity anyway. I'm not too fond of thinking this way, though (although I am aware that ascribing "reality" to the wave function leads to nasty problems like simultaneity of the collapse etc.)

 

[Jilang]

You are mixing up terms here. I am sorry if I didn't explain myself correctly. I never said that you should equate the particle with the measuring equipment! Just that you might consider that the operator corresponds to the particle, rather than to the measurement. In that case there would generally be no time evolution of the state (as the measuring equipment is generally a pretty static affair). If you required the equipment to have some sort of time evolution though it wouldn't be a problem, it would just add another term to the calculation. (That term being an operator acting in the state of the equipment).

 

[Sonderval]

@Jilang
Sorry, then I completely misunderstood your point. So in this view, the state of the particle is time-independent, but the considered observable (like position, momentum) evolves in time. I agree. I think this could be rephrased as "we consider the initial state of the particle together with the evolution of its observables".
Basically, it seems that that's all I'm asking about: Is it permissible to replace the phrase "state" with "initial state" in the Heisenberg picture? Mathematically, the answer is obviously "yes" because they are the same Hilbert space vectors. Conceptually, I see a difference, but this may be due to me considering the state vector as "the description" of the system (which is probably more a matter of taste than anything else).

 

[vanhees71]

Again, the choice of the picture of time evolution is in the theory. It has no immediate meaning for the association of the abstract objects with the physical system (including the measurement apparatus). The meaning of the abstract objects is given by Born's Rule, and the observable quantities as predicted by QT is independent of the picture of time-evolution. One should not mix these things up somehow.

 

[Jilang]

@Jilang
Sorry, then I completely misunderstood your point. So in this view, the state of the particle is time-independent, but the considered observable (like position, momentum) evolves in time.

No, you are still equating the particle with the state. I can sympathise as I've been here and it's tricky if you are well grounded in the other picture.

In this view the particle still has the time evolution and the operator has the time evolution. So consider the particle as the operator rather than the state. The measuring equipment is time independent and so is the state so consider the measuring equipment as the state. The observable is a function of both when the operator acts on the state.

 

[vanhees71]

Again! A particle is not (and in no picture of time evolution whatsoever) a Hilbert-space vector and its observables are no self-adjoint operators on the Hilbert space. There are real things in the lab called measurement apparati and a particle source but no mathematical abstract objects. The link to associate the mathematical abstract objects of the theory and the real-world equipment is Born's Rule, associating the modulus squared of a wave function with the probabilities and/or probability densities of the occurance of measurement results, and these probailities (and derived quantities from them like expectation values of observables or scattering cross sections and the like) are independent of the picture chosen. Any attempt to associate real-world equipment directly with the abstract objects of the QT formalism leads to confusion and are thus of no help!

 

[atyy]

Again! A particle is not (and in no picture of time evolution whatsoever) a Hilbert-space vector and its observables are no self-adjoint operators on the Hilbert space. There are real things in the lab called measurement apparati and a particle source but no mathematical abstract objects. The link to associate the mathematical abstract objects of the theory and the real-world equipment is Born's Rule, associating the modulus squared of a wave function with the probabilities and/or probability densities of the occurance of measurement results, and these probailities (and derived quantities from them like expectation values of observables or scattering cross sections and the like) are independent of the picture chosen. Any attempt to associate real-world equipment directly with the abstract objects of the QT formalism leads to confusion and are thus of no help!

Can you show an actual error if such an association is made?

 

[vanhees71]

I can't not even imagine how you'd make such and association, let allone which possible errors this might imply. Since physics should be independent of the representation, particularly the choice of the time-evolution picture, the only thing you could somehow think to associate with the particle (or any other entity under consideration) is the probability distribution of observables, given by the statistical operator of the system (determined by an appropriate preparation procedure), but then you run into the well-known problems which lead Born to his probabilistic interpretation of the wave function in the first place.

 

[atyy]

I can't not even imagine how you'd make such and association, let allone which possible errors this might imply. Since physics should be independent of the representation, particularly the choice of the time-evolution picture, the only thing you could somehow think to associate with the particle (or any other entity under consideration) is the probability distribution of observables, given by the statistical operator of the system (determined by an appropriate preparation procedure), but then you run into the well-known problems which lead Born to his probabilistic interpretation of the wave function in the first place.

Well, one just adds "FAPP". First, we make a classical/quantum cut, so we already say the wave function is not necessarily real. But once we have done that, I don't see any problem with saying that the observable to represent the apparatus FAPP, and the wave function to represents the system FAPP.

It's the same as simultaneity in classical special relativity. Simultaneity is not absolute, but it is absolute relative to a family of observers. So having chosen the family of observers, we can treat simultaneity as real FAPP - this merely involves using Newtonian-like intuition in a particular inertial coordinate system.

Similarly, the wave function can be taken to be real relative to a particular Heisenberg cut.

 

[Sonderval]

@Jilang

So consider the particle as the operator rather than the state.

O.k., I'm happy with that.
What I'm unhappy with is that in the standard phrasing the word "state" is used although the state does not fully describe the system at any point in time (except at the initial point). As far as I'm aware, everywhere else in physics (classical mechanics, thermodynamics), a "state" of a system describes the system at a certain time in point.
So I find it more clear to say that the "state" meant in the Heisenberg picture is the "initial state" (or initial condition), that the observables (operators) evolve (and the nice thing about the Heisenberg picture is that they evolve independently of the initial state, i.e., I can calculate the evolution of teh ooperators once and for all and then apply them to different initial states) and that to actually calculate any measurable quantity I of course need to apply the operator to the initial state. Would you say that this interpretation/phrasing is incorrect?
PS: I do agree that the association of the observable with the measurement apparatus only was highly problematic (if not so say, wrong...)

 

[atyy]

@vanhees71,all I mean is that in physics we do indeed have a intuitive notion of reality, so I agree with you that only that level is "truly real". But I think it is good to have the option to have some fake realities that help our intuition, which anyway may turn out to be real. For example, if it turns out that Lorentz invariance is broken at high enough energy, then although within special relativity, the absolute frame is observer dependent, in the new theory, the absolute frame could be real and reproduce all predictions that made us consider special relativity to be a superb theory.

Also, from mathematics and physics, we usually assume the natural numbers to "exist", whatever that means. But in fact all our prediction to date can be made with computers with finite memory, so it isn't obvious that we really need a theory in which infinitely large integers are possible. Maybe we could live with a theory with a largest integer (100^100^100^100). So in this sense the integers may not exist, but it's ok for us to act as if they do exist FAPP.

 

[vanhees71]

Well, but #29 just before your posting shows the confusion coming out of the idea to (wrongly) associate abstract mathematical entities of the theory with the real things in the lab. As a theoretician myself, I feel, it's quite important to look at a real lab from time to time and how "quantum things" are prepared and observed.

 

[atyy]

Well, but #29 just before your posting shows the confusion coming out of the idea to (wrongly) associate abstract mathematical entities of the theory with the real things in the lab. As a theoretician myself, I feel, it's quite important to look at a real lab from time to time and how "quantum things" are prepared and observed.

But that is in the Heisenberg picture, I think the OP does understand how to apply the Schroedinger and Heisenberg pictures to experiment, and also how to "interpret" the Schroedinger picture in a naive but helpful way in which the state is real FAPP.

I think he is only asking whether the Heisenberg picture also has a naive interpretation which is also helpful to the intuition. (As far as I know, it doesn't. If it does it is something like the system is unchanged, but what you consider a position measurement changes - but I think most people will not consider that helpful. Mathematically, of course the time evolution is just a rotation, and it doesn't matter if the system rotates and you keep still, or you rotate and the system keeps still.)

 

[vanhees71]

There is no difference in interpretation depending on the picture of time evolution used. So how can it make sense to associate the one or other mathematical element of the theory with the one or other physics piece of reality?

 

[atyy]

There is no difference in interpretation depending on the picture of time evolution used. So how can it make sense to associate the one or other mathematical element of the theory with the one or other physics piece of reality?

It depends on what one means by interpretation. If in Copenhagen, one is working at the level of true reality, then there is no difference in interpretation. But if in Copenhagen, one is working at the level of FAPP reality, then the state is the state in the sense of classical physics - it is the complete specification of of an individual system within the theory. And if one is trying to solve the measurement problem, then so far the Schroedinger picture seems privileged, since Bohmian Mechanics and Many-Worlds both privilege the Schroedinger picture.

It is a bit like in classical mechanics it doesn't matter whether we use Newtonian, Lagrangian or Hamiltonian formulations. However, in quantum mechanics, the Hamiltonian formulation is privileged. Even if one were to argue for the Lagrangian formulation via path integrals, the interpretation is dramatically changed, since the idea that the particle takes an extremal path is no longer true.

 

[Jilang]

@Jilang

O.k., I'm happy with that.
What I'm unhappy with is that in the standard phrasing the word "state" is used although the state does not fully describe the system at any point in time (except at the initial point). As far as I'm aware, everywhere else in physics (classical mechanics, thermodynamics), a "state" of a system describes the system at a certain time in point.
So I find it more clear to say that the "state" meant in the Heisenberg picture is the "initial state" (or initial condition), that the observables (operators) evolve (and the nice thing about the Heisenberg picture is that they evolve independently of the initial state, i.e., I can calculate the evolution of teh ooperators once and for all and then apply them to different initial states) and that to actually calculate any measurable quantity I of course need to apply the operator to the initial state. Would you say that this interpretation/phrasing is incorrect?
PS: I do agree that the association of the observable with the measurement apparatus only was highly problematic (if not so say, wrong...)

I think you are getting there (although you are still perhaps subconsciously identifying the state with the particle!), however it is misleading to identify observables with operators in any picture (as Vanhees pointed out). Obervables arise as a function of both the operator and the state. If the interpretation works for you, stick with it. It's only maths.