- #1
audifanatic51
- 10
- 0
Hi,
I've been asked to find the Laplace transform of a function and I have not the slightest clue where to begin. My professor derived the basic Laplace transforms in class(sin, cos, delta function, step function, etc), all of which I understood perfectly. However, he never really gave us an example of how to use those to find other Laplace transforms, let alone discuss how to approach the homework problem.
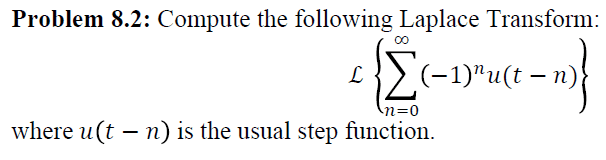
i made a few attempts. The first was by saying that the transform of a summation is the summation of the transforms. And then trying to take the transform of that, which seems ugly. I also tried graphing it and re-writing it as a series of step functions to get an idea, but I obviously can't do that until the end of time, so I'm stuck. Could somebody lead me in the right direction? Thanks!
I've been asked to find the Laplace transform of a function and I have not the slightest clue where to begin. My professor derived the basic Laplace transforms in class(sin, cos, delta function, step function, etc), all of which I understood perfectly. However, he never really gave us an example of how to use those to find other Laplace transforms, let alone discuss how to approach the homework problem.
i made a few attempts. The first was by saying that the transform of a summation is the summation of the transforms. And then trying to take the transform of that, which seems ugly. I also tried graphing it and re-writing it as a series of step functions to get an idea, but I obviously can't do that until the end of time, so I'm stuck. Could somebody lead me in the right direction? Thanks!