Chestermiller said:
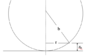
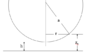
Yes. Let P be a horizontal plane tangent to the larger sphere b at its base. We wish to determine the elevation ##z_b## of the surface of b above the plane P as a function of the radial distance r measured from the vertical axis of the sphere. If we draw an appropriate right triangle in the diagram, we can use the Pythagorean theorem to show that $$(b-z_b)^2+r^2=b^2$$If we linearize this with respect to ##z_b##, we find that: $$z_b=\frac{r^2}{2b}$$Similarly, for the sphere a, we find that the elevation of its surface above the plane P (tangent to b) is
$$z_a=h+\frac{r^2}{2a}$$Therefore, the gap between the two surfaces H(r) is given by
$$H(r)=z_a-z_b=h+\frac{r^2}{2a}-\frac{r^2}{2b}$$
I am a bit confused as to why there is a minus sign in
H(r) (the answer for the force has a plus)
.
What I did is the following: if we position the spheres vertically, I thought of looking at the gap between the two spheres in a way that we move the bigger sphere upwards and the smaller one downwards. In that case:
$$ H(r) = z_{a} + z_{b} + h = h + \frac{r^2}{2a} + \frac{r^2}{2b}$$
If we move the bigger sphere upwards - that corresponds to the first part of the calculation you have indicated.
If I understood it correctly, you then lift the smaller sphere closer to the plane and I do not see how the distance to the plane P can be:
$$z_{a} = h +\frac{r^2}{2a}$$ which is bigger than
h (which I do not understand how this is possible: if we lift it by more than
h and then say that
H(r) = z_a - z_b that means the spheres will intersect). In addition, if we lift the smaller sphere upwards, I do not understand how we calculate the distance it was raised by. I would draw a right angle triangle having the origin of the smaller sphere as one of the vertices but then the hypotenuse will be leaving the radius of the sphere, making it difficult to find it.
If I proceed by lifting the bigger sphere and moving the smaller one downwards, as I mentioned before, I get:
$$ H(r) = z_{a} + z_{b} + h = h + \frac{r^2}{2a} + \frac{r^2}{2b}$$
Then, if I attempt to find the volume of fluid in that gap (i.e. right underneath the spheres), the volume that the fluid occupies looks like a frustum cone. Then using its formula for the volume, I find:
$$ V = \frac{\pi H(r)}{3} (a^2 + b^2 +ab) $$
However, I do not see why we need to find this volume and how it goes into calculating the resisting force since for that I would suppose we need change in pressure.
I hope it makes sense what I am trying to say - I am just trying to understand the procedure.