I Mapertius's Principle and 2nd Newton's law

  • I
  • Thread starter Thread starter MP97
  • Start date Start date
  • Tags Tags
    Newton's law
Click For Summary
The discussion focuses on deriving the second Newton's law using the Mapertius principle in the context of perfectly elastic collisions and free fall acceleration. The original poster is encountering a persistent issue with obtaining a coefficient of 2 in their final calculations. Participants are asked to provide insights or explanations for this discrepancy. The conversation aims to clarify the application of principles and calculations involved in the derivation process. Assistance is sought to resolve the confusion surrounding the coefficient.
MP97
Messages
4
Reaction score
0
Hi, I have been working on deriving the second Newton's law from the Mapertius principle applied to a perfectly elastic collision from a free fall acceleration problem. These are my calculations, but I keep getting a coefficient of 2 in my final answer for some reason. Could someone explain to me what I am doing wrong?

IMG_3194.jpg
IMG_3195.jpg
IMG_3196.jpg


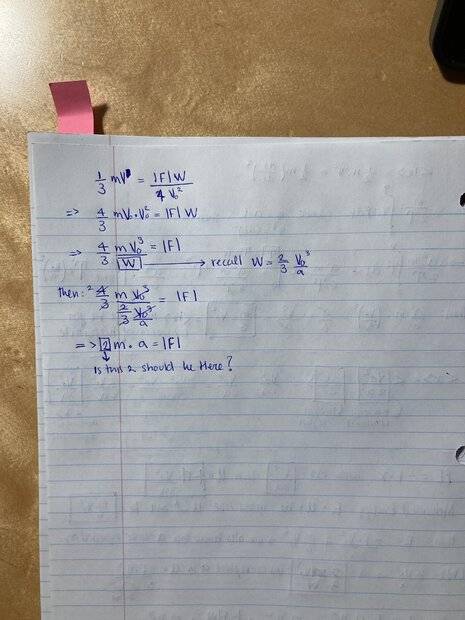
I appreciate any help you can provide!
 

Thread 'Please Explain Elementary Physics Elevator Question'

Hello, I'm joining this forum to ask two questions which have nagged me for some time. I am in no way trolling. They both are presumed obvious, yet don't make sense to me. Nobody will explain their positions, which is...uh...aka science. I also have a thread for the other question. Yes, I'm questioning the most elementary physics question we're given in this world. The classic elevator in motion question: A person is standing on a scale in an elevator that is in constant motion...
View full post »

Similar threads

I Spring balance analysis from a system point of view
Replies
24
Views
2K
I Newton's 2nd law for an object in free fall
Replies
15
Views
2K
I Are Newton's Laws just definitions?
Replies
11
Views
2K
I Weak principle of equivalence (Galileo, Newton)
Replies
5
Views
1K
B Action-reaction of a sailing boat and wind
Replies
8
Views
1K
I Is there an integral version of Newton's law of gravity?
Replies
10
Views
2K
I Work - Energy Principle Application to Fluid Flow
Replies
35
Views
4K
I Do objects of different mass but same size/shape accelerate the same?
Replies
1
Views
1K
I Work - Energy Principle Application to Fluid Flow
Replies
48
Views
5K
B How's it possible to push on systems with 2 parts? (Newton's third law)
Replies
14
Views
1K