- #1
unscientific
- 1,734
- 13
In the book 'Macroscopic and Statistical Thermodynamics' they derived the Maxwell-Boltzmann distribution by maximizing entropy using lagrangian multipliers with constants ##\alpha## and ##\beta##.
The final result is given as:
[tex] \frac{\overline {n_j}}{g_j} = e^{-\beta \epsilon_j}e^{-\alpha}[/tex]
where ##\overline {n_j}## is the occupation number and ##g_j## is the number of states of jth energy level.
After solving for ##e^{-\alpha} = \frac{N}{V}\left(\frac{h^2}{2\pi mkT}\right)^{\frac{3}{2}}## and integrating density of states to find ##g_j = \frac{V}{h^3} 4\pi p^2 dp##:
We obtain the maxwell-boltzmann distribution:
[tex]\overline {n_j} = \overline {n_{(p)}} dp = N 4\pi \left(\frac{\beta}{2\pi m}\right)^{\frac{3}{2}} p^2 e^{\frac{-p^2}{2mkT}} dp[/tex]
I obtain the correct speed distribution ##\propto p^2 e^{\frac{-p^2}{2mkT}}##, but What is the probability/fraction of finding a particle with momentum p?
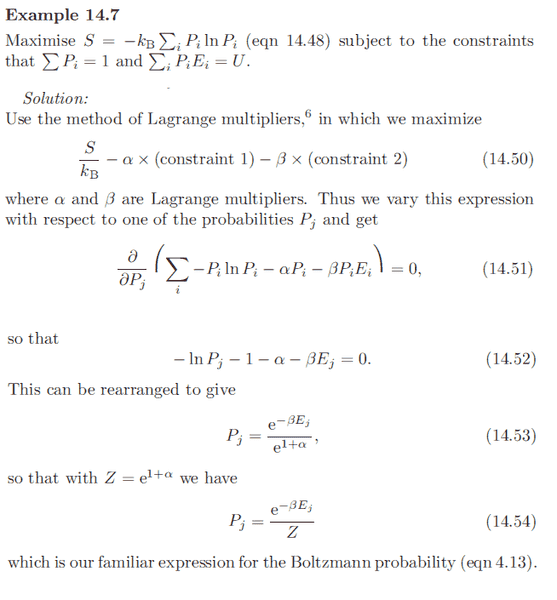
In Blundell's Book, a shorter approach is taken using gibbs' expression for entropy to find the Boltzmann probability:
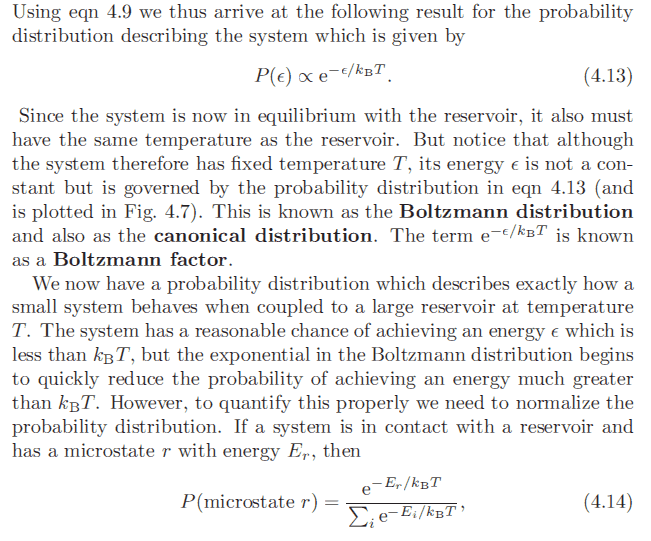
Here's the earlier reference to equation (4.13):
The final result is given as:
[tex] \frac{\overline {n_j}}{g_j} = e^{-\beta \epsilon_j}e^{-\alpha}[/tex]
where ##\overline {n_j}## is the occupation number and ##g_j## is the number of states of jth energy level.
After solving for ##e^{-\alpha} = \frac{N}{V}\left(\frac{h^2}{2\pi mkT}\right)^{\frac{3}{2}}## and integrating density of states to find ##g_j = \frac{V}{h^3} 4\pi p^2 dp##:
We obtain the maxwell-boltzmann distribution:
[tex]\overline {n_j} = \overline {n_{(p)}} dp = N 4\pi \left(\frac{\beta}{2\pi m}\right)^{\frac{3}{2}} p^2 e^{\frac{-p^2}{2mkT}} dp[/tex]
I obtain the correct speed distribution ##\propto p^2 e^{\frac{-p^2}{2mkT}}##, but What is the probability/fraction of finding a particle with momentum p?
In Blundell's Book, a shorter approach is taken using gibbs' expression for entropy to find the Boltzmann probability:
Here's the earlier reference to equation (4.13):
Last edited: