- #1
PhysicsBoi1908
- 50
- 12
- Homework Statement
- A uniform right angled triangular lamina is placed on a horizontal floor, which is not frictionless. One of the acute angles of lamina is theta. If F_A and F_B are the minimum forces required to rotate the lamina about stationary vertical axes through A and B respectively, then find the minimum force required to rotate the lamina about a stationary vertical axis passing through C.
- Relevant Equations
- τ=Iα
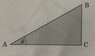
When the lamina rotates about A, FA must act on B (because it is the farthest away) perpendicular to AB (so that all of FA contributes to rotation).
Same argument is valid for rotation of lamina about B as well.
Having noted that, I tried two approaches:
Approach 1-

If I assume that the lamina has mass m, then maximum static friction becomes μmg. FC must act on A such that it is perpendicular to AC. Then I just have to equate FClcosθ=∫dfr, where l is the length of the hypotenuse.
I can find df by writing dfr=dmαr
where FClcosθ=ICα, where IC is the moment of inertia of the lamina about C.
There are a lot of problems with this approach:
I don't know IC, I couldn't calculate it.
If I evaluated FC this way, then I would have to repeat the process for FA and FB as well, which would make the solution very lengthy.
Approach 2-

While the last approach was, according to me, theoretically correct, I can't assure that for this one.
I argue that the minimum force required to turn A and B (and thus the lamina) about C must be the minimum force required to rotate lamina (and thus C) about A and B.
Then, the torque due to these forces about C must equal FClcosθ.
This approach dues give an answer, albeit the wrong one.
I think I know why the answer comes out to be incorrect. If FA or B is enough to cause rotation of A or B about C, then it can effectively rotate the lamina, and thus the answer must either by FA or B or something less than that.
Indeed, the correct answer is less than what I get from the above approach.
At this point, I have no more ideas.
(Note for approach one, I did find some videos online which derive moment of inertia of a triangular lamina, using "area moment" or something like that. But I would prefer to solve this question using high school physics, as the problem book expects me to.
Last edited: