Moment of inertia of cylinder with a rectangel shaped hole

[top12eaper]

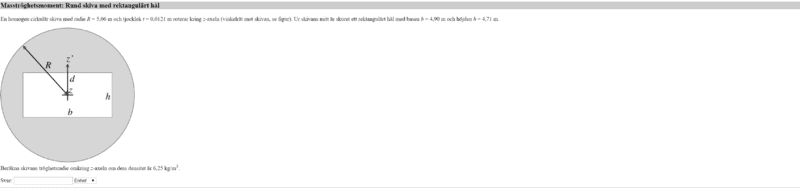
i know how to calculate the inersia for the cylinder, than i have to take away the inersia of the rectangel, but i can't find an equation for the inersia for a rectangel with the plane spinning perpendicular to the rotation axis, please help !

 

[sophiecentaur]

Which axis do you want?
Does this wiki page help? Have you looked anywhere else for this info?

 

[top12eaper]

Which axis do you want?
Does this wiki page help? Have you looked anywhere else for this info?

dont think so, they give me the equation for the inersia about the x and y axis, in my case the axis pierces the plane of the rectangel.

 

[Nidum]

Do you know how to do double integrals ?

If yes then we can derive the formula from first principles .

If no then :

Spoiler

http://www.engineersedge.com/polar-moment-inertia.htm

or

Spoiler