Path integral applied to circular path

[symmetric]

Homework Statement

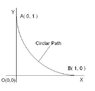
Consider path given by equation ( x - 1 )^2 + ( y - 1 ) ^2 = 1 that connect the points
A = ( 0 , 1 ) and B = ( 1 , 0 ) in xy plane ( shown in image attached ).
A bead falling under influence of gravity from a point A to point B along a curve is given by -

T = \int \frac{dt}{v}

where the bead's velocity is v = \sqrt{2gy} , where g is the gravitational constant.

Homework Equations

( x - 1 )^2 + ( y - 1 ) ^2 = 1

The Attempt at a Solution

Given curve ( x - 1 )^2 + ( y - 1 ) ^2 = 1 can be parametrized as -

x - 1 = \cos\Theta
y - 1 = \sin\Theta

curve can be written as

c( \Theta) = ( 1 + \cos\Theta , 1 + \sin\Theta )
c'( \Theta) = ( - \sin\Theta , \cos\Theta )
\left| c'( \Theta) \right| = 1

From given relation we have -

T = \int \frac{dt}{v}

T = \int_{c} \frac{ \left| c'( \Theta) \right| d\Theta}{ \sqrt{2gy}}

T = \int_{\pi}^{ \frac{3\pi}{2}} \frac{d\Theta}{ \sqrt{2g( 1 + \sin\Theta)}}


As, 1 + \sin\Theta = \left( \cos\frac{\Theta}{2} + \sin\frac{\Theta}{2} \right) ^2

T = \frac{1}{ \sqrt{2g}} \int_{\pi}^{\frac{3\pi}{2}} \frac{d\Theta}{ \cos\frac{\Theta}{2} + \sin\frac{\Theta}{2}}

Changing variables ,

\frac{\theta}{2} = x , d\Theta = 2 dx

T = \frac{1}{ \sqrt{2g}} \int_{\frac{\pi}{2}}^{\frac{3\pi}{4}} \frac{ 2 dx }{ \cos x + \sin x }


As, \sin\frac{\pi}{4} = \cos\frac{\pi}{4} = \frac{1}{\sqrt{2}}

multiplying Nr and Dr by \frac{1}{\sqrt{2}}

T = \frac{1}{\sqrt{g}} \int_{\frac{\pi}{2}}^{\frac{3\pi}{4}} \frac{dx}{ \sin\frac{\pi}{4}\cos x + \cos\frac{\pi}{4} \sin x }

T = \frac{1}{\sqrt{g}} \int_{ \frac{\pi}{2} } ^ {\frac{3\pi}{4}} \frac{dx} { \sin\left( x + \frac{\pi}{4} \right) }

T = \frac{1}{\sqrt{g}} \int_{\frac{\pi}{2}}^{\frac{3\pi}{4}} \csc \left( x + \frac{\pi}{4} \right) dx

T = \frac{1}{\sqrt{g}} \left| \ln \left[ \tan \left( \frac{ x + \frac{\pi}{4} } {2} } \right) \right] \right |_{\frac{\pi}{2}}^{\frac{3\pi}{4}}


The value of first term after limit substitution is infinity. Here the value of integral i.e. ' time ' should be strictly finite. What is wrong with my solution?

 

[vela]

Homework Statement

Consider path given by equation ( x - 1 )^2 + ( y - 1 ) ^2 = 1 that connect the points
A = ( 0 , 1 ) and B = ( 1 , 0 ) in xy plane ( shown in image attached ).
A bead falling under influence of gravity from a point A to point B along a curve is given by -

T = \int \frac{dt}{v}

where the bead's velocity is v = \sqrt{2gy}, where g is the gravitational constant.

"A bead is given by T=..." doesn't really make sense.

The problem is the integral you started with is wrong. You want a time, but if you check the units of the integral, you get time/(distance/time) = time²/distance.

 

[Hurkyl]

What does t have to do with the curve?

 

[tiny-tim]

Welcome to PF!

Hi symmetric! Welcome to PF!

(have a theta: θ and a pi: π and a square-root: √ and try using the X² tag just above the Reply box )

Nooo … your own notation is bad, and you've misunderstood the question's notation.

i] never use t for a distance parameter, use s (or x etc)

ii] you don't need to use the usual θ (starting with θ = 0 along the positive x-axis) … you can choose any distance parameter, so you may as well start with θ = 0 at A, going to θ = π/2 at B

iii] the y in the given formula, √2gy, is not the y-coordinate, it's the difference in height (so at A it's 0).

Try again. ​

 

[vela]

What does t have to do with the curve?

i] never use t for a distance parameter, use s (or x etc)

D'oh! I just assumed t stood for time. Just ignore my earlier post.

 

[symmetric]

@vela @Hurkyl @tiny-tim

Thanks for your reply.

To clear confusion of variable 't' attaching original problem snapshot.

What does t have to do with the curve?

From my understanding of given question it's distance traveled along circular path ( specifically for above problem ) .

i] never use t for a distance parameter, use s (or x etc)

ii] you don't need to use the usual θ (starting with θ = 0 along the positive x-axis) … you can choose any distance parameter, so you may as well start with θ = 0 at A, going to θ = π/2 at B

iii] the y in the given formula, √2gy, is not the y-coordinate, it's the difference in height (so at A it's 0).

Try again. ​

i] i generally don't use 't' for distance but given problem follows that notation .

ii] and iii] But we have to calculate transit time T for bead traveling along path given ( part of circle ). The given formula \sqrt{2gy} is valid for any path ( circular, straight line etc ) with same start and end point. Hence, given curve must be parametrized in order to get transit time T along specific path. If we chose the parametrization mentioned by me, then \Theta = 0 and \Theta = \frac{\pi}{2} will not leads to co-ordinates A(0,1) and B(1,0).