Does Rope Position Affect Acceleration in Rotational Movement?

[chimay]

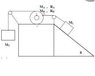
I refer to the figure:

Is it true that affixing the rope at the point A isn't the same of affixing it at the point B? (I consider the rotation movement around O since the disk is rolling, and so the radius of rotation is different in the two cases)
I'm sorry because my english is not very good, if something is not clear enough I'will give further explanations of the problem.

 

[zhermes]

What do you think? Why would they have the same effect? Why might they have different effects?

 

[chimay]

I thought so:


-the inertia moment respect the point O is $$I_o$$

-The distance BO (that is the radius of the circumference that is drescribed by the motion of rotation of the disk around O) is $$\sqrt{2R(R+r)}$$

The work of the inertial force of the equivalent mass is:$$I_o\alpha d \beta$$. Converting the rotational values into the respective traslational ones we have $$\frac{I_o adx}{2R(R+r)}$$
So it's not the same because the moment of the force is the same but the displacement depends on the radius.

 

[zhermes]

So far you're entirely correct.
At the first instant (i.e. the instant at which this image applies) the two situations are identical---the moment of the force is the same for both locations. As soon as the wheel starts to turn, the distance will be different for the two points, and the moment of the force will be different (when the wheel is at a different angle).

 

[chimay]

Ultimately, if the request of a problem of this kind is: calculate the acceleration of each corps of the figure. Affixing the rope in A or B gives us a different solution!

 

[chimay]

It was a question! So am I right?

 

[zhermes]

I think the instantaneous acceleration is the same.
After the first instance, however, the geometry will change and the moment-arm (the 'moment of the force') will be different for the two point (imagine that the wheel rotates just a tiny bit in either direction).
So I think it depends on if the question is asking about the very first instant, or in general.

English side note: 'body' can refer to basically any object or physical structure in general. 'Corps' refers specifically to a dead human-body.

 

[chimay]

Thanks for the english note too! :) However I posted the problem in the section "homework". The statement of the problem simply says: Calculate the acceleration of the bodies. I'd be glad if you could solve it in your free time..Anyway thanks a lot!