- #1
Lucas_30
- 5
- 0
Hello everyone, this is my first post, so go easy on me! Thank you to those who are able to help.
1. Homework Statement
The problem is taken from Professor Shankar's Fundamentals of Physics exercises (Problem 3).
Romeo is at x = 0 m at t = 0 s when he sees Juliet at x = 6 m
Romeo begins to run towards her at v = 5 m/s. Juliet, in turn, begins to accelerate towards him at a = −2 m/s 2
When and where will they cross?
My personal questions relate to:
1. My approach to the question as opposed to the given solution
2. Some of the algebra in the solution
Working from a position function of x(t) = 1/2at^2 + vot + xo (where a = constant acceleration; vo = initial velocity; xo = initial position)
I took known variables and formed:
x(romeo) = vot + xo (because we know Romeo's initial velocity (5m/s) and his initial position (0 m))
x(juliet) = 1/2at^2 + vot (because we know Juliet's acceleration (-2 m/s^2) and initial position (6m))
Prof. Shankar equates x(romero) to simply vt, as the position would be 0 at t = 0.
Would my way of forming x(romeo) and x(juliet) be correct?
Romeo and Juliet should cross when x(romeo) = x(juliet)
Giving according to my more verbose variables:
vot(r) + xo(r) = xo(j) + 1/2at^2(j)
To solve we would equate one side to 0 and "move across" the required variables, to get for example:
0 = 1/2at^2(j) - vot(r) + xo(j)
Does it matter which way the variables get "moved across"?
Solving 0 = 1/2at^2(j) - vot(r) + xo(j) with the given variables I get a result of:
-3.38600... (-3.0 s rounded to the nearest integer)
0.88600... (1.0 s rounded to the nearest integer)
Prof. Shankar's solutions state: 1 second exactly. Would my above solution be incorrect?
Here is his reasoning:
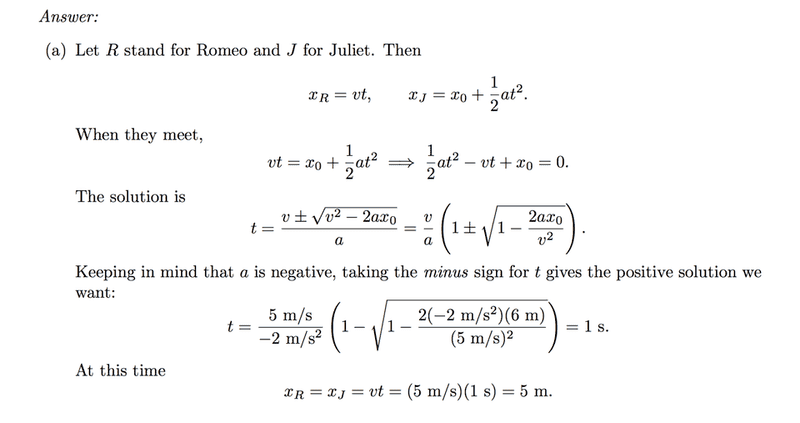
Would I be correct in saying that he has amended the quadratic equation to better fit the time function t = v2 - v1 / a?
Finally! I don't quite get the algebra behind v/a (1 +- ... ) Would someone be able to help me understand how he derived this from the initial equation?
I hope I've been clear. Thanks again to anyone that can help!
-- Lucas
1. Homework Statement
The problem is taken from Professor Shankar's Fundamentals of Physics exercises (Problem 3).
Romeo is at x = 0 m at t = 0 s when he sees Juliet at x = 6 m
Romeo begins to run towards her at v = 5 m/s. Juliet, in turn, begins to accelerate towards him at a = −2 m/s 2
Homework Equations
When and where will they cross?
My personal questions relate to:
1. My approach to the question as opposed to the given solution
2. Some of the algebra in the solution
The Attempt at a Solution
Working from a position function of x(t) = 1/2at^2 + vot + xo (where a = constant acceleration; vo = initial velocity; xo = initial position)
I took known variables and formed:
x(romeo) = vot + xo (because we know Romeo's initial velocity (5m/s) and his initial position (0 m))
x(juliet) = 1/2at^2 + vot (because we know Juliet's acceleration (-2 m/s^2) and initial position (6m))
Prof. Shankar equates x(romero) to simply vt, as the position would be 0 at t = 0.
Would my way of forming x(romeo) and x(juliet) be correct?
Romeo and Juliet should cross when x(romeo) = x(juliet)
Giving according to my more verbose variables:
vot(r) + xo(r) = xo(j) + 1/2at^2(j)
To solve we would equate one side to 0 and "move across" the required variables, to get for example:
0 = 1/2at^2(j) - vot(r) + xo(j)
Does it matter which way the variables get "moved across"?
Solving 0 = 1/2at^2(j) - vot(r) + xo(j) with the given variables I get a result of:
-3.38600... (-3.0 s rounded to the nearest integer)
0.88600... (1.0 s rounded to the nearest integer)
Prof. Shankar's solutions state: 1 second exactly. Would my above solution be incorrect?
Here is his reasoning:
Would I be correct in saying that he has amended the quadratic equation to better fit the time function t = v2 - v1 / a?
Finally! I don't quite get the algebra behind v/a (1 +- ... ) Would someone be able to help me understand how he derived this from the initial equation?
I hope I've been clear. Thanks again to anyone that can help!
-- Lucas