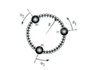
- #1
Wavefunction
- 99
- 4
Homework Statement
a) Write down the Lagrangian and show that the equations of motion are:
m\ddot{θ_1}+k[2θ_1-θ_2-θ_3] = 0
m\ddot{θ_2}+k[2θ_2-θ_1-θ_3] = 0
m\ddot{θ_3}+k[2θ_3-θ_1-θ_2] = 0
b) Show that the mode in which θ_1 = θ_2 = θ_3 corresponds to constant total angular momentum \vec{L}.
c) Assume that the total angular momentum is zero and that θ_1 = θ_2 = θ_3 = 0. Find two degenerate oscillatory modes and their frequency. Hint: you might want to express the three coupled equations as a single equation for the vector \vec{θ}, then assume that the eigenvectors of the tensor appearing in this equation have a time dependence e^{iωt} and find the values of ω for each eigenvector.
Homework Equations
eq(1)\frac{∂L}{∂q_j}-\frac{d}{dt}\frac{∂L}{∂\dot{q_j}}=0
The Attempt at a Solution
Part A
Step 1) The Lagrangian:
T = \frac{mR^2}{2}[\dot{θ_1}^2+\dot{θ_2}^2+\dot{θ_3}^2]
U = \frac{kR^2}{2}[θ_1^2+θ_2^2+θ_3^2-θ_1θ_2-θ_1θ_3-θ_2θ_3]
L = T-U = \frac{mR^2}{2}[\dot{θ_1}^2+\dot{θ_2}^2+\dot{θ_3}^2] - \frac{kR^2}{2}[θ_1^2+θ_2^2+θ_3^2-θ_1θ_2-θ_1θ_3-θ_2θ_3]
\frac{∂L}{∂θ_1} = -kR^2[2θ_1-θ_2-θ_3]
\frac{d}{dt}\frac{∂L}{∂\dot{θ_1}} = \frac{d}{dt}[mR^2\dot{θ_1}] = mR^2\ddot{θ_1}
-kR^2[2θ_1-θ_2-θ_3]-mR^2\ddot{θ_1}=0
eq(2) m\ddot{θ_1}+k[2θ_1-θ_2-θ_3]
Similarly for θ_2 and θ_3
eq(3) m\ddot{θ_2}+k[2θ_2-θ_1-θ_3]
eq(4) m\ddot{θ_3}+k[2θ_3-θ_1-θ_2]
Part B
Combining eqs(2,3,and 4) into a vector equation:
\ddot{\vec{θ}}+ω_0^2\begin{pmatrix} 2&-1&-1\\-1&2&-1\\-1&-1&2\end{pmatrix}\vec{θ}=\vec{0}
Let the above matrix be called \mathbf A
Now when θ_1= θ_2= θ_3 the matrix vector product \mathbf A\vec{θ}=\vec{0}
This in turn yields \ddot{\vec{θ}} = \vec{0} →\dot{\vec{θ}}=\vec{C} where \vec{C} is a constant vector.
Part C
First I'll need the eigenvalues and the resulting eigenvectors of \mathbf A which without too much trouble are:
λ_1=0 and a degenerate eigenvalue λ_2 = λ_3 = 3
Which corresponds to the eigenvectors which have the time dependence e^{iωt}:
\vec{e_1} = (1,1,1)e^{iωt}, \vec{e_2} = (-1,0,1)e^{iωt}, and \vec{e_3} = (-1,1,0)e^{iωt}
When \mathbf A acts on \vec{θ} it produces two equations:
eq(5) \ddot{\vec{θ}}+(0)ω_0^2\vec{θ}=0
eq(6) \ddot{\vec{θ}}+(3)ω_0^2\vec{θ}=0
When I plug in eigenvectors 2 and 3 into eq(6) I get that ω^2 = 3ω_0^2.
Physically, I think this scenario would correspond to one particle being held still while the other two oscillate back and forth with the same angular speed but in the opposite angular direction. Its been forever since I have dealt with systems of DE's and I seem to remember needing to construct another vector when a matrix had a repeated eigenvalue (like this one does) in order to form a complete solution space for the system. Also the scenario I give satifies that total angular momentum is zero, but it doesn't satisfy the second condition that θ_1=θ_2=θ_3=0 which is what really kind of has me worried. Thank you for any help in advance!(:
Last edited:
