Temperature change (first law of thermodynamics)

In summary, the first law of thermodynamics states that as long as the current rate of solar heat flow is positive, the temperature will continue to increase. This can be seen in the idealized diurnal evolution of air temperature, where the temperature keeps rising until its maximum value, despite there being net heat loss. However, it is important to note that temperature change is Eulerian (a local change at a fixed position) while energy flux density is Lagrangian (it crosses the air on its path with varying values). Both of these are observed by a stationary observer on the surface of the earth.
  • #1
jones123
10
0
Hi,

From the first law of thermodynamics it follows:

Cp * (δT/δt) = (δQ/δt)
where Cp = specific heat capacity, T = temperature, Q = heat, t = time

From this formula, you would derive that temperature keeps on increasing as long as dQ/dt > 0. But if you, for example, look at the idealized diurnal evolution of air temperature, it can be seen that the temperature keeps on increasing until its maximum value, despite the fact that there is net heat loss (dQ/dt < 0 for example between noon and 4h where the area between both curves becomes smaller)...

It seems that on the figure here the temperature keeps on rising as long as the value of Q itself > 0 but that doesn't necessarily mean that dQ/dt has to be > 0, right? Is my reasoning wrong or how can this correctly be explained with formulas?

Thanks already!

DailyT.jpg
 
Science news on Phys.org
  • #2
The sun doesn't heat the air, it heats the ground.
 
  • #3
jones123 said:
Hi,

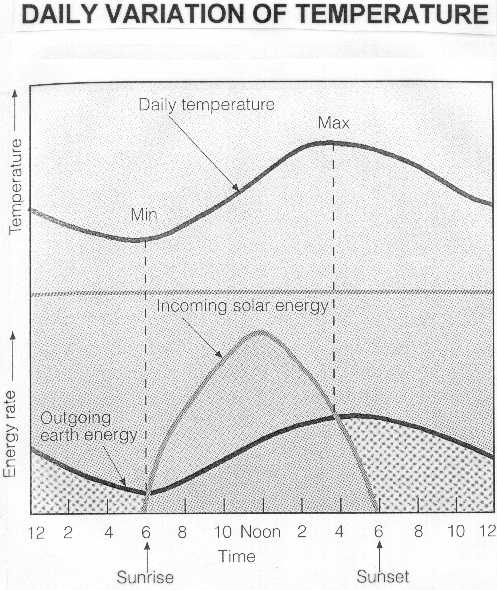
From the first law of thermodynamics it follows:

Cp * (δT/δt) = (δQ/δt)
where Cp = specific heat capacity, T = temperature, Q = heat, t = time

From this formula, you would derive that temperature keeps on increasing as long as dQ/dt > 0. But if you, for example, look at the idealized diurnal evolution of air temperature, it can be seen that the temperature keeps on increasing until its maximum value, despite the fact that there is net heat loss (dQ/dt < 0 for example between noon and 4h where the area between both curves becomes smaller)...

It seems that on the figure here the temperature keeps on rising as long as the value of Q itself > 0 but that doesn't necessarily mean that dQ/dt has to be > 0, right? Is my reasoning wrong or how can this correctly be explained with formulas?

Thanks already!

View attachment 205308
dQ/dt is the current rate of solar heat flow. So throughout the day, current rate of solar heat flow is positive. The graph says Energy Rate, not cumulative amount of energy.
 
  • Like
Likes russ_watters
  • #4
Chestermiller said:
dQ/dt is the current rate of solar heat flow. So throughout the day, current rate of solar heat flow is positive. The graph says Energy Rate, not cumulative amount of energy.
Oh I get it,

it's just that the temperature change is Eulerian (a local change at a fixed position) whereas the energy flux density is Lagrangian (it crosses the air on its path while having a negative or positive value).
 
  • #5
jones123 said:
Oh I get it,

it's just that the temperature change is Eulerian (a local change at a fixed position) whereas the energy flux density is Lagrangian (it crosses the air on its path while having a negative or positive value).
Both are as reckoned by a "stationary" observer on the surface of the earth.
 

1. What is the first law of thermodynamics?

The first law of thermodynamics states that energy cannot be created or destroyed, only transferred or converted from one form to another. This is also known as the law of conservation of energy.

2. How does the first law of thermodynamics relate to temperature change?

The first law of thermodynamics explains that when energy is transferred or converted, it can manifest as a change in temperature. For example, when heat is added to a system, the molecules within the system gain kinetic energy and their movement causes an increase in temperature.

3. Why is temperature change important in thermodynamics?

Temperature change is important in thermodynamics because it is a key factor in determining the direction of energy flow and the efficiency of energy conversions. It also plays a crucial role in many physical and chemical processes, such as phase changes and chemical reactions.

4. How does the first law of thermodynamics apply to the Earth's climate?

The first law of thermodynamics applies to the Earth's climate by explaining the energy balance between incoming solar radiation and outgoing thermal radiation. When the Earth's temperature changes, it is due to a change in the balance of energy, either from an increase in incoming radiation or a decrease in outgoing radiation.

5. How is temperature change measured in thermodynamics?

In thermodynamics, temperature change is typically measured using the Kelvin scale, which is based on absolute zero (-273.15 degrees Celsius). This scale allows for more precise measurements and eliminates negative values. Other commonly used scales for temperature include Celsius and Fahrenheit.

Similar threads

I Understand the Thermodynamic Identity: Is This Correct?
    • Thermodynamics
Replies
3
Views
994
I Application of the first law of thermodynamics
    • Thermodynamics
2
Replies
46
Views
2K
I Graphical difference between adiabatic and isothermal processes.
    • Thermodynamics
Replies
1
Views
624
I How Can Internal Energy of the Canonical Ensemble Change (Fluctuate)?
    • Thermodynamics
Replies
15
Views
1K
A Average temperature in a greenhouse
    • Thermodynamics
Replies
2
Views
1K
B Change in entropy of reversible isothermal process
    • Thermodynamics
Replies
11
Views
301
I Entropy change due to heat transfer between sources
    • Thermodynamics
Replies
15
Views
1K
Question about the first law of thermodynamics
    • Thermodynamics
Replies
3
Views
1K
The Second Law of Thermodynamics and the Stefan-Boltzmann Law
    • Thermodynamics
Replies
6
Views
1K
Definition of Heat and the First Law of Thermodynamics (discrepancy?)
    • Thermodynamics
Replies
5
Views
1K

Hot Threads

Recent Insights

Back
Top