- #1
perwiradua
- 7
- 0
Could you please check the statement of the theorem and the proof? If the proof is more or less correct, can it be improved?
Theorem
Let
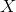 be a topological space and
be a topological space and
 be the discrete space.
be the discrete space.
The space
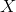 is connected if and only if for any continuous functions
is connected if and only if for any continuous functions
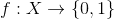 , the function
, the function
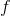 is not onto.
is not onto.
Proof
Only if:
Suppose the space
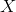 is connected and suppose there exists a continuous function
is connected and suppose there exists a continuous function
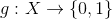 which is onto. Then
which is onto. Then
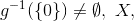 since
since
 is onto. Also since
is onto. Also since
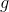 is continuous,
is continuous,
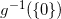 is open and a proper subset of
is open and a proper subset of
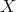 . Let
. Let
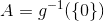 and
and
 . Both
. Both
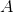 and
and
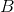 are
are
proper and nonempty and clopen, and
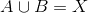 . But this contradicts the connectedness of
. But this contradicts the connectedness of
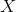 .
.
If:
Conversely suppose for all functions
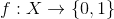 , the function
, the function
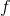 is not onto. Suppose
is not onto. Suppose
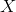 is disconnected, say
is disconnected, say
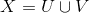 where
where
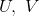 are clopen and
are clopen and
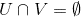 . But then the function
. But then the function
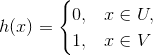
is continuous and onto.
Theorem
Let
The space
Proof
Only if:
Suppose the space
proper and nonempty and clopen, and
If:
Conversely suppose for all functions
is continuous and onto.