Transformation of the contravariant and covariant components of a tensor

[shinobi20]

TL;DR Summary

I wanted to clarify some things on the transformation of contravariant and covariant components of a tensor given the fact that I have viewed many articles and post here.

I have read many GR books and many posts regarding the title of this post, but despite that, I still feel the need to clarify some things.

Based on my understanding, the contravariant component of a vector transforms as,

$A'^\mu = [L]^\mu~ _\nu A^\nu$

the covariant component of a vector transforms as,

$A'_\mu = [L^{-1}]^\nu~ _\mu A_\nu = [L]_\mu~^\nu A_\nu$

Note that $~[L^{-1}]^\nu~_\mu = g^{\nu \alpha} g_{\mu \beta} [L]^\beta~_\alpha = [L]_\mu~^\nu$.

So based on this, it is my impression that all LT and inverse LT matrix must be written so that their indices are like this $^\mu~ _\nu$ and only when you want to rewrite the inverse LT as an LT then you realign $_\mu~ ^\nu$.

This is what I think until I read Cheng's GR book.

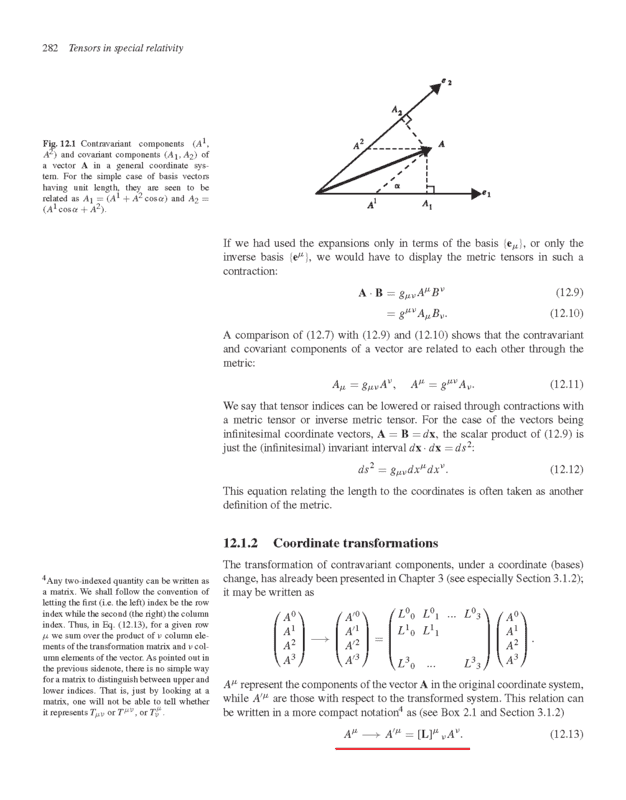
For the review question 3 & 4, why did he write the covariant transformation in that way $_\mu~ ^\nu$?

 

[Ibix]

The index placement looks odd to me in places throughout that. For example, the third rank tensor is shown as $T^\lambda_{\mu\nu}$. Perhaps it's a notation scheme I'm unfamiliar with, but it was my understanding that you have to keep track of which index was raised. That is, I understand $T^\lambda{}_{\mu\nu}$, $T_\mu{}^\lambda{}_\nu$ and $T_{\mu\nu}{}^\lambda$ as the same tensor with different indices raised, but it's not clear to me which index is supposed to be raised in $T^\lambda_{\mu\nu}$.

I don't have the book so I cannot investigate myself. Is it possible there are just some typographical issues here?

 

[shinobi20]

The index placement looks odd to me in places throughout that. For example, the third rank tensor is shown as $T^\lambda_{\mu\nu}$. Perhaps it's a notation scheme I'm unfamiliar with, but it was my understanding that you have to keep track of which index was raised. That is, I understand $T^\lambda{}_{\mu\nu}$, $T_\mu{}^\lambda{}_\nu$ and $T_{\mu\nu}{}^\lambda$ as the same tensor with different indices raised, but it's not clear to me which index is supposed to be raised in $T^\lambda_{\mu\nu}$.

I don't have the book so I cannot investigate myself. Is it possible there are just some typographical issues here?

I totally agree with what all you said. Looking at the errata sheet for the book and scanning the whole book, that seems not to be a typo but a notation used throughout, which I think is confusing hence my post.

 

[vanhees71]

The index placement is indeed very important and in #1 it's correct. You must make sure to have the indices located right in both horizontal and vertical placement. The example notation in #2, $T_{\mu \nu}^{\lambda}$ is thus useless and meaningless. I guess it's the inability of authors to use the tensor package properly in LaTeX, and nowadays publishers don't care about typography but simply take what the authors provide (sometimes even distorting it in the process, as anybody knows who publishes in standard physics journals ;-)). For this reason I'd not recommend the use of this book from just looking at the two example pages given in #1. Maybe it's a good exercise to repair all the sloppy notation, but to start learning a subject with such a sloppy source is not a good idea, if you ask me.

 

[shinobi20]

The index placement is indeed very important and in #1 it's correct. You must make sure to have the indices located right in both horizontal and vertical placement. The example notation in #2, $T_{\mu \nu}^{\lambda}$ is thus useless and meaningless. I guess it's the inability of authors to use the tensor package properly in LaTeX, and nowadays publishers don't care about typography but simply take what the authors provide (sometimes even distorting it in the process, as anybody knows who publishes in standard physics journals ;-)). For this reason I'd not recommend the use of this book from just looking at the two example pages given in #1. Maybe it's a good exercise to repair all the sloppy notation, but to start learning a subject with such a sloppy source is not a good idea, if you ask me.

Oh, so what GR book do you think I need to read to understand it using correct index placements?
Do you also know any tensor calculus books that teach using the correct index placement?

 

[vanhees71]

My favorite introductory GR textbook is Landau and Lifshitz vol. 2 (which also has a very good intro into classical electrodynamics).

 

[Ibix]

tensor package

Can you use that at PF? I've always used T^a{}_b to get $T^a{}_b$.

Oh, so what GR book do you think I need to read to understand it using correct index placements?

I used Sean Carroll's online lecture notes and Ben Crowell's downloadable GR book (because I wanted something I could read on the train) supplemented by Schutz' First Course in General Relativity.

I must get hold of Landau and Lifshitz at some point.

 

[shinobi20]

My favorite introductory GR textbook is Landau and Lifshitz vol. 2 (which also has a very good intro into classical electrodynamics).

Can you use that at PF? I've always used T^a{}_b to get $T^a{}_b$.

I used Sean Carroll's online lecture notes and Ben Crowell's downloadable GR book (because I wanted something I could read on the train) supplemented by Schutz' First Course in General Relativity.

I must get hold of Landau and Lifshitz at some point.

All these authors, I believe explain GR very well, however, I have not been able to find a book that explicitly explains the subtleties of index placements, index raising/lowering, etc. Thus, I am very curious if anyone can point me in the proper direction on this. I can handle the GR part using many of the famous books, it is the tensor algebra/analysis that really irritates me since many authors just don't bother with the subtleties.

 

[vanhees71]

I'm not aware how to insert the tensor package on PF. I also use brackets. For most purposes that's good enough, but sometimes you have quite troublesome typographical issues, where the tensor package gives better results though it's also not perfect, but one can live with it, though the alignment is sometimes not too good (see e.g. Eq. (A.5.1) in my SR manuscript [1]). If you have an idea, how to improve the alignment in such cases beyond what I achieved with \tensor*, it's very welcome!

[1] https://itp.uni-frankfurt.de/~hees/pf-faq/srt.pdf

 

[shinobi20]

I'm not aware how to insert the tensor package on PF. I also use brackets. For most purposes that's good enough, but sometimes you have quite troublesome typographical issues, where the tensor package gives better results though it's also not perfect, but one can live with it, though the alignment is sometimes not too good (see e.g. Eq. (A.5.1) in my SR manuscript [1]). If you have an idea, how to improve the alignment in such cases beyond what I achieved with \tensor*, it's very welcome!

[1] https://itp.uni-frankfurt.de/~hees/pf-faq/srt.pdf

Can you clear it up to me what I should interpret as row or column in the indices? For example

$[L^{-1}]^\nu~_\mu = g^{\nu \alpha} g_{\mu \beta} [L]^\beta~_\alpha = [L]_\mu~^\nu$

In $~[L^{-1}]^\nu~_\mu~$ ,$\nu$ is the row and $\mu$ is the column.

How about in $~[L]_\mu~^\nu$? Still $\nu$ is the row and $\mu$ is the column right?

Such that,

$A'_\mu B'^\mu = [L^{-1}]^\nu~_\mu [L]^\mu~_\lambda A_\nu B^\lambda = \delta^\nu~_\lambda A_\nu B^\lambda = A_\nu B^\nu$

where the column $\mu$ of $[L^{-1}]^\nu~_\mu$ is summed with the row $\mu$ of $[L]^\mu~_\lambda$.

But what if I use $[L^{-1}]^\nu~_\mu = [L]_\mu~^\nu$, so that

$A'_\mu B'^\mu = [L]_\mu~^\nu [L]^\mu~_\lambda A_\nu B^\lambda = \delta^\nu_\lambda A_\nu B^\lambda = A_\nu B^\nu$

I am not sure if I placed the indices in the $\delta$ correctly since both $\nu$ and $\lambda$ are on the right side of the placement. Please clarify my questionS

 

[PeterDonis]

I have not been able to find a book that explicitly explains the subtleties of index placements, index raising/lowering, etc.

Misner, Thorne, and Wheeler go into it in some detail.

Can you clear it up to me what I should interpret as row or column in the indices?

You should first understand that transformation matrices are not tensors, and their indices don't mean the same thing as tensor indices.

 

[shinobi20]

You should first understand that transformation matrices are not tensors, and their indices don't mean the same thing as tensor indices.

Yes, like the LT matrix is not a tensor, but can you expound on what you mean?

 

[vanhees71]

In the matrix notation of "objects" with two indices the first index labels the rows and the second one the columns. You cannot so easily distinguish upper and lower indices. As your posting shows, there's no need for the matrix calculus. Everything works out with much more safety in the Ricci calculus with indices, where you have clear transformation properties due to the notation with upper and lower indices for contra- and covariantly transforming components.

Your calculations look all good. For the $\delta$ (Kronecker symbol) the "horizontal order" is irrelevant, because it's symmetric, i.e.,
$$\delta_{\mu}^{\nu}={\delta_{\mu}}^{\nu} ={\delta^{\nu}}_{\mu}=\begin{cases} 1 &\text{for} \quad \mu=\nu, \\ 0 & \text{for} \quad \mu \neq \nu. \end{cases}.$$

 

[shinobi20]

In the matrix notation of "objects" with two indices the first index labels the rows and the second one the columns. You cannot so easily distinguish upper and lower indices. As your posting shows, there's no need for the matrix calculus. Everything works out with much more safety in the Ricci calculus with indices, where you have clear transformation properties due to the notation with upper and lower indices for contra- and covariantly transforming components.

Your calculations look all good. For the $\delta$ (Kronecker symbol) the "horizontal order" is irrelevant, because it's symmetric, i.e.,
$$\delta_{\mu}^{\nu}={\delta_{\mu}}^{\nu} ={\delta^{\nu}}_{\mu}=\begin{cases} 1 &\text{for} \quad \mu=\nu, \\ 0 & \text{for} \quad \mu \neq \nu. \end{cases}.$$

Ok, I think I get the tensor manipulation with indices stuff (contravariant/covariant transformation, index raising/lowering, etc), but at some point I have to interpret it in terms of matrix if I need to calculate matrices right? So when should I distinguish between the two and what are the rules (do's and don'ts)? It is easy to say that yes you don't need matrix calculus if you are working with Ricci calculus etc, but as an amateur who only relies on textbooks, I can see those stuff used interchangeably and there are no clear cut explanation as what I should be careful about, so is it possible to discuss that here clearly or could you point me to the proper resources (aside from MTW, if ever it is explained there) where I can read this on my own?

Also what do you mean when you said, "You cannot so easily distinguish upper and lower indices"

If it is as trivial as knowing which index is up and which index is down, then by looking, you will already know, so I think this is not what you mean.
So what you mean is... It is not easy to distinguish which is the row or column between the upper and lower indices? Correct me if I'm wrong, and if I'm correct, then how do you distinguish which is row or column between the upper and lower indices (which is one of my original question)?

 

[PeterDonis]

at some point I have to interpret it in terms of matrix

No, you don't. You can do all the tensor calculations without ever representing any tensors as matrices. Which is a good thing, since for a tensor with more than two indexes, you can't represent it as a matrix anyway. And even for two-index tensors, the matrix representation obfuscates some things that really shouldn't be obfuscated.

 

[shinobi20]

No, you don't. You can do all the tensor calculations without ever representing any tensors as matrices. Which is a good thing, since for a tensor with more than two indexes, you can't represent it as a matrix anyway. And even for two-index tensors, the matrix representation obfuscates some things that really shouldn't be obfuscated.

If I need to use the Lorentz transformation matrix explicitly, then I would need to. So if ever that is the case, then the question is as in post #14.

 

[vanhees71]

$\newcommand{\uvec}[1]{\underline{#1}}$

Ok, I think I get the tensor manipulation with indices stuff (contravariant/covariant transformation, index raising/lowering, etc), but at some point I have to interpret it in terms of matrix if I need to calculate matrices right? So when should I distinguish between the two and what are the rules (do's and don'ts)? It is easy to say that yes you don't need matrix calculus if you are working with Ricci calculus etc, but as an amateur who only relies on textbooks, I can see those stuff used interchangeably and there are no clear cut explanation as what I should be careful about, so is it possible to discuss that here clearly or could you point me to the proper resources (aside from MTW, if ever it is explained there) where I can read this on my own?

Also what do you mean when you said, "You cannot so easily distinguish upper and lower indices"

If it is as trivial as knowing which index is up and which index is down, then by looking, you will already know, so I think this is not what you mean.
So what you mean is... It is not easy to distinguish which is the row or column between the upper and lower indices? Correct me if I'm wrong, and if I'm correct, then how do you distinguish which is row or column between the upper and lower indices (which is one of my original question)?

If you want to use the matrix notation (which I'd not recommend in general, but it sometimes can shorten some calculations) then you have to stick to the rules, i.e., in a matrix the first index numbers the rows, the second the columns, no matter whether they are upper or lower indices. So you have to clearly specify which "index pattern" you associate with your matrix. E.g., for the Lorentz-transformation matrix usually one defines
$$\hat{\Lambda}=({\Lambda^{\mu}}_{\nu})$$
and for vectors you write a column
$$\underline{V}=(V^{\mu}).$$
Then the transformation rule under Lorentz transformation reads
$$\underline{V}'=\hat{\Lambda} \underline{V}.$$
Further for the metric components
$$\hat{\eta}=(\eta_{\mu \nu})=(\eta^{\mu \nu})=\mathrm{diag}(1,-1,-1,-1).$$
Then the Minkowski product reads
$$U \cdot V=\underline{U}^{\text{T}} \hat{\eta} \underline{V}.$$
Then from
$$\underline{U}^{\prime \text{T}} \hat{\eta} \underline{V}'=\underline{U}^{\text{T}} \hat{\eta} \underline{V}$$
you get (since it should hold for any $\underline{U}$ and $\underline{V}$) for the Lorentz-transformation matrices
$$\hat{\Lambda}^{\text{T}} \hat{\eta} \hat{\Lambda}=\hat{\eta}.$$
multiplying with $\hat{\eta}$ from the right and with $\hat{\Lambda}^{-1}$ from the left yields
$$\hat{\Lambda}^{-1} = \hat{\eta}\hat{\Lambda}^{\text{T}} \hat{\eta}.$$
For the transformation rule of the covariant components note that the covariant components given as a column are $\hat{\eta} \uvec{V}$. For the corresponding transformation matrix $\tilde{\Lambda}$ you want
$$\hat{\eta} \uvec{V}'=\tilde{\Lambda} \hat{\eta} \uvec{V}=\hat{\eta} \hat{\Lambda} \uvec{V} = (\hat{\eta} \hat{\Lambda} \hat{\eta}) \hat{\eta} \uvec{V}$$
and since this should hold for all $\uvec{V}$
$$\tilde{\Lambda} = \hat{\eta} \hat{\Lambda} \hat{\eta} = (\hat{\Lambda}^{-1})^{\text{T}},$$
i.e., the covariant components transform contravariantly to the contravariant components as expected.

 

[Ibix]

If I need to use the Lorentz transformation matrix explicitly, then I would need to. So if ever that is the case, then the question is as in post #14.

You never need to. I have to say, my feeling is that all of the index placement (except upper and lower) on coordinate transforms is an unnecessary flourish. There's only the forward and inverse transforms - but they have opposite effects when applied to co- and contra-variant tensor components. So people add this diagonal index placement to try to indicate that this forward transformation is actually an inverse transformation applied to a co-vector... or something. Honestly, as long as I establish clearly what the relationship between my coordinates is (i.e., write out my primed coordinates explicitly in terms of my unprimed ones at some point) I can do this:$$\begin{eqnarray*}
V'^\nu&=&L^\nu_\mu V^\mu\\
\omega'_\nu&=&L^\mu_\nu\omega_\mu\\
V^\nu&=&L^\nu_\mu V'^\mu\\
\omega_\nu&=&L^\mu_\nu\omega'_\mu\end{eqnarray*}$$I would not describe that as a good notation, using the same symbol for both forward and inverse transforms, but it's still completely unambiguous. I'm just saying do a Lorentz transform (or whatever transform I established previously). You can see from the primes whether I'm transforming from unprimed to primed coordinates or vice versa. And you can tell from that and the index placement what the components of $L$ must be. The rules are:

• Each component of $L$ is a partial derivative of one coordinate from one system with respect to one coordinate in the other system (i.e. they all look like either $\partial x^a/\partial x'^b$ or $\partial x'^a/\partial x^b$)
• If you're transforming an upper index, you need the matching index on the bottom of the derivative
• If you're transforming a lower index, you need the matching index on the top of the derivative
• Prime goes on the top or bottom coordinate, whichever has the index from the primed tensor

Applying those rules:$$\begin{eqnarray*}
V'^\nu&=&\frac{\partial x'^\nu}{\partial x^\mu} V^\mu\\
\omega'_\nu&=&\frac{\partial x^\mu}{\partial x'^\nu}\omega_\mu\\
V^\nu&=&\frac{\partial x^\nu}{\partial x'^\mu} V'^\mu\\
\omega_\nu&=&\frac{\partial x'^\mu}{\partial x^\nu}\omega'_\mu\end{eqnarray*}$$That works generally - not just for the Lorentz transforms. You are welcome to write out the sums explicitly and fill in the partial derivatives for the Lorentz transforms to see if you get the matrices you expect.

So my point is that upper/lower index placement is important, but order only matters for tensors. NW/SE index placement on coordinate transformations is unnecessary, and isn't used consistently between authors anyway (Carroll notes explicitly that Shutz uses a different convention from Carroll's). You can deduce what's actually meant purely from the upper/lower index placement and knowing what a coordinate transform actually does. The conventions are meant to help you, to remind you which is the forward and which is the inverse transform. But, frankly, since they're inconsistently used I think they're more confusing than helpful, and I just note that people have conventions and otherwise ignore them.

 

[vanhees71]

But again. I strongly recommend to be careful with the vertical AND HORIZONTAL placement of the indices. The first equation must read
$$V^{\prime \nu}={\Lambda^{\nu}}_{\mu} V^{\mu}.$$
The second one is
$$\omega_{\nu}'=g_{\nu \rho} \omega^{\prime \rho} = g_{\nu \rho} {\Lambda^{\rho}}_{\sigma} \omega^{\sigma} = g_{\nu \rho} {\Lambda^{\rho}}_{\sigma} \eta^{\sigma \alpha} \omega_{\alpha} ={\Lambda_{\nu}}^{\alpha} \omega_{\alpha}={\Lambda_{\nu}}^{\mu} \omega_{\mu}.$$
The third reads
$$V^{\nu} = {(L^{-1})^{\nu}}_{\mu} V^{\prime \mu} = {L_{\mu}}^{\nu} V^{\prime \mu},$$
because
$$\eta_{\mu \nu} {\Lambda^{\mu}}_{\rho} {\Lambda^{\nu}}_{\sigma}=\eta_{\rho \sigma}$$
and thus by mutliplying with $\eta^{\rho \alpha}$ on both sides
$${\Lambda_{\nu}}^{\alpha} {\Lambda^{\nu}}_{\sigma}=\delta_{\sigma}^{\alpha}$$
and thus
$${(\Lambda^{-1})^{\nu}}_{\mu} = {\Lambda_{\mu}}^{\nu}.$$
As you see, the horizontal placement of the indices is as crucial in the Ricci calculus as is the vertical!

 

[shinobi20]

$\newcommand{\uvec}[1]{\underline{#1}}$

$$\tilde{\Lambda} = \hat{\eta} \hat{\Lambda} \hat{\eta} = (\hat{\Lambda}^{-1})^{\text{T}},$$
i.e., the covariant components transform contravariantly to the contravariant components as expected.

So a covariant vector transforms in such a way that the transformation matrix is the transpose of the inverse of the LT (transformation matrix for a contravariant vector). By explicitly showing this in your derivation, there are a lot of things that I learned because textbooks don't explicitly explain this. (Please read my discussion/statement below to confirm if my understanding of what you derived is correct)

What do you mean by "the covariant components transform contravariantly to the contravariant components as expected"?

You never need to. I have to say, my feeling is that all of the index placement (except upper and lower) on coordinate transforms is an unnecessary flourish. There's only the forward and inverse transforms - but they have opposite effects when applied to co- and contra-variant tensor components. So people add this diagonal index placement to try to indicate that this forward transformation is actually an inverse transformation applied to a co-vector... or something. Honestly, as long as I establish clearly what the relationship between my coordinates is (i.e., write out my primed coordinates explicitly in terms of my unprimed ones at some point) I can do this:$$\begin{eqnarray*}
V'^\nu&=&L^\nu_\mu V^\mu\\
\omega'_\nu&=&L^\mu_\nu\omega_\mu\\
V^\nu&=&L^\nu_\mu V'^\mu\\
\omega_\nu&=&L^\mu_\nu\omega'_\mu\end{eqnarray*}$$I would not describe that as a good notation, using the same symbol for both forward and inverse transforms, but it's still completely unambiguous. I'm just saying do a Lorentz transform (or whatever transform I established previously). You can see from the primes whether I'm transforming from unprimed to primed coordinates or vice versa. And you can tell from that and the index placement what the components of $L$ must be. The rules are:

• Each component of $L$ is a partial derivative of one coordinate from one system with respect to one coordinate in the other system (i.e. they all look like either $\partial x^a/\partial x'^b$ or $\partial x'^a/\partial x^b$)
• If you're transforming an upper index, you need the matching index on the bottom of the derivative
• If you're transforming a lower index, you need the matching index on the top of the derivative
• Prime goes on the top or bottom coordinate, whichever has the index from the primed tensor

Applying those rules:$$\begin{eqnarray*}
V'^\nu&=&\frac{\partial x'^\nu}{\partial x^\mu} V^\mu\\
\omega'_\nu&=&\frac{\partial x^\mu}{\partial x'^\nu}\omega_\mu\\
V^\nu&=&\frac{\partial x^\nu}{\partial x'^\mu} V'^\mu\\
\omega_\nu&=&\frac{\partial x'^\mu}{\partial x^\nu}\omega'_\mu\end{eqnarray*}$$That works generally - not just for the Lorentz transforms. You are welcome to write out the sums explicitly and fill in the partial derivatives for the Lorentz transforms to see if you get the matrices you expect.

So my point is that upper/lower index placement is important, but order only matters for tensors. NW/SE index placement on coordinate transformations is unnecessary, and isn't used consistently between authors anyway (Carroll notes explicitly that Shutz uses a different convention from Carroll's). You can deduce what's actually meant purely from the upper/lower index placement and knowing what a coordinate transform actually does. The conventions are meant to help you, to remind you which is the forward and which is the inverse transform. But, frankly, since they're inconsistently used I think they're more confusing than helpful, and I just note that people have conventions and otherwise ignore them.

I think if I explicitly use partial derivatives as the transformation between contravariant and covariant vectors then you have a point in that there are only two kinds of transformations, $\frac{\partial x'^\nu}{\partial x^\mu}$ (forward/contravariant transformation) and $\frac{\partial x^\mu}{\partial x'^\nu}$ (inverse/covariant transformation).

According to what you wrote,

$V'^\nu = \frac{\partial x'^\nu}{\partial x^\mu} V^\mu$
$\omega'_\nu = \frac{\partial x^\mu}{\partial x'^\nu}\omega_\mu$

The contravariant transformation shows that the prime is in the numerator and the unprimed is in the denominator. For a covariant transformation, we switch the prime and unprimed, BUT then due to the nature of the contravariant and covariant vectors, the indices also switches, i.e., that is for contravariant $\nu$ is in the numerator and $\mu$ is in the denominator, but for covariant $\mu$ is in the numerator and $\nu$ is in the denominator, I think this is the reason the horizontal index placement is important if NOT writing in partial derivative form! @vanhees71 am I correct here?

But again. I strongly recommend to be careful with the vertical AND HORIZONTAL placement of the indices. The first equation must read
$$V^{\prime \nu}={\Lambda^{\nu}}_{\mu} V^{\mu}.$$
The second one is
$$\omega_{\nu}'=g_{\nu \rho} \omega^{\prime \rho} = g_{\nu \rho} {\Lambda^{\rho}}_{\sigma} \omega^{\sigma} = g_{\nu \rho} {\Lambda^{\rho}}_{\sigma} \eta^{\sigma \alpha} \omega_{\alpha} ={\Lambda_{\nu}}^{\alpha} \omega_{\alpha}={\Lambda_{\nu}}^{\mu} \omega_{\mu}.$$
The third reads
$$V^{\nu} = {(L^{-1})^{\nu}}_{\mu} V^{\prime \mu} = {L_{\mu}}^{\nu} V^{\prime \mu},$$
because
$$\eta_{\mu \nu} {\Lambda^{\mu}}_{\rho} {\Lambda^{\nu}}_{\sigma}=\eta_{\rho \sigma}$$
and thus by mutliplying with $\eta^{\rho \alpha}$ on both sides
$${\Lambda_{\nu}}^{\alpha} {\Lambda^{\nu}}_{\sigma}=\delta_{\sigma}^{\alpha}$$
and thus
$${(\Lambda^{-1})^{\nu}}_{\mu} = {\Lambda_{\mu}}^{\nu}.$$
As you see, the horizontal placement of the indices is as crucial in the Ricci calculus as is the vertical!

I will summarize.

For contravariant,

$V' = \Lambda V \quad \rightarrow \quad (\Lambda^{-1})^T V' = (\Lambda^{-1})^T \Lambda V = V$

where $\tilde{\Lambda} = (\Lambda^{-1})^T$ is the covariant transformation matrix as @vanhees71 stated in #17. I just want to clarify, so it is $(\Lambda^{-1})^T \Lambda = \Lambda (\Lambda^{-1})^T = I~$ not $~(\Lambda^{-1}) \Lambda = \Lambda (\Lambda^{-1})= I~$? Or is it just a notation issue where in matrix notation $(\Lambda^{-1})$ already has an inherent transpose in the operation (which is the case when taking inverses), so the transpose $T$ that you are pertaining in $(\Lambda^{-1})^T$ is the inherent transpose or is a transpose that must be done after taking the inverse?

$V' ^\nu = \Lambda^\nu~_\mu V^\mu$

$(\Lambda^{-1})^\alpha~_\nu \Lambda^\nu~_\mu V^\mu = (\Lambda^{-1})^\alpha~_\nu V'^\nu$

$V^\alpha = (\Lambda^{-1})^\alpha~_\nu V'^\nu$

For $\Lambda^\nu~_\mu$, $\nu$ is the row, but for $(\Lambda^{-1})^\alpha~_\nu$, $\nu$ is the column, this is exactly the effect of the transpose $(\Lambda^{-1})^T$.

For covariant,

$\omega' = (\Lambda^{-1})^T \omega \quad \rightarrow \quad \Lambda \omega' = \Lambda (\Lambda^{-1})^T \omega = \omega$

$\omega'_\nu = (\Lambda^{-1})^\mu~_\nu \omega_\mu$

$\Lambda^\nu~_\alpha (\Lambda^{-1})^\mu~_\nu \omega_\mu = \Lambda^\nu~_\alpha \omega'_\nu$

$(\Lambda^{-1})^\mu~_\nu \Lambda^\nu~_\alpha \omega_\mu = \Lambda^\nu~_\alpha \omega'_\nu$

$\omega_\alpha = \Lambda^\nu~_\alpha \omega'_\nu$

Notice that since $(\Lambda^{-1})^\mu~_\nu$, so the correct index placement is that for the contravariant transformation, we have $\Lambda^\nu~_\alpha$, we switch (transpose) the indices and change $\mu$ to $\alpha$.

So $\omega_\alpha$ transforms in the same way as $V' ^\nu$ and $V^\alpha$ transforms in the same way as $\omega'_\nu$.

 

[vanhees71]

This posting again shows that the matrix notation is much more complicated than the Ricci calculus.

First of all tensors (including scalars and vectors as special cases) are basis-independent objects and thus do not transform under basis changes. It's the components of tensors that change under basis transformations.

For a Lorentz-transformation matrix $\hat{\Lambda}=({\hat{\Lambda}^{\mu}}_{\nu})$, using the pseudometric components wrt. Minkowski bases, $\hat{\eta}=(\eta_{\mu \nu})=(\eta^{\mu \nu})=\mathrm{diag}(1,-1,-1,-1)$, as derived in my previous posting you have
$$\hat{\Lambda}^{-1}=\hat{\eta} \hat{\Lambda}^{\text{T}} \hat{\eta}$$
and, of course,
$$\hat{\Lambda}^{-1} \hat{\Lambda}=\hat{1} = \hat{\eta} \hat{\Lambda}^{\text{T}} \hat{\eta} \hat{\Lambda} \; \Rightarrow \; \hat{\Lambda}^{\text{T}} \hat{\eta} \hat{\Lambda}=\hat{\eta}.$$
Translated into the Ricci calculus these formulae read
$${(\hat{\Lambda}^{-1})^{\mu}}_{\nu} = \hat{\eta}^{\mu \alpha} {\hat{\Lambda}^{\beta}}_{\alpha} \eta_{\beta \nu} = {\Lambda_{\nu}}^{\mu}$$
and
$${\hat{\Lambda}^{\alpha}}_{\mu} \hat{\eta}_{\alpha \beta} {\hat{\Lambda}^{\beta}}_{\nu}=\eta_{\mu \nu}.$$

 

[shinobi20]

This posting again shows that the matrix notation is much more complicated than the Ricci calculus.

First of all tensors (including scalars and vectors as special cases) are basis-independent objects and thus do not transform under basis changes. It's the components of tensors that change under basis transformations.

For a Lorentz-transformation matrix $\hat{\Lambda}=({\hat{\Lambda}^{\mu}}_{\nu})$, using the pseudometric components wrt. Minkowski bases, $\hat{\eta}=(\eta_{\mu \nu})=(\eta^{\mu \nu})=\mathrm{diag}(1,-1,-1,-1)$, as derived in my previous posting you have
$$\hat{\Lambda}^{-1}=\hat{\eta} \hat{\Lambda}^{\text{T}} \hat{\eta}$$
and, of course,
$$\hat{\Lambda}^{-1} \hat{\Lambda}=\hat{1} = \hat{\eta} \hat{\Lambda}^{\text{T}} \hat{\eta} \hat{\Lambda} \; \Rightarrow \; \hat{\Lambda}^{\text{T}} \hat{\eta} \hat{\Lambda}=\hat{\eta}.$$
Translated into the Ricci calculus these formulae read
$${(\hat{\Lambda}^{-1})^{\mu}}_{\nu} = \hat{\eta}^{\mu \alpha} {\hat{\Lambda}^{\beta}}_{\alpha} \eta_{\beta \nu} = {\Lambda_{\nu}}^{\mu}$$
and
$${\hat{\Lambda}^{\alpha}}_{\mu} \hat{\eta}_{\alpha \beta} {\hat{\Lambda}^{\beta}}_{\nu}=\eta_{\mu \nu}.$$

Indeed it can be more complicated than Ricci calculus but that is why maybe we can discuss it so that I can at least understand that complication.

What can you say on post #20?

Also, how do you reconcile

in your post #17 you said that for covariant transformation $\tilde{\Lambda} = (\hat{\Lambda}^{-1})^T$, so that for two vectors $\vec{V}$ and $\vec{\omega}$,

$\vec{V'} \cdot \vec{\omega'} = \hat{\Lambda} \vec{V} \cdot \tilde{\Lambda} \vec{\omega} = \hat{\Lambda} \vec{V} \cdot (\hat{\Lambda}^{-1})^T \vec{\omega} = \hat{\Lambda} (\hat{\Lambda}^{-1})^T \vec{V} \cdot \vec{\omega} = \vec{V} \cdot \vec{\omega}$

where $\vec{V}$ is expanded in contravariant and $\vec{\omega}$ is expanded in covariant, so that $\hat{\Lambda} (\hat{\Lambda}^{-1})^T = \hat{1}$, but then you said $\hat{\Lambda} \hat{\Lambda}^{-1} = \hat{1}$. I guess my manipulation here is wrong but my point is made clear in tensor notation,

$V'^\mu = \hat{\Lambda}^\mu~_\nu V^\nu$

$\omega'_\mu = (\hat{\Lambda}^{-1})^\alpha~_\mu \omega_\alpha$

where $(\hat{\Lambda}^{-1})^\alpha~_\mu$ is obviously equal to $\tilde{\Lambda} = (\hat{\Lambda}^{-1})^T$ in matrix notation, they are both inverses and you can see that the index placement is transposed compared to $\hat{\Lambda}^\mu~_\nu$.

You can see that $(\hat{\Lambda}^{-1})^\alpha~_\mu \hat{\Lambda}^\mu~_\nu = \delta^\alpha_\nu$, so in matrix notation $(\hat{\Lambda}^{-1})^T \hat{\Lambda} = \tilde{\Lambda} \hat{\Lambda} = \hat{1}$.

 

[vanhees71]

Again, you must make clear for yourself first that vectors are invariant objects and only components depend on the basis chosen. It is clear that $\hat{\Lambda}^{-1} \hat{\Lambda}=\hat{\Lambda} \hat{\Lambda}^{-1}=\hat{1}$. There must be no transpose symbols in that formula, and in my previous posting I've shown to you again, how you translate from the Ricci to the matrix calculus and vice versa.

Just look at your last line: Your Ricci calculus is correct, but why did you put a transpose symbol in your matrix notation? There's none as the Ricci calculus clearly shows.

The matrix notation for $\tilde{\lambda}$ is correct, i.e., in the Ricci calculus
$${\tilde{\Lambda}_{\mu}}^{\nu}={(\hat{\Lambda}^{-1})^{\nu}}_{\mu}.$$
Note that the vertical placement of the indices is lost in the matrix calculus, and you have to be careful when you switch from the one to the other calculus.

The horizontal placement of the indices in symbols with two indices is always such that the horizontally 1st index labels the rows and the 2nd the columns (no matter whether they are upper or lower indices). Transposition means to exchange the horizontal order of the indices (not their vertical placement).

 

[vanhees71]

Indeed it can be more complicated than Ricci calculus but that is why maybe we can discuss it so that I can at least understand that complication.

What can you say on post #20?

Also, how do you reconcile

in your post #17 you said that for covariant transformation $\tilde{\Lambda} = (\hat{\Lambda}^{-1})^T$, so that for two vectors $\vec{V}$ and $\vec{\omega}$,

$\vec{V'} \cdot \vec{\omega'} = \hat{\Lambda} \vec{V} \cdot \tilde{\Lambda} \vec{\omega} = \hat{\Lambda} \vec{V} \cdot (\hat{\Lambda}^{-1})^T \vec{\omega} = \hat{\Lambda} (\hat{\Lambda}^{-1})^T \vec{V} \cdot \vec{\omega} = \vec{V} \cdot \vec{\omega}$

where $\vec{V}$ is expanded in contravariant and $\vec{\omega}$ is expanded in covariant, so that $\hat{\Lambda} (\hat{\Lambda}^{-1})^T = \hat{1}$, but then you said $\hat{\Lambda} \hat{\Lambda}^{-1} = \hat{1}$. I guess my manipulation here is wrong but my point is made clear in tensor notation,

$V'^\mu = \hat{\Lambda}^\mu~_\nu V^\nu$

$\omega'_\mu = (\hat{\Lambda}^{-1})^\alpha~_\mu \omega_\alpha$

where $(\hat{\Lambda}^{-1})^\alpha~_\mu$ is obviously equal to $\tilde{\Lambda} = (\hat{\Lambda}^{-1})^T$ in matrix notation, they are both inverses and you can see that the index placement is transposed compared to $\hat{\Lambda}^\mu~_\nu$.

You can see that $(\hat{\Lambda}^{-1})^\alpha~_\mu \hat{\Lambda}^\mu~_\nu = \delta^\alpha_\nu$, so in matrix notation $(\hat{\Lambda}^{-1})^T \hat{\Lambda} = \tilde{\Lambda} \hat{\Lambda} = \hat{1}$.

No! That's my whole point the whole time! You must obey the rules of matrix multiplication a matrix product (let's write first matrices with lower indices only, which is used to describe the Ricci calculus in Cartesian coordinates of a Euclidean vector space to avoid the complication that you have to be careful with the vertical placement too). By definition a matrix product $\hat{A} \hat{B}$ in the matrix notation translates to $$(\hat{A} \hat{B})_{ab}=A_{ac} B_{cb}.$$
This is DIFFERENT (!) from $\hat{A}^{\text{T}} \hat{B}$ which translates in the Ricci calculus to
$$(\hat{A}^{\text{T}} \hat{B})_{ab} = A_{ca} B_{cb}.$$
Except for the special case of a symmetric matrix, $\hat{A}^{-1} \neq \hat{A}^{-1 \text{T}}$!