Wave Equation: d'Alembert solution -- semi-infinite string with a fixed end

[Master1022]

Homework Statement

At $t = 0$ the string is released. Let $y(t,x) = f(x - ct) + g(x - ct)$. Obtain $f(u)$ and $g(v)$ for u > 0 and v > 0 using the initial condition.

Relevant Equations

d'Alembert solution to the wave equation

Hi,

I was trying to get some practice with the wave equation and am struggling to solve the problem below. I am unsure of how to proceed in this situation.

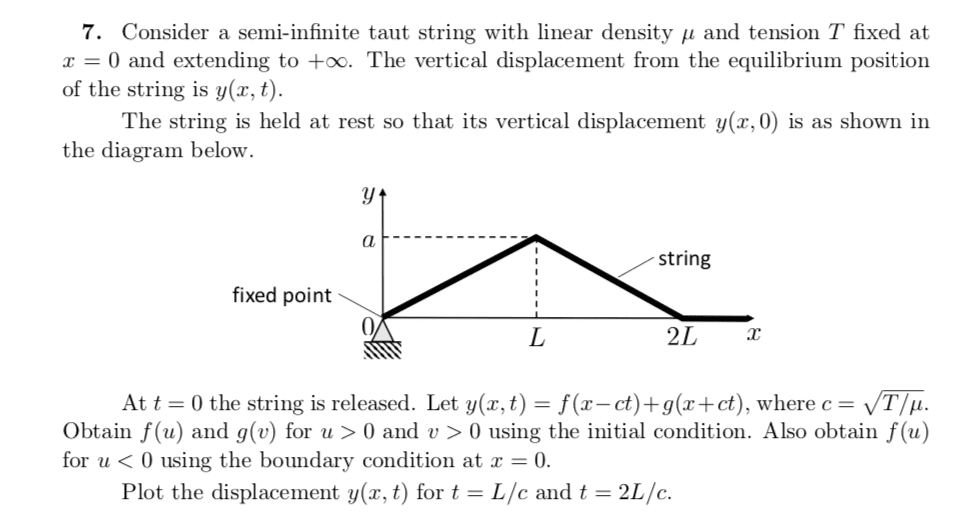
My attempt:
So we are told that the string is held at rest, so we only need to think about the displacement conditions for the wave equation solution. If we are using the given expression, then f will be the 'forward' (+ ve $x$) traveling wave and g will be the 'backward' (-ve $x$) traveling wave.

I would turn the given function $$H(x) = \begin{cases} -h(-x), & -2L \leq x \lt 0 \\ h(x), & 0 \leq x \lt 2L \\ (periodic), & otherwise \end{cases}$$
(where $h(x)$ is the triangular function shown - I could have explicitly written out the exact function, but just after the method for the moment)
(EDIT: was I correct to make it periodic?)
Then, we can use the solution to write: $y(t,x) = \frac{1}{2} \left( H(x + ct) + H(x - ct) \right)$ (replacing f and g with our defined function). Is that correct up to that point?

Some specific questions I have are:
- how do we deal with the fixed boundary condition? - I have just tried to create the odd periodic extension of the initial condition so that the zero displacement is satisfied at $x = 0$
- in general, do I start the two oppositely traveling waves from the same place on the string
- Perhaps related for the boundary condition, but how do I include the reflection that will take place? - I presume that we just allow our two waves to pass over one another so that the -ve part of the forward wave superposes with the +ve part of the backwards wave. After that, our backwards wave solution will be 'beyond the boundary' so we won't need to consider it any more?

Thanks in advance.

 

[LCKurtz]

You want to consider the odd extension of the function. Not the odd periodic extension. So your initial problem looks like an infinite string with initial displacement the odd reflection of the triangle and zero outside of $[-2L,2L]$ and released from rest. Solve that infinite string problem and just look at the part of the solution where $x>0$. By the way, +ve and -ve are not words.

 

[Master1022]

Thank you for your reply. I appreciate the help.

You want to consider the odd extension of the function. Not the odd periodic extension.

So I can just remove the periodicity to obtain the correct form of $H(x)$? Is this true for other d'Alembert solution questions as well (i.e. the fact that we only want the odd extension, but not the periodic form of it)? I ask as I seem to recall online sources frequently mentioned 'odd-periodic' extensions, but perhaps I misunderstood what they were saying?

So your initial problem looks like an infinite string with initial displacement the odd reflection of the triangle and zero outside of $[-2L,2L]$ and released from rest. Solve that infinite string problem and just look at the part of the solution where $x>0$.

Am I correct in thinking that there will be interference to consider in the beginning when the waves are passing over one another until they separate?

By the way, +ve and -ve are not words.

I am aware of that. I only included them to add some directionality to my explanation.

 

[LCKurtz]

Thank you for your reply. I appreciate the help.
So I can just remove the periodicity to obtain the correct form of $H(x)$? Is this true for other d'Alembert solution questions as well (i.e. the fact that we only want the odd extension, but not the periodic form of it)? I ask as I seem to recall online sources frequently mentioned 'odd-periodic' extensions, but perhaps I misunderstood what they were saying?

It depends on the boundary conditions. For a string with fixed ends, you might want a periodic extension. The idea is if you have an infinite string where a point never moves, that is indistinguishable from the string being tied down at that point.

Am I correct in thinking that there will be interference to consider in the beginning when the waves are passing over one another until they separate?

Yes. It is that interference that gives what looks like a reflection. I have a couple of .gifs below to help you see. I have a string that starts as one arch of a translated sine wave and its odd reflection. The first .gif below shows its motion:

You can see the interference where they meet. The .gif below just shows the plot for $x\ge 0$ and you can see how the interference really makes it look like a reflection:

 

[Master1022]

Many thanks for your quick reply.

It depends on the boundary conditions. For a string with fixed ends, you might want a periodic extension. The idea is if you have an infinite string where a point never moves, that is indistinguishable from the string being tied down at that point.

This makes more sense now, thanks for clearing that up. Also, thanks for including the animations.