- #1
Taylor_1989
- 402
- 14
Homework Statement
A string of length L is fixed at both ends ##u(0,L)=u(L,t)=0## The string is struck in the middle with a hammer of width a, leading to an intial condtion ##u(x,0)=0## and
$$U_t(x,0)=v_0 $$ for $$\frac{l}{2}-\frac{a}{2} \leq x \leq \frac{l}{2}+\frac{a}{2} $$
and
$$U_t(x,0)=0 $$ other wise
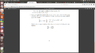
I have printed screen in the question Just encase it make no sense what I have wirtten
I am not going to put full working, I am having trouble with one particular part the intial condtions for my Fourier series.
Homework Equations
$$U_t(x,0)=\sum_{n=1}^\infty \left(-\frac{n\pi c}{L}\right)B_n sin(\frac{n\pi}{L}x)$$ [1]
$$\left(-\frac{n\pi c}{L}\right)B_n=\frac{2}{l}\int _0^lv_0sin\left(\frac{n\pi }{L}x\right)dx\:$$ [2]
$$B_n=-\frac{2v_0}{-n\pi c}\int _{\left(\frac{L}{2}-\frac{a}{2}\right)}^{\frac{L}{2}+\frac{a}{2}}sin\left(\frac{n\pi }{L}x\right)dx\:$$ [3]
The Attempt at a Solution
[3] soultuion
$$B_n=\frac{2Lv_0}{n^2\pi ^2c}\left(cos\left(\frac{n\pi }{2}\left(\frac{L+a}{L}\right)-cos\left(\frac{n\pi }{2}\left(\frac{L-a}{L}\right)\right)\right)\right)$$
I believe I have gone wrong with the limits and my problem lies in how the size of the hammer is effecting the wave in this case, could someone please advise, thanks in advace