Why Aren't All Space Rockets More Efficient?

[AkiAkane1973]

Hi PF,

I'm working on a paper about rockets but I've come across something which confuses me. For my paper I calculated the fuel/mass ratios for several rockets, and found that their ratios vary quite significantly (assuming of course that I've done the calculation correctly).

From my reading and understanding, the fuel/mass ratio is used as a measure of a rockets' efficiency. So why do they vary this much? Surely most rockets should have round about the same efficiency rates, and in my calculations some of the older rockets were even more efficient than more modern ones. In essence my question is, why do efficiency rates vary so significantly? Surely they are wasting fuel?

I currently have one line of thought, and I would love to get some outside help or opinions on this, particularly because I know next to nothing about the topic.

My first thought is to do with the missions on which the rockets were being used for. They most likely had different reasons for going to space, which leads to different equipment being needed, which leads to inconsistent mass readings across my examples. This is then why the efficiency is so different between the rockets. However my counter thought for this is based on the fact that I thought something like an efficiency rate would be constant for any individual rocket regardless of the ship's mass since increased ship mass would mean increased fuel mass. Essentially, I thought it was essential a straight line, and thus the gradient would be the same at any point.

Here is a "table" of my results.

Rocket Fuel Mass Dry Mass Ratio
TITAN 2G 146740 7080 20.7
TITAN 3 138300 8900 15.5
TITAN 4 208400 12800 16.2
Falcon 9 475000 22900 20.7
Soyuz-U 286300 24145 11.9
Angara 1.2PP 143300 13800 10.4
Saturn V 2725600 183600 14.8

And here is a copy of the mass ratio equation.

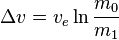
Here is the link to the mass ratio wikipedia page.
- http://en.wikipedia.org/wiki/Mass_ratio

 

[willem2]

The exhaust speed of the rocket is also very important for the efficiency. Liquid oxygen and hydrogen is the best option here, but liquid hydrogen is very bulky compared to the other options, and the large tank needed will likely decrease the mass ratio, and the extra air resistance is also a problem with the first stage. Many designs use something other than liquid hydrogen in the first stage, and liquid hydrogen for the next stages.

 

[BobG]

You need the orbit as well as the mass of the spacecraft . The higher the orbit, the more energy it takes to get your spacecraft there. The significance of the delta V is its relationship to energy. You can only add kinetic energy (unless you're on the USS Enterprise and have a transporter). The delta V is actually referring to the energy you're adding - not necessarily your final velocity, since some of the energy you add gets converted to potential energy.

In other words, it's not a given that all of those examples you gave obtain the same orbit.

Type of fuel is very important, too. Basically different fuel types give a different chemical reaction and a different exhaust velocity for the fuel. Thrusters work on conservation of momentum. That means the higher the exhaust velocity, the less mass that has to be thrown out the back to get a given delta V. Bipropellant thrusters usually have a higher exhaust velocity than solid fuel boosters. Different types of thrusters can packaged together on a single booster. In other words, a liquid fuel booster can have solid strap-on thrusters to increase the overall thrust.

The addition of an upper stage booster can change your values, as well. Your main booster could just get you to a low parking orbit, while the upper stage booster pushes the satellite up the rest of the way. In fact, for your very high orbits (semi-synchronous, geosynchronous), that's probably the case. Ironically, it's entirely possible that it would require more fuel from a main booster to get a satellite into a low altitude, since its final altitude may be higher than a parking orbit, but not high enough to add an upper stage booster.

How fast you want to get to your final orbit plays a part, too. Some of your most efficient thrusters have exhaust velocities in excess of 30,000 meters/second, but can only throw out such minute amounts of fuel that they'd be inappropriate to get your spacecraft out of the atmosphere. Your "thrust" would be measured in milliNewtons. (XIPS thrusters being the prime example).

In other words, you need more information than just booster type and fuel mass to figure out any meaningful relationship.