- #1
athosanian
- 67
- 8
dear all, the virtual work pinciple can be used to derive the equilibrium equations for the mechanical systems. however, when I want to apply it to a continuous system, I found it can not give out the simple equilibrium equations. there should be something wrong with my thinking. I expect some expert could give me some advice. thanks very much.
the problem detail is shown in the pictures:
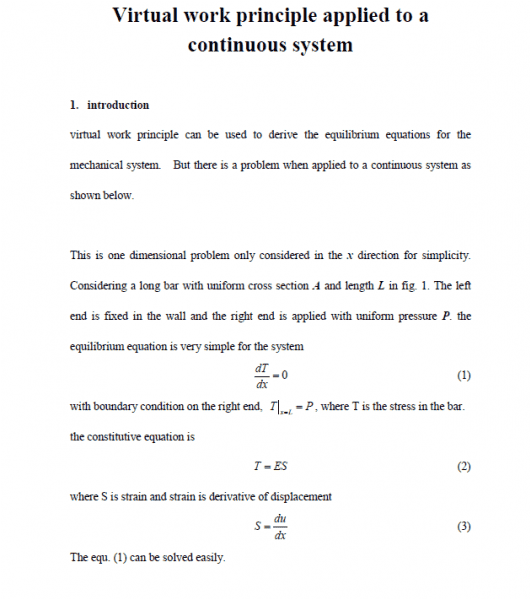
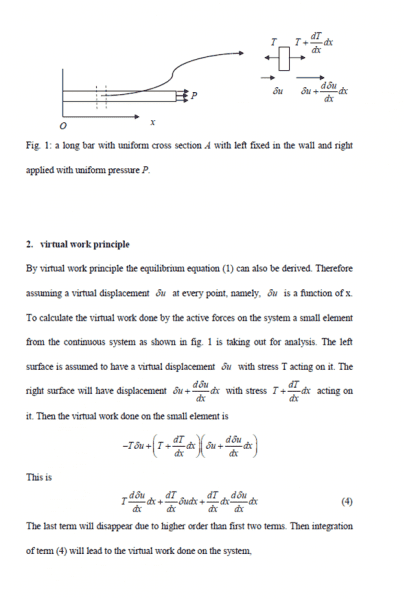

the problem detail is shown in the pictures:


