- #1
Diracobama2181
- 75
- 2
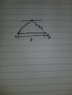
Suppose we have two different masses, m1m1 and m2m2 with each at the end of a massless rod of length ll, with each mass being attached to a separate spring of constant k such that both springs stem from the same point on the ceiling? What would be the Lagrangian of this system. I have tried several different approaches, but none seem to be quite right.
What I got thus far, r1=(x1,y1)(coordinate of the first mass) r2=(x2,y2) (coordinate of the second mass)
So T=(1/2)m1T=(1/2)m1((x˙1)^2+(y˙1)^2)+(1/2)m2((x˙2)^2+(y˙2)^2)
U=-mgy1-mgy2+(1/2)k((x1)^2+(y1)^2)+(1/2)k((x2)^2+(y2)^2).
From here, I get L=T-V. Is there any issues with my setup?
What I got thus far, r1=(x1,y1)(coordinate of the first mass) r2=(x2,y2) (coordinate of the second mass)
So T=(1/2)m1T=(1/2)m1((x˙1)^2+(y˙1)^2)+(1/2)m2((x˙2)^2+(y˙2)^2)
U=-mgy1-mgy2+(1/2)k((x1)^2+(y1)^2)+(1/2)k((x2)^2+(y2)^2).
From here, I get L=T-V. Is there any issues with my setup?
 !
!