Binding Energy per Nucleon trend for fusion vs fission

[Guest432]

Hello all!

In my Nuclear Power assignment I decided to analyse this graph:

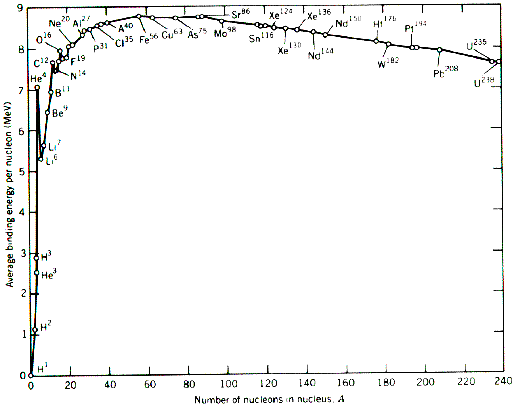
I mention that
"The difference in atomic mass and binding energy per nucleon for deuterium and helium (fusion elements) is ≈3u and 5.96 MeV respectively. However, for all elements past Iron (fission elements) the difference in any two binding energies is < 1.3 MeV. Such a vast energy yield, even in comparison to fission, is the reason why obtaining net power from fusion is such a holy grail."

However, I am perplexed as to why exactly the difference in binding energy of elements before Iron is so high! I have discussed the fact neutrons increase the distance between protons in nuclei, so I am taking a guess that elements with a low atomic mass have less neutrons, ergo more repulsive force and greater binding energy. However, this logic seems weak and doesn't really explain why the trend of binding energy per nucleon is what it is.

Thanks :)

 

[e.bar.goum]

The Semi-Empirical Mass Formula gets you a long way to replicating the trend in BE/A. I would strongly suggest that a good way to understand this trend is to understand each term in the SEMF. Plot BE/A for each term in the SEMF separately, and look at the contribution of each one.

https://en.wikipedia.org/wiki/Semi-empirical_mass_formula
http://www.hep.ph.ic.ac.uk/~dauncey/will/lecture19.pdf

 

[mfb]

I have discussed the fact neutrons increase the distance between protons in nuclei,

I don't understand that statement.

Such a vast energy yield, even in comparison to fission, is the reason why obtaining net power from fusion is such a holy grail.

The energy density is not the key point. The atoms used in fusion are also cheap and available everywhere in the world, their fusion product helium is not radioactive and you have no risk of a runaway reaction.

 

[Guest432]

I don't understand that statement.The energy density is not the key point. The atoms used in fusion are also cheap and available everywhere in the world, their fusion product helium is not radioactive and you have no risk of a runaway reaction.

Was told this by my physics teacher.

The energy density point is good, but it still doesn't explain to me why exactly the graph trends rapidly upwards then slowly downwards.

 

[mfb]

Ah, well, that is a bit hand-waving, because it does not explain why the size of the nucleus increases with more nucleons. Nucleons are not billard balls, they do not occupy a volume.

The energy density point is good, but it still doesn't explain to me why exactly the graph trends rapidly upwards then slowly downwards.

That was just meant as comment on commercial applications. See @e.bar.goum's answer for a good model of the shape of that curve.