- #1
yopy
- 43
- 0
Hi, I am currently doing a practice test for my final exam coming up, I am wondering anyone can double check the questions to see if i did them write, below is a picture of the questions, the answers i got are listed at the bottom,
If you could, please post whether you agree with my answers to any of the following or if you disagree.
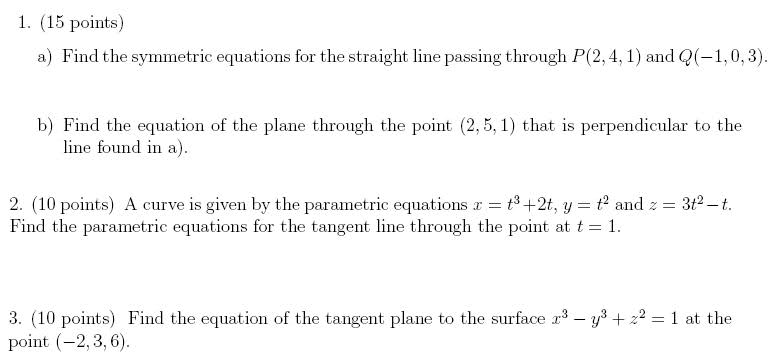
for 1 A) i got, (X-2)/-3=(Y-4)/-4=(Z-1)/2
for 1 B) after simplifying i came up with -3x-4y+2z=-24
For 2) i got the following,
x= 3+5t
y=1+2t
z=2+5t
for 3) after simplifying i got, 3x-2y-5z=-42
If you could, please post whether you agree with my answers to any of the following or if you disagree.
Homework Statement
for 1 A) i got, (X-2)/-3=(Y-4)/-4=(Z-1)/2
for 1 B) after simplifying i came up with -3x-4y+2z=-24
For 2) i got the following,
x= 3+5t
y=1+2t
z=2+5t
for 3) after simplifying i got, 3x-2y-5z=-42