Can someone solve this exponential equation for me?

[Ameer Bux]

2 = X to the power x to the power x, until infinity.

I really need to know how its possible for the answer to be √2

 

[mgkii]

Not totally sure I follow the logic here. It feels like it should be correct... but I'm going to have to read this a few more times! Taken from http://mathforum.org/

Dear Dr. Math,

What is the value of x in the following equation:

' ...
' x
' x
' x
' x
'x = 2

Thank you.

Benno

Date: 10/14/97 at 19:15:09
From: Doctor Tom
Subject: Re: algebra

There's a trick to solving this problem instantly. Since the exponents
go on forever, the exponent of the lowest and leftmost x is the same
as x to that power, so replace the equation by

2
x = 2

So x is the square root of 2, or 1.4142135...

 

[TeethWhitener]

Plug in $\sqrt{2}$ for $x$ and solve for $y$ like so:
$$y = \sqrt{2}^{\sqrt{2}^\ldots}$$
Since the power tower goes to infinity, this is equivalent to:
$$y=(\sqrt{2})^y$$
Do you see why? Now solve for $y$. (Or if you like, just plug in $y=2$ and verify that the equation is true.)

 

[BvU]

[edit] I goofed here! see further down...

Well, I think you have a problem. Since $$\left (\left ( \sqrt 2 \right )^\sqrt 2 \right )^\sqrt 2 = 2 $$ I think it should stop at $$ 2 = x^{x^x}$$

You are being lured into the reasoning of post #2, but it's a circular reasoning: IF $$2 = 2^{x^{x^{x^{x^{x^{x^{x^{...}}}}}}}} $$ THEN x =2 . If not, then not!

 

[TeethWhitener]

Well, I think you have a problem. Since $$\left (\left ( \sqrt 2 \right )^\sqrt 2 \right )^\sqrt 2 = 2 $$ I think it should stop at $$ 2 = x^{x^x}$$

You are being lured into the reasoning of post #2, but it's a circular reasoning: IF $$2 = 2^{x^{x^{x^{x^{x^{x^{x^{...}}}}}}}} $$ THEN x =2 . If not, then not!

The problem here is that
$$((\sqrt{2})^{\sqrt{2}})^{\sqrt{2}} \neq \sqrt{2}^{\sqrt{2}^{\sqrt{2}}}$$ because exponentiation is not associative.

 

[BvU]

Ah! It's me making the mistake ? What are the conventions then to interpret $\sqrt{2}^{\sqrt{2}^{\sqrt{2}}}$ ?

Got it !

From here :

Without parentheses to modify the order of calculation, by convention the order is top-down, not bottom-up:

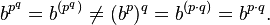
Note that some computer programs (notably Microsoft Office Excel) associate to the left instead, i.e. a^b^c is evaluated as (a^b)^c.

And I was just as dumb as XL...

 

[TeethWhitener]

Maybe it's different for you, but I've always seen
$$\sqrt{2}^{\sqrt{2}^{\sqrt{2}}} = \sqrt{2}^{\bigg(\sqrt{2}^{\sqrt{2}}\bigg)} $$
It's too early for this much nested LaTeX.

 

[BvU]

Yeah, it is. (here it is too late, actually -- time to go home ).

But thanks for putting us me right !

 

[Martin Rattigan]

The "trick" mentioned by Dr. Tom does in this instance give a correct solution, but it's not a proof. As mentioned by Peter Winkler in his book "Mathematical Puzzles" one has only to consider the similar equation $$x^{x^{x^{.^{.^.}}}}=4$$
Using the same logic gives $x^4=4$, i.e. $x=\sqrt{2}$. If the "proof" were correct we would then have $$2=\sqrt{2}^{\sqrt{2}^{\sqrt{2}^{.^{.^.}}}}=4$$

(Not to mention that $x^2=2$ actually has two solutions.)

 

[zinq]

If a tower of exponentials, defined as the limit of x_n as n → ∞, where for some real c > 0 we define

x₁ = c
​

and

x_n+1 = c^x_n,​

actually converges, then it can be proved that c satisfies the inequality

e^-e ≤ c e^1/e,​

(where e is the famous mathematical constant e = 2.718281828...) or in decimals,

0.065988... ≤ c ≤ 1.444667...,​

and in fact x_n will converge for all such c.

It's rather unusual to have a situation like this where the endpoints of the region of convergence are included in the region of convergence!

It's not hard to show that, if we do have convergence to some number S = S(c):

lim_n→∞ x_n = S​

then we must also have

c^S = S,​

as suggested above. But, this does not mean that any S satisfying the above equation is necessarily the limit of the x_n.

So in particular, the fact that the 4th power of √2 is 4 does not mean that 4 is the limit of the x_n for the value c = √2. It most certainly is not.

In fact, it is known that for the lower end of the range of c that gives a convergent tower, we have:

for c = e^-e, S = 1/e,​

and at the high end, we have:

for c = e^1/e, S = e.​

These facts rule out, for instance, the possibility that for c = √2 we have S = 4.

 

[Martin Rattigan]

I was actually playing Devil's advocate to point out that there was something missing from the original logic.

I did prove that $\lim_{n \to \omega}(\sqrt{2} \uparrow\uparrow n)=2$ without establishing the convergence range you give. The solution $x=-\sqrt{2}$ also needs to be discounted.