You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
What is Exponential: Definition and 1000 Discussions
In mathematics, the exponential function is the function
f
(
x
)
=
e
x
,
{\displaystyle f(x)=e^{x},}
where e = 2.71828... is Euler's constant.
More generally, an exponential function is a function of the form
f
(
x
)
=
a
b
x
,
{\displaystyle f(x)=ab^{x},}
where b is a positive real number, and the argument x occurs as an exponent. For real numbers c and d, a function of the form
f
(
x
)
=
a
b
c
x
+
d
{\displaystyle f(x)=ab^{cx+d}}
is also an exponential function, since it can be rewritten as
a
b
c
x
+
d
=
(
a
b
d
)
(
b
c
)
x
.
{\displaystyle ab^{cx+d}=\left(ab^{d}\right)\left(b^{c}\right)^{x}.}
The exponential function
f
(
x
)
=
e
x
{\displaystyle f(x)=e^{x}}
is sometimes called the natural exponential function for distinguishing it from the other exponential functions. The study of any exponential function can easily be reduced to that of the natural exponential function, since
a
b
x
=
a
e
x
ln
b
{\displaystyle ab^{x}=ae^{x\ln b}}
As functions of a real variable, exponential functions are uniquely characterized by the fact that the growth rate of such a function (that is, its derivative) is directly proportional to the value of the function. The constant of proportionality of this relationship is the natural logarithm of the base b:
d
d
x
b
x
=
b
x
log
e
b
.
{\displaystyle {\frac {d}{dx}}b^{x}=b^{x}\log _{e}b.}
For b > 1, the function
b
x
{\displaystyle b^{x}}
is increasing (as depicted for b = e and b = 2), because
log
e
b
>
0
{\displaystyle \log _{e}b>0}
makes the derivative always positive; while for b < 1, the function is decreasing (as depicted for b = 1/2); and for b = 1 the function is constant.
The constant e = 2.71828... is the unique base for which the constant of proportionality is 1, so that the function is its own derivative:
This function, also denoted as exp x, is called the "natural exponential function", or simply "the exponential function". Since any exponential function can be written in terms of the natural exponential as
b
x
=
e
x
log
e
b
{\displaystyle b^{x}=e^{x\log _{e}b}}
, it is computationally and conceptually convenient to reduce the study of exponential functions to this particular one. The natural exponential is hence denoted by
The former notation is commonly used for simpler exponents, while the latter is preferred when the exponent is a complicated expression. The graph of
y
=
e
x
{\displaystyle y=e^{x}}
is upward-sloping, and increases faster as x increases. The graph always lies above the x-axis, but becomes arbitrarily close to it for large negative x; thus, the x-axis is a horizontal asymptote. The equation
d
d
x
e
x
=
e
x
{\displaystyle {\tfrac {d}{dx}}e^{x}=e^{x}}
means that the slope of the tangent to the graph at each point is equal to its y-coordinate at that point. Its inverse function is the natural logarithm, denoted
log
,
{\displaystyle \log ,}
ln
,
{\displaystyle \ln ,}
or
log
e
;
{\displaystyle \log _{e};}
because of this, some old texts refer to the exponential function as the antilogarithm.
The exponential function satisfies the fundamental multiplicative identity (which can be extended to complex-valued exponents as well):
It can be shown that every continuous, nonzero solution of the functional equation
f
(
x
+
y
)
=
f
(
x
)
f
(
y
)
{\displaystyle f(x+y)=f(x)f(y)}
is an exponential function,
f
:
R
→
R
,
x
↦
b
x
,
{\displaystyle f:\mathbb {R} \to \mathbb {R} ,\ x\mapsto b^{x},}
with
b
≠
0.
{\displaystyle b\neq 0.}
The multiplicative identity, along with the definition
e
=
e
1
{\displaystyle e=e^{1}}
, shows that
e
n
=
e
×
⋯
×
e
⏟
n
factors
{\displaystyle e^{n}=\underbrace {e\times \cdots \times e} _{n{\text{ factors}}}}
for positive integers n, relating the exponential function to the elementary notion of exponentiation.
The argument of the exponential function can be any real or complex number, or even an entirely different kind of mathematical object (e.g., matrix).
The ubiquitous occurrence of the exponential function in pure and applied mathematics has led mathematician W. Rudin to opine that the exponential function is "the most important function in mathematics". In applied settings, exponential functions model a relationship in which a constant change in the independent variable gives the same proportional change (i.e., percentage increase or decrease) in the dependent variable. This occurs widely in the natural and social sciences, as in a self-reproducing population, a fund accruing compound interest, or a growing body of manufacturing expertise. Thus, the exponential function also appears in a variety of contexts within physics, chemistry, engineering, mathematical biology, and economics.
View More On Wikipedia.org
f
(
x
)
=
e
x
,
{\displaystyle f(x)=e^{x},}
where e = 2.71828... is Euler's constant.
More generally, an exponential function is a function of the form
f
(
x
)
=
a
b
x
,
{\displaystyle f(x)=ab^{x},}
where b is a positive real number, and the argument x occurs as an exponent. For real numbers c and d, a function of the form
f
(
x
)
=
a
b
c
x
+
d
{\displaystyle f(x)=ab^{cx+d}}
is also an exponential function, since it can be rewritten as
a
b
c
x
+
d
=
(
a
b
d
)
(
b
c
)
x
.
{\displaystyle ab^{cx+d}=\left(ab^{d}\right)\left(b^{c}\right)^{x}.}
The exponential function
f
(
x
)
=
e
x
{\displaystyle f(x)=e^{x}}
is sometimes called the natural exponential function for distinguishing it from the other exponential functions. The study of any exponential function can easily be reduced to that of the natural exponential function, since
a
b
x
=
a
e
x
ln
b
{\displaystyle ab^{x}=ae^{x\ln b}}
As functions of a real variable, exponential functions are uniquely characterized by the fact that the growth rate of such a function (that is, its derivative) is directly proportional to the value of the function. The constant of proportionality of this relationship is the natural logarithm of the base b:
d
d
x
b
x
=
b
x
log
e
b
.
{\displaystyle {\frac {d}{dx}}b^{x}=b^{x}\log _{e}b.}
For b > 1, the function
b
x
{\displaystyle b^{x}}
is increasing (as depicted for b = e and b = 2), because
log
e
b
>
0
{\displaystyle \log _{e}b>0}
makes the derivative always positive; while for b < 1, the function is decreasing (as depicted for b = 1/2); and for b = 1 the function is constant.
The constant e = 2.71828... is the unique base for which the constant of proportionality is 1, so that the function is its own derivative:
This function, also denoted as exp x, is called the "natural exponential function", or simply "the exponential function". Since any exponential function can be written in terms of the natural exponential as
b
x
=
e
x
log
e
b
{\displaystyle b^{x}=e^{x\log _{e}b}}
, it is computationally and conceptually convenient to reduce the study of exponential functions to this particular one. The natural exponential is hence denoted by
The former notation is commonly used for simpler exponents, while the latter is preferred when the exponent is a complicated expression. The graph of
y
=
e
x
{\displaystyle y=e^{x}}
is upward-sloping, and increases faster as x increases. The graph always lies above the x-axis, but becomes arbitrarily close to it for large negative x; thus, the x-axis is a horizontal asymptote. The equation
d
d
x
e
x
=
e
x
{\displaystyle {\tfrac {d}{dx}}e^{x}=e^{x}}
means that the slope of the tangent to the graph at each point is equal to its y-coordinate at that point. Its inverse function is the natural logarithm, denoted
log
,
{\displaystyle \log ,}
ln
,
{\displaystyle \ln ,}
or
log
e
;
{\displaystyle \log _{e};}
because of this, some old texts refer to the exponential function as the antilogarithm.
The exponential function satisfies the fundamental multiplicative identity (which can be extended to complex-valued exponents as well):
It can be shown that every continuous, nonzero solution of the functional equation
f
(
x
+
y
)
=
f
(
x
)
f
(
y
)
{\displaystyle f(x+y)=f(x)f(y)}
is an exponential function,
f
:
R
→
R
,
x
↦
b
x
,
{\displaystyle f:\mathbb {R} \to \mathbb {R} ,\ x\mapsto b^{x},}
with
b
≠
0.
{\displaystyle b\neq 0.}
The multiplicative identity, along with the definition
e
=
e
1
{\displaystyle e=e^{1}}
, shows that
e
n
=
e
×
⋯
×
e
⏟
n
factors
{\displaystyle e^{n}=\underbrace {e\times \cdots \times e} _{n{\text{ factors}}}}
for positive integers n, relating the exponential function to the elementary notion of exponentiation.
The argument of the exponential function can be any real or complex number, or even an entirely different kind of mathematical object (e.g., matrix).
The ubiquitous occurrence of the exponential function in pure and applied mathematics has led mathematician W. Rudin to opine that the exponential function is "the most important function in mathematics". In applied settings, exponential functions model a relationship in which a constant change in the independent variable gives the same proportional change (i.e., percentage increase or decrease) in the dependent variable. This occurs widely in the natural and social sciences, as in a self-reproducing population, a fund accruing compound interest, or a growing body of manufacturing expertise. Thus, the exponential function also appears in a variety of contexts within physics, chemistry, engineering, mathematical biology, and economics.
View More On Wikipedia.org
-

I Double exponential integral
Hello frens, How should one approach this sort of integral? Any tips would be appreciated. Let's say we have $$ \int_{(1)}^{(2)}\exp\left[ a+b\exp\left[ f(x) \right] \right]dx$$ ...where the limits of integration are not important. Any tips? Thanks!- Steve Zissou
- Thread
-
- Tags
- Approach Exponential Integral
- Replies: 3
- Forum: Calculus
-
C
I Numerically how to approximate exponential decay in a discrete signal
Given a vector of numbers, say [exp(-a t) ] for t - [1, 2, 3, 4, 5] and choose maybe a = -2.4, how can I approximate -2.4 from using Laplace transform methods? I know you can use regression for this, but I'd like to know the Laplace transform (or Z-transform since it is discrete) approach.- cppIStough
- Thread
- Replies: 4
- Forum: General Math
-
T
I Integrating a product of exponential and trigonometric functions
I am looking for a closed form solution to an integral of the form: $$ \int_0^\infty \frac{e^{-Du^2t}u \sin{ux}}{u^2+h^2} du $$ D, t, and h are positive and x is unrestricted. I have tried everything, integration by parts, substitution, even complex integration with residue analysis. I've... -
L
How do you prove that ln(a^x) = xln(a) and a^x = e^xln(a) without using exponent rules?
In the book "Calculus by Michael Spivak" it says that a^x = e^xln(a) is a definition. And I am not convinced to accept this as true without a proof.- logicgate
- Thread
-
- Tags
- Exponential Ln Proof
- Replies: 6
- Forum: Calculus and Beyond Homework Help
-

Solve the problem that involves iteration
part (a) Asymptote at ##x=0.5## part (b) ##\dfrac{e^x}{4x^2-1}= -2## ##e^x=2-8x^2## ##2e^x= 4-16x^2## ##16x^2=4-2e^x## ##x^2= \dfrac{4-2e^x}{16}## ##x=\dfrac{\sqrt{4-2e^x}}{4}## part (c) ... ##x_{2}=0.2851## ##x_{3}=0.2894## ##x_{4}=0.2881## ##x_{5}=0.2885## ##x_{6}=0.2884##...- chwala
- Thread
-
- Tags
- Asymptote Exponential
- Replies: 21
- Forum: Precalculus Mathematics Homework Help
-

POTW Find Limit of $$\frac{x}{e} - \left(\frac{x}{x+1}\right)^x$$ at Infinity
Find the limit $$\lim_{x\to \infty} x\left[\frac{1}{e} - \left(\frac{x}{x+1}\right)^x\right]$$- Euge
- Thread
-
- Tags
- Exponential Infinity Limit
- Replies: 3
- Forum: Math POTW for University Students
-
T
I Identity involving exponential of operators
Hey all, I saw a formula in this paper: (https://arxiv.org/pdf/physics/0011069.pdf), specifically equation (22): and wanted to know if anyone knew how to derive it. It doesn't seem like a simple application of BCH to me. Thanks.- thatboi
- Thread
-
- Tags
- Exponential Identity Operators
- Replies: 2
- Forum: Quantum Physics
-
C
Is ##f(x)=2^{x}-1## considered an exponential function?
I wonder if it's ##f(x)=2^{x}-1## considered an exponential function because in my textbook it's stated that the set of values of an exponential function is a set of positive real numbers, while when graphing this function I get values(y line) that are not positive(graph in attachments), so I am...- Callmelucky
- Thread
- Replies: 12
- Forum: Precalculus Mathematics Homework Help
-
M
Computing the derivative of an exponential function
- member 731016
- Thread
- Replies: 10
- Forum: Calculus and Beyond Homework Help
-
M
B How do I invert this exponential function?
Preface: I have not done serious math in years. Today I tried to do something fancy for a game mechanic I'm designing. I've got an item with a variable power level. It uses x amount of ammo to produce f(x) amount of kaboom. Initially it was linear, e.g. fL(x) = x, but I didn't like the scaling... -
T
I Second Order ODE with Exponential Coefficients
Hi all, I have another second order ODE that I need help with simplifying/solving: ##p''(x) - D\frac{e^{\gamma x}}{A-Ae^{\gamma x}}p'(x) - Fp(x) = 0## where ##\gamma,A,F## can all be assumed to be nonzero real numbers and ##D## is a purely nonzero imaginary number. Any help would be appreciated!- thatboi
- Thread
- Replies: 1
- Forum: Differential Equations
-
D
MHB Is There an Exact Solution to the Equation \(4^x + 3^x = 12\)?
Solve $4^x+3^x=12$ without using CAS?- DrLiangMath
- Thread
-
- Tags
- Exponential
- Replies: 7
- Forum: General Math
-

I Curiosity: there exists the exponential integral?
Hi, my question regard the possibility to consider a generalization on the product integral (of type II). The product integral is defined in analogy to the definite integral where instead the limit of a sum there is a limit of a product and, instead the multiplication by ##dx## there is the...- Ssnow
- Thread
-
- Tags
- Curiosity Exponential Integral
- Replies: 3
- Forum: Calculus
-
A
Properties of limits of exponential functions
I did only the the first three prop. And on a means we have, on pose or posons means let there be , propriétés means properties, alors meand then. I apologize i am a french native speaker and i am busy so i cannot rewrite this in entirely english- A dummy progression
- Thread
- Replies: 5
- Forum: Calculus and Beyond Homework Help
-

Worth learning complex exponential trig derivations in precalc?
This is a pedagogical /time management / bandwidth / tradeoff question, no argument that learning the complex exponential derivation is valuable, but is it a good strategy for preparing for first year Calculus? my 16YO son is taking AP precalc and AP calc next year and doing well, but struggled...- BWV
- Thread
- Replies: 22
- Forum: STEM Educators and Teaching
-

MHB -s6.6 solve exponential eq 3^x-14\cdot 3^{-x}=5
\tiny{s464\\6.6} solve the exponential equation. $3^x-14\cdot 3^{-x}=5$ Rewrite $\quad 3^x+\dfrac{14}{3^x}=5$ $\times$ $\quad 3^x \quad 3^{2x}+14=5\cdot 3^x$ quadratic $\quad 3^{2x}-5\cdot3^x-14 =0$ Factor $\quad (3^x-7)(3^x+2)=0$ Discard $\quad 3^x =7$ hence $\quad...- karush
- Thread
-
- Tags
- Exponential
- Replies: 1
- Forum: General Math
-
T
Exponential potential energy state diagram
It is my second "energy state diagram problem" and I would want to know if I am thinking correctly. First I have done some function analysis to get a glimpse of the plot: - no roots but ##\lim\limits_{x\to-\infty}U(x)=\lim\limits_{x\to+\infty}U(x)=0## - y interception: ##U(0)=-U_0## - even...- ThEmptyTree
- Thread
- Replies: 13
- Forum: Introductory Physics Homework Help
-
J
I Is this business graph an exponential or polynomial function?
GRAPH WITH VALUES: Sorry I have a small dilema, I don't know if this is a exponential or polynomial function. I'd think its exponential but it doesn't have same change of factors.- jorgevf200002
- Thread
- Replies: 17
- Forum: Calculus
-

Integration of an exponential function
My trial : I think ## \int ~ dy ~ e^{-2 \alpha(y)} ## dose not simply equal: ## - \frac{1}{2}e^{-2 \alpha(y)} ## cause ##\alpha## is a function in ##y ##. So any help about the right answer is appreciated!- Safinaz
- Thread
- Replies: 4
- Forum: Calculus and Beyond Homework Help
-
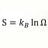
B Is this curve quadratic or exponential?
Does this curve look quadratic or exponential- morrobay
- Thread
-
- Tags
- Curve Exponential Quadratic
- Replies: 3
- Forum: General Math
-
J
Proof involving exponential of anticommuting operators
For ##N=1##, I have managed to prove this, but for ##N>1##, I am struggling with how to show this. Something that I managed to prove is that $$\langle\psi |b_k^\dagger=-\langle 0 | \sum_{n=1}^N F_{kn}c_n \prod_{m=1\neq k, l}^N \left(1+b_m F_{ml}c_l \right)$$ which generalizes what I initially...- Joker93
- Thread
- Replies: 1
- Forum: Advanced Physics Homework Help
-

Calculate (and argue) the critical points of an exponential function
Hi everybody We can't differentiate ##x^x## neither like a power function nor an exponential function. But ##x^x=e^{x\mbox{ln}x}##. So ##\dfrac{d}{dx}x^x=\dfrac{d}{dx}e^{x\mbox{ln}x}=x^x(\mbox{ln}x+1)## And here comes the doubt: prove the domain of ##x^x## is ##(0, +\infty)## Why is only...- mcastillo356
- Thread
- Replies: 10
- Forum: Calculus and Beyond Homework Help
-
T
MHB How Does Exponential Growth Affect a Bacterial Population?
A bacterial population x is known to have a growth rate proportional to x itself. If between 12 noon and 2:00 pm, the popilation triples. 1. What is the growth rate pf the given problem? 2. Is the number of bacterial population important to the problem? 3. At what time should x become 100...- Tineeyyy
- Thread
-
- Tags
- Exponential Growth
- Replies: 1
- Forum: Calculus
-
A Fourier Transform of an exponential function with sine modulation
I want to know the frequency domain spectrum of an exponential which is modulated with a sine function that is changing with time. The time-domain form is, s(t) = e^{j \frac{4\pi}{\lambda} \mu \frac{\sin(\Omega t)}{\Omega}} Here, \mu , \Omega and \lambda are constants. A quick...- tworitdash
- Thread
- Replies: 5
- Forum: Calculus
-
H
Question about the argument in a Complex Exponential
I know that e^-ix = cos(-x)-isin(x), but if we have e^-iwx does that equal cos(-wx) - isin(wx)? Thanks- Haku
- Thread
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-

I Integral involving exponential
Just a quick question: Does anybody know if there is a closed-form solution to this rather simple-looking definite integral? ##F(\lambda) = \int_0^{\infty} \dfrac{e^{-x}}{1 + \lambda x} dx## If ##\lambda > 0##, it definitely converges. It has a limit of 1 as ##\lambda \rightarrow 0##. But it...- stevendaryl
- Thread
-
- Tags
- Exponential Integral
- Replies: 9
- Forum: Calculus
-
P
Proving the existence of a real exponential function
Ok, first I tried to show that ##A = \left \{a^{r}|r\in\mathbb{Q},r<x \right \}## does not have a maximum value. Assume ##\left\{ a^{r}\right\}## has a maximum, ##a^{r_m}##. By this hypothesis, ##r_{m}<x## and ##r_{m}>r\forall r\neq r_{m}\in\mathbb{Q}##. Consider now that ## q\in\mathbb{Q}|q>0##...- Portuga
- Thread
- Replies: 5
- Forum: Calculus and Beyond Homework Help
-
A
Problem with series convergence — Taylor expansion of exponential
Good day and here is the solution, I have questions about I don't understand why when in the taylor expansion of exponential when x goes to infinity x^7 is little o of x ? I could undesrtand if -1<x<1 but not if x tends to infinity? many thanks in advance!- Amaelle
- Thread
- Replies: 4
- Forum: Calculus and Beyond Homework Help
-
L
A Exponential statistical process to be characterised
I'd be grateful for any formulation that describes this statistical process- Larry Lacey
- Thread
- Replies: 14
- Forum: Set Theory, Logic, Probability, Statistics
-
A
I Add an exponential number of elements, what will be the final cardinality?
Suppose we construct a set, adding at each step a polynomial number of elements. My impression that after we do countably infinite number of steps, the set will have countably infinite cardinality. But what happens if we add exponential number of elements each step? For instance, on step 0 we...- Anixx
- Thread
- Replies: 16
- Forum: Set Theory, Logic, Probability, Statistics
-
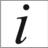
Proof that the exponential function is convex
I try to proof it but i got stuck right here, i want your opinions Can I get a solution if i continue by this way? or Do I have to take another? and if it is so, what would yo do?- L Navarro H
- Thread
- Replies: 5
- Forum: Calculus and Beyond Homework Help
-
U
MHB How to solve an expression with inverse of exponential distribution
I have an Energy harvesting expression something like the following $R = \tau B \log\Big(1 + \frac{E h^2}{\tau r^\alpha\sigma^2} \Big)$ $E = \tau(2^{R/\tau B}-1 )\frac{r^\alpha\sigma^2}{h^2}$ Let all constant terms as $a$ to simplify the expression into : $E = a\frac{1}{h^2}$ $E$ is a random...- user_01
- Thread
- Replies: 1
- Forum: Set Theory, Logic, Probability, Statistics
-

Problem solving an exponential limit
I know it's probably an easy one, but I'm getting confused on how to treat that exponential numerator in order to escape from the indeterminate form ##\frac{0}{0}##- greg_rack
- Thread
- Replies: 27
- Forum: Precalculus Mathematics Homework Help
-
M
Mean and var of an exponential distribution using Fourier transforms
Hi, I was just thinking about different ways to use the Fourier transform in other areas of mathematics. I am not sure whether this is the correct forum, but it is related to probability so I thought I ought to put it here. Question: Is the following method an appropriate way to calculate the...- Master1022
- Thread
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-

Exponential distribution probability exercise
1) Since I want at least ##6## flights to come within ##2## hours, then the time interval between each should be, at worse, ##2/6=1/3## hours, and the probability is ##P(X\leq1/3)=1-e^{-1/3}##. 2) The probability that at best 5 airplanes arrive at the airport is...- archaic
- Thread
- Replies: 13
- Forum: Calculus and Beyond Homework Help
-
A
Exponential Wavefunction for Infinite Potential Well Problem
Using the boundary conditions where psi is 0, I found that k = n*pi/a, since sin(x) is zero when k*a = 0. I set up my normalization integral as follows: A^2 * integral from 0 to a of (((exp(ikx) - exp(-ikx))*(exp(-ikx) - exp(ikx)) dx) = 1 After simplifying, and accounting for the fact that...- a1234
- Thread
- Replies: 7
- Forum: Advanced Physics Homework Help
-

I Scalar powers of a matrix exponential
Starting from the definition of a matrix exponential as a power series, how would we show that ##(e^A)^n=e^{nA}##? I know how to show that if A and B commute then ##e^Ae^B = e^{A+B}## and from this we can show that the first identity is true for integer values of n, but how can we show it’s...- Hiero
- Thread
-
- Tags
- Exponential Matrix Scalar
- Replies: 6
- Forum: General Math
-

Solving a Pendulum Problem with Exponential Solution
a) This looks somewhat like a pendulum problem (length ##\ell/2##). I reasoned there will be a clockwise rotation, and that the acceleration is due to the force of...- archaic
- Thread
-
- Tags
- Exponential Pendulum
- Replies: 23
- Forum: Introductory Physics Homework Help
-
A
Complex numbers: convert the exponential to polar form
Summary:: Hello, my question asks if the complex exponential equation 4e^(-j) is equal to 4 ∠-180°. I tried to use polar/rectangular conversions: a+bj=c∠θ with c=(√a^2 +b^2) and θ=tan^(-1)[b/a] 4e^(-j)=4 ∠-180° c=4, 4=(√a^2 +b^2) solving for a : a=(√16-b^2) θ=tan^(-1)[b/a]= -1 b/(√16-b^2)=...- ac7597
- Thread
- Replies: 9
- Forum: Precalculus Mathematics Homework Help
-

How does this exponential decay formula get derived?
https://www.asi.edu.au/wp-content/uploads/2015/03/PhysicsASOE2013soln.pdf Q12 e) Working backwards, P = Ae^kt form, i.e. EAts = Emin e^(ln2/τ x t). Not sure how they get this formula in the first place with these values.- aspodkfpo
- Thread
- Replies: 7
- Forum: Introductory Physics Homework Help
-

I Exponential of momenta to entangle harmonic oscillators
Consider two harmonic oscillators, described by annihilation operators a and b, both initially in the vacuum state. Let us imagine that there is a coupling mechanism governed by the Hamiltonian H=P_A P_B, where P_i is the momentum operator for the oscillator i. For example P_A =...- matteo137
- Thread
- Replies: 2
- Forum: Quantum Physics
-

MHB Exponential distribution question
Hi, I want to know how the highlighted steps are arrived at in the first page. What are \(R_X (y), R'_X (y),F'_X (0) ? \)How \(R_X (0) = 1 ?\) Solution to differential equation should be \(R_X (y)=K*e^{\int{R'_X (0) dx}}\) But it is different. How is that? What is $-R'_X...- WMDhamnekar
- Thread
- Replies: 1
- Forum: Set Theory, Logic, Probability, Statistics
-

What is the Exponential Fourier Transform of an Even Function?
From the sketch, I know that this function is an even function. So, I simplify the Fourier transform in the limit of the integration (but still in exponential form). Then, I try to find the exponential FOurier transform. Here what I get: $$g(a)=\frac{2}{2\pi} \int_{0}^{\infty} e^{-x} e^{-iax}...- agnimusayoti
- Thread
- Replies: 8
- Forum: Calculus and Beyond Homework Help
-

Expressing a vector in the exponential form
I managed to expand a general expression from the alternatives that would leave me to the answer, that is: I will receive the alternatives like above, so i find the equation: C = -sina, P = cosa So reducing B: R: Reducing D: R: Is this right?- LCSphysicist
- Thread
-
- Tags
- Exponential Form Vector
- Replies: 2
- Forum: Calculus and Beyond Homework Help
-
I
I Matrices Commuting with Matrix Exponential
The summary pretty much explains my question. I know that ##\left[ A, e^B \right]=0## if ##[A,B]=0## (and can prove it), but I can't figure out how to prove if it is or is not an "if and only if" statement. Thanks in advance!- Isaac0427
- Thread
-
- Tags
- Exponential Matrices Matrix
- Replies: 30
- Forum: Linear and Abstract Algebra
-
M
B Exponential formula for Excel sheet
Hello, i am testing batteries for a project of mine. I first measure the voltage on the battery (OCV, Open Circuit Voltage), and then i place a load resistor over the battery. This results in a voltage drop with a exponential decrease in voltage like in the image. But i am working with a...- MrNewton
- Thread
-
- Tags
- Excel Exponential Formula
- Replies: 2
- Forum: Classical Physics
-

Question regarding the exponential of a matrix
Hi, I think this is a nitpicking question, but oh well let me hear your inputs. Actually I tried to solve this question straightforwardly, by Taylor expanding the exponential and showing that: \textbf{A}^n = \begin{pmatrix} a^n & nba^{n-1} \\ 0 & a^n \end{pmatrix} i.e. e^{\textbf{A}t} =...- ChrisVer
- Thread
-
- Tags
- Exponential Matrix
- Replies: 8
- Forum: Calculus and Beyond Homework Help
-
J
MHB Exponential functions (calculator exercise)
Hi there, I have tried to do these questions but I don't understand. Any help would be appreciated!- Joshuaniktas
- Thread
-
- Tags
- Exercise Exponential Functions
- Replies: 1
- Forum: General Math
-

Evaluating an integral of an exponential function
the integral is: and according to mathematica, it should evaluate to be: . So it looks like some sort of Gaussian integral, but I'm not sure how to get there. I tried turning the cos function into an exponential as well: however, I don't think this helps the issue much.- dykuma
- Thread
- Replies: 3
- Forum: Calculus and Beyond Homework Help
-

MHB Inequality of cubic and exponential functions
Prove that $3^n\ge(n+3)^3$ for any natural number $n\ge6$.- anemone
- Thread
- Replies: 1
- Forum: General Math