- #1
Isomorphism
- 8
- 1
1. Homework Statement :
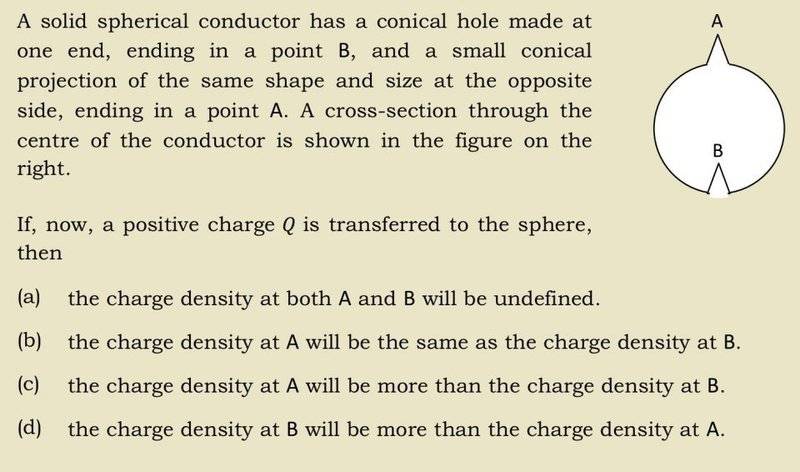
So I think it is option (a). Am I right?
Thanks.
Homework Equations
:[/B] A conductor is an equipotential surface. The charge density near a conductor is proportional to the electric field. Electric field is the negative gradient of potential and thus electric field is in a direction normal to the surface.The Attempt at a Solution
: [/B]Since electric field is the gradient of potential, if the surface of a conductor has sharp turns, the gradient will be undefined (cannot draw a tangent plane at such points) and thus the charge density is undefined.So I think it is option (a). Am I right?
Thanks.