Confused as to what forces are acting on the ladder

[Scrambler]

Homework Statement

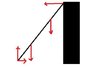
Mr. X ( mass 96.0 kg ), he just finished a rather large breakfast) is finally getting around to taking down his holiday lights when a sudden ice storm hits. the coefficient of static friction between his ladder (mass 16.4 kg ) and the level ground has been reduced to 0.090. To reach the lights Mr. X must climb 4.20 m up the 5.00m ladder. To improve on his frictional force, Mr.X gets Mr.Y to stand on the first rung of the ladder which is located 0.42m from the ground, will Mr. X reach the lights safely? There is no friction between the ladder and the wall and the ladder makes an angle of 68° with the ground.

Homework Equations

Fun = ma
torque = R. F.
Ff= fn ( coefficient of friction)

The Attempt at a Solution

i really don't know where to start, what should i calculate first, i tried to calculate the amount of force that the co-efficient of friction can handle but i don't know how to do this, and I'm confused as to what forces are acting on the ladder.

i know that there will be a force off the wall, a normal force off of the ground and a gravity force from Mr.Y, but do i take account a gravitational force from Mr.X even though he's moving up the ladder ?

 

[bakin]

but do i take account a gravitational force from Mr.X even though he's moving up the ladder ?

You aren't really interested in him moving up the ladder though. In your problem, it asks whether he's going to fall when he reaches 4.20m up the ladder.

I would first draw a picture showing all the forces. Remember the weight of the ladder, too.

 

[xxChrisxx]

draw a fbd of the problem

 

[Scrambler]

okay so first i have to find the components that will make all the forces acting on the ladder perpendicular.

I will choose the very bottom of the ladder as my pivot point therefore eliminating the normal force from the ground and the frictional force on the ladder. because when R= 0, torque = 0.

so

Fw ( force of wall ) sin 68 ( 5 m ) = Force of gravity Mr. X ( 4.2m) + force gravity Mr. Y ( 0.42m) + force gravity ladder ( 2.1 m)

then find the force of the wall and use the sum of the forces in the x direction must = 0 to find the frictional force ! but

i don't get how i will find out if he falls or not ?

 

[bakin]

Draw a picture first.

You should have three equilibrium equations, correct? You're going to need those.

What I would do is leave the friction force as a variable for now, that way you can find out just how much friction force is needed for the ladder to be in equilibrium. Then once you find that value, compare it with the maximum value (based on your coefficient of static).

 

[Scrambler]

okay, I've uploaded a diagram so should the force off the wall equal the frictioanl force in equilubrium ?