- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading Andrew Browder's book: "Mathematical Analysis: An Introduction" ... ...
I am reading Chapter 13: Differential Forms ... ... and am currently focused on Section 13.1 Tensor Fields ...
I need some help in order to fully understand some statements by Browder in Section 13.1 ... ...
Section 13.1 reads as follows:
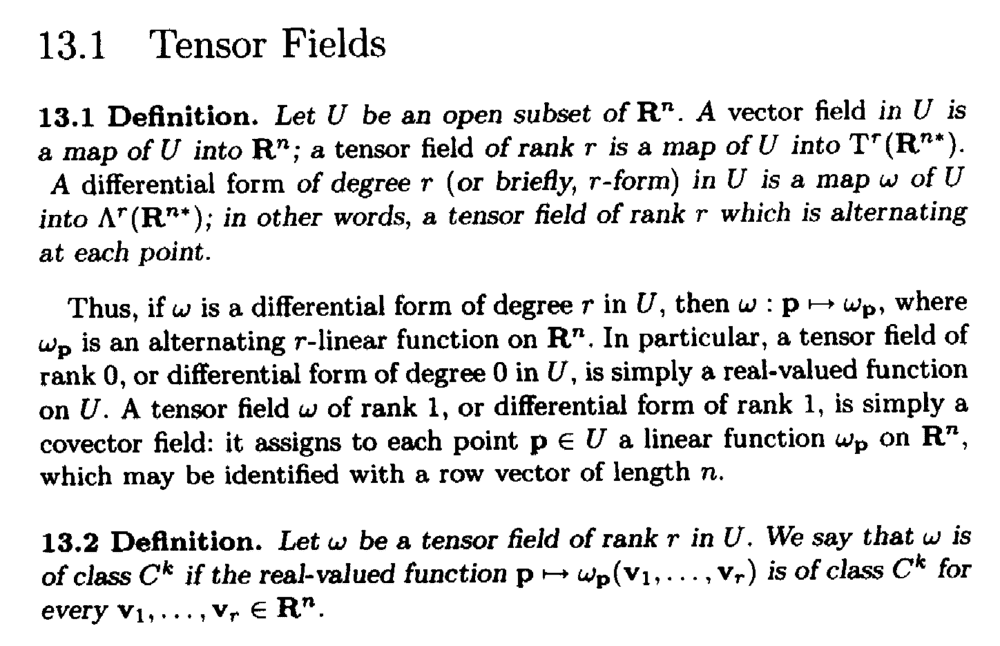
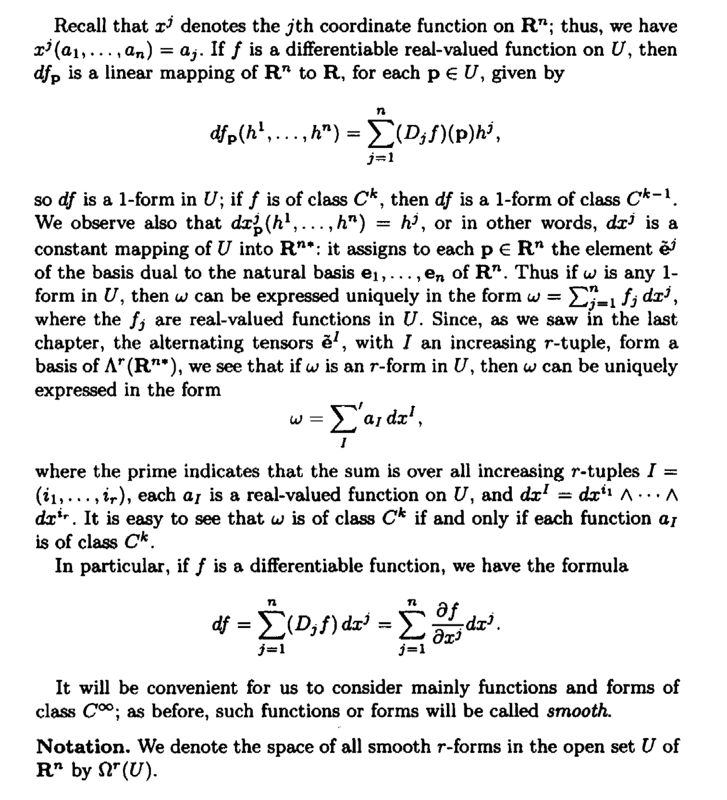
In the above text we read the following:
" ... ... We observe also that ##\text{dx}_P^j (h^1, \cdot \cdot \cdot , h^n) = h^j## ... ... "My question is what is the nature of the ##h^i## ... given that ##\text{dx}_P^j## is a constant mapping from an open subset ##U## of ##\mathbb{R}^n## it seems that the ##h^i## are real numbers ...
... BUT ... then it seems strange that ##\text{dx}_p^j (h^1, \cdot \cdot \cdot , h^n) = h^j## ... that is ... how can the function evaluate to a real number when it is a constant mapping into ##\mathbb{R}^{ n \ast }## ... it should evaluate to a linear functional, surely ...
... indeed ... should Browder have written ...
##\text{dx}_P^j (h^1, \cdot \cdot \cdot , h^n) = \tilde{e}^j##
Can someone please clarify the above ...
Peter
I am reading Chapter 13: Differential Forms ... ... and am currently focused on Section 13.1 Tensor Fields ...
I need some help in order to fully understand some statements by Browder in Section 13.1 ... ...
Section 13.1 reads as follows:
In the above text we read the following:
" ... ... We observe also that ##\text{dx}_P^j (h^1, \cdot \cdot \cdot , h^n) = h^j## ... ... "My question is what is the nature of the ##h^i## ... given that ##\text{dx}_P^j## is a constant mapping from an open subset ##U## of ##\mathbb{R}^n## it seems that the ##h^i## are real numbers ...
... BUT ... then it seems strange that ##\text{dx}_p^j (h^1, \cdot \cdot \cdot , h^n) = h^j## ... that is ... how can the function evaluate to a real number when it is a constant mapping into ##\mathbb{R}^{ n \ast }## ... it should evaluate to a linear functional, surely ...
... indeed ... should Browder have written ...
##\text{dx}_P^j (h^1, \cdot \cdot \cdot , h^n) = \tilde{e}^j##
Can someone please clarify the above ...
Peter
Attachments
-
 Browder - Defn of a differential form .png59.4 KB · Views: 314
Browder - Defn of a differential form .png59.4 KB · Views: 314 -
 Browder - 2 - Start of Section 13.1 ... ... PART 2 ... .png60.3 KB · Views: 299
Browder - 2 - Start of Section 13.1 ... ... PART 2 ... .png60.3 KB · Views: 299 -
 Browder - 2 - Start of Section 13.1 ... ... PART 2 ... .png60.3 KB · Views: 299
Browder - 2 - Start of Section 13.1 ... ... PART 2 ... .png60.3 KB · Views: 299 -
 ?temp_hash=b63a36a349d9b9bbedf37aea4772fe69.png59.4 KB · Views: 395
?temp_hash=b63a36a349d9b9bbedf37aea4772fe69.png59.4 KB · Views: 395 -
 ?temp_hash=b63a36a349d9b9bbedf37aea4772fe69.png60.3 KB · Views: 362
?temp_hash=b63a36a349d9b9bbedf37aea4772fe69.png60.3 KB · Views: 362