- #1
albert1992
- 11
- 0
I have trouble disproving the following expression
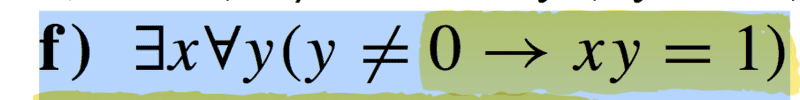
I worded it as follows:
The product of certain number and every other nonzero number is 1
I worded it as follows:
The product of certain number and every other nonzero number is 1