Does the Wigner-Eckart theorem require good quantum numbers?

[Decimal]

I have a question related to the following passage in the quantum mechanical scattering textbook by Taylor,

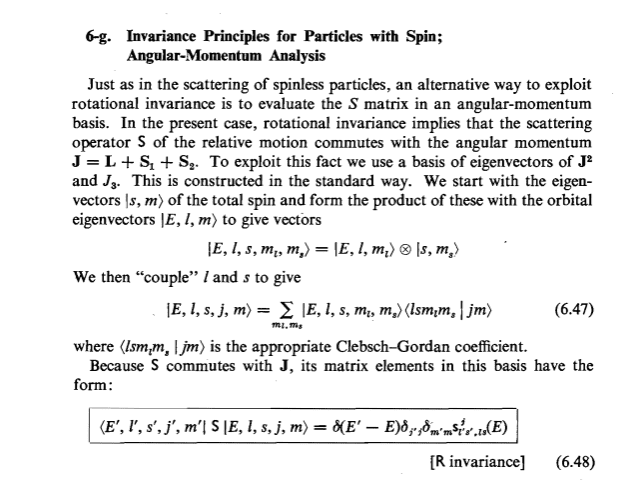
Here Taylor makes the choice to use a basis of total angular momentum eigenvectors instead of using the simple tensor product given in the first equation above (6.47). I understand that this is because $j$ is conserved in this case, so the $|E, l,s, j, m\rangle$ states consist of good quantum numbers which are clearly convenient. However in the way it is worded by Taylor I think he may also imply that we should use these states such that equation 6.48 holds, i.e. such that we may apply the Wigner-Eckart theorem to the scattering matrix $S$. In my understanding of the Wigner-Eckart theorem this is not a requirement, and we could just as well have written something like, $$\langle l', s', m_{l'}, m_{s'}|S|l, s, m_{l}, m_{s} \rangle = \delta_{ll'} \delta_{m_l m_{l'}}\frac{\langle l' ,s',m_{s'}||S||l, s, m_s\rangle }{\sqrt{2l+1}}$$ where I have now made the decision to not couple $l$ and $s$ but rather apply Wigner-Eckart to the $l$ states only. I realize that this is probably not the most convenient choice in this case, but I am just wondering if its still correct. The reason I am asking is that I have to set up a scattering theory in a much more complicated system with additional nuclear spin and an external magnetic field, such that the total angular momentum is not a good quantum number. I would however still like to apply the Wigner-Eckart theorem to the scattering matrix if possible.

 

[HAYAO]

I'm not a physicist and not very knowledgeable in quantum mechanics so I also want to understand this.

Since I don't have the textbook I don't know the ultimate goal of this section, but from what I read here, the author is attempting to prove that the rotational invariance means that scattering operator commutes with total angular momentum. From my understanding, total angular momentum operator is a generator of rotation operator of the entire system (including both orbital and spin) so that's what I speculate the author focused on. So I don't really see the point in attempting to evaluate $S$ without total angular momentum. If you only consider the $l$ states, then you would only be rotating the spatial position of electrons without spin. So like you said, this would be inappropriate in this section.

Wigner-Eckart theorem does require good quantum numbers, but if you are ignoring spin-orbit coupling $l$, $s$, $j$, and $m$ are all "good" quantum numbers. I'm assuming if you do the calculations, they should all commute with the scattering operator; since the author says "Just as in the scattering of spinless particles...", the previous section must've been about commutation relationship of $l$ operator with $S$, so at least we have one example there probably, and another one in the end of this section. Even if you include spin-orbit coupling, $j$ and $m$ are still "good" quantum numbers, so I don't see a problem in the author's statement.

If you must include spin-orbit coupling, only $j$ and $m$ are going to be good quantum numbers, but if you want to start with the basis of L and S eigenstate in the L-S coupling scheme, and then you would have to apply spin-orbit coupling operator to it. The resulting wavevectors are going to be expressed with the linear combinations of these basis (mixes the states with same $j$).

If you are going to apply nuclear spin and external magnetic field, I'm completely clueless. If they are relatively small interactions, then you might be able to apply perturbation theory. In the evaluation of the transition dipole moments of lanthanides, crystal field is important but it is small enough to be treated as perturbation. In this case, we start with basis of L and S angular momentum eigenstates in L-S coupling scheme for 4f-electrons without spin-orbit coupling. Then we apply electrostatic interaction and spin-orbit coupling. Finally, we use these 4f states with crystal field operator in perturbation that admixes 4f5d states. I'm hoping that you can do something similar in your system if your external field can be treated as a perturbation.

(If I'm making a stupid error here, then please point it out. It really helps me understand.)

 

[Decimal]

Thank you for the response HAYAO, I have since discussed this problem with my supervisors as well.

Your point on the spin orbit coupling was actually very helpful, I had (stupidly) not considered yet that this is the actual reason $l$ and $s$ can no longer be considered good quantum numbers and should be coupled. Taylor also doesn't mention this explicitly, so I had assumed it is a more general property of angular momentum theory rather than a consequence of spin orbit coupling.

In the literature on the actual problem I am studying I can only assume then that there is an implicit assumption that the spin orbit coupling is ignored. Let me give an overview of the actual problem. We have two particles $j$ of electronic spin $\mathbf{s}_j$ and nuclear spin $\mathbf{i}_j$, which scatter in a magnetic field. The total Hamiltonian reads, $$H = H^0 + \sum_j \left[H_j^{\mathrm{hf}} + H_j^{\mathrm{Z}} \right] + V.$$ Here $H_j^{\mathrm{hf}} \sim \mathbf{s}_j \cdot \mathbf{i}_j$ denotes the hyperfine interaction and $H_j^{\mathrm{Z}} \sim \mathbf{s}_j \cdot \mathbf{B} + \mathbf{i}_j \cdot \mathbf{B}$ is the Zeeman coupling to the magnetic field. Let us for now ignore the potential $V$. At zero magnetic field eigenstates of the Hamiltonian are then formed as $\ket{\chi} = \ket{f_1, m_{f_1}} \ket{f_2, m_{f_2}}$, where $\mathbf{f}_j = \mathbf{s}_j + \mathbf{i}_j$ is the total spin angular momentum. At nonzero magnetic field $f_j$ is no longer a good quantum number and these basis states mix. Let us then call the total eigenstates of the Hamiltonian (still without $V$) $\ket{\alpha}$, which will be superpositions of $\ket{\chi}$ states with magnetic field dependent expansion coefficients. The question is now how to form a partial wave expansion of this problem. If there exists no spin orbit coupling I would think that writing something like this for the scattering matrix is correct, $$\langle l', m_{l'}, \alpha' | S | l, m_l, \alpha \rangle = \delta_{l'l} s_{l' \alpha', l \alpha}^l.$$ Here I have neglected the kinetic energy terms since it is not so important for this problem. I think this obeys Wigner-Eckart since the orbital angular momentum is conserved, and $S$ will commute with $L^2$ and $L_z$. If the interaction $V$ is included I think this still holds as long as the potential is spherically symmetric, but I don't have direct proof for this.

If spin-orbit coupling is included as you said the problem seems to become very difficult. I think at that point the partial wave expansion is possible in theory but doesn't really make sense anymore practically. Any spherical symmetry is broken and the scattering matrix is not diagonal in any quantum number, so why would you even go to the effort. I suppose that in literature the neglect of spin orbit coupling is usually implicit, but I have not yet found any text that looks at this problem rigorously. It seems like most authors just assume that an expansion in terms of partial waves is possible without justifying what this means for the scattering matrix.