- #71
zoobyshoe
- 6,510
- 1,290
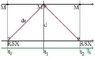
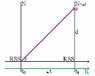
OK. My next step, I believe, should be to look at the distance L. In the inertial frame of the station the distance L = 300,000 km. It will not be the same distance to the ship by virtue of the ships speed. To the ship the length L will be contracted. The ship will find L to measure something less than 300,000 km.
jcsd has provided the formula [tex]\gamma L[/tex] to use to determine what the ship will find L to be from its perspective.
He has provided this formula:
[tex]\gamma = \frac{1}{\sqrt{1 - \beta^2}}[/tex]
to describe "gamma"([tex]\gamma[/tex]).
The value I got when I solved for gamma earlier using .5 c for the relative velocity of the ship and the station was
1.1547005.
1.1547005 times 300,000 = 346,410.15 km
346,410.15 km > 300,000, not < 300,000.
Instead of contracting, the length has dilated!
jcsd has given us the wrong formula. He has given us the formula for time dilation, not length contraction.
The formula for length contraction is simply:
[tex]\sqrt {1 - \frac {v^2}{c^2}}[/tex]
the result is the percentage of the length that the observer will see that length contracted to.
In our example, the ship will see the 300,000 km to have contracted to .8660254 % of its original length.
That comes out to be 300,000 times .8660254 = 259,807.62 km.
jcsd has provided the formula [tex]\gamma L[/tex] to use to determine what the ship will find L to be from its perspective.
He has provided this formula:
[tex]\gamma = \frac{1}{\sqrt{1 - \beta^2}}[/tex]
to describe "gamma"([tex]\gamma[/tex]).
The value I got when I solved for gamma earlier using .5 c for the relative velocity of the ship and the station was
1.1547005.
1.1547005 times 300,000 = 346,410.15 km
346,410.15 km > 300,000, not < 300,000.
Instead of contracting, the length has dilated!
jcsd has given us the wrong formula. He has given us the formula for time dilation, not length contraction.
The formula for length contraction is simply:
[tex]\sqrt {1 - \frac {v^2}{c^2}}[/tex]
the result is the percentage of the length that the observer will see that length contracted to.
In our example, the ship will see the 300,000 km to have contracted to .8660254 % of its original length.
That comes out to be 300,000 times .8660254 = 259,807.62 km.