Find the linear speed of the particle when system rotates about axis

[Aurelius120]

Homework Statement

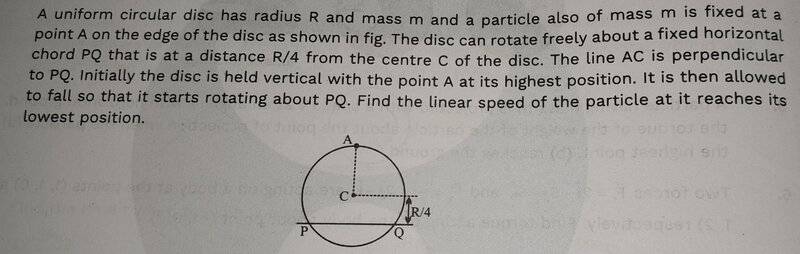
A uniform circular disc radius R and mass M with a particle of mass M fixed on edge. The system is free to rotate about chord PQ. It is allowed to fall from vertical. Find the linear speed of the particle when it reaches the lowest point.

Relevant Equations

$P.E.=\int{Fdx}$

Question image:

The question should be solved by conservation of mechanical energy.( I assume surface density$\sigma$ and acceleration due to gravity$g=const.$)Therefore:
$$PE_i+KE_i=PE_f+KE_f$$
The axis of rotation $PQ$ is line of zero potential. Then
1) $PE_i=\int Fdy$
Since coordinates of center are $(0,\frac{R}{4})$ I define the integral as:
$$PE_i=\int^{R}_{-R}\left( 2g \sigma \left(y+\frac{R}{4}\right) \sqrt{R^2-y^2}\right)dy +\frac{5MgR}{4}$$ y is with respect to center.

2) $$KE_i=0$$
3) $$PE_f=-PE_i$$
4) $$KE_f=\frac{I\omega^2}{2}=\left( \frac{MR^2}{4}+\frac{MR^2}{16}+\frac{25MR^2}{16} \right)\frac{\omega^2}{2}$$

I thought I had it but then integration struck and I could not do it no more. Moreover the final answer keeps giving a $pi$(wrong integration on my part I guess) term that does not cancel.

So my questions are :
1) How to find the potential energy of the system?
2) Is there any other method that could give answers faster?
3) Finally is my integral correct? Any hint/help in further solving it?

 

[Aurelius120]

I looked for the solution online but there is no explanation of how they find the potential energies. Just this:

Thank you

 

[kuruman]

So my questions are :
1) How to find the potential energy of the system?
2) Is there any other method that could give answers faster?
3) Finally is my integral correct? Any hint/help in further solving it?

(1) Consider that the external force of gravity acts at the center of gravity. That's what the solution that you found did.
(2) See (1) above.
(3) No need to integrate. See (1) above.

 

[Aurelius120]

(1) Consider that the external force of gravity acts at the center of gravity. That's what the solution that you found did.
(2) See (1) above.
(3) No need to integrate. See (1) above.

Ok but just for the sake of it, is the integral correct? You know in case it had to be proven that the center of gravity method is correct

 

[kuruman]

Ok but just for the sake of it, is the integral correct?

Finish integrating and if you get $PE_i=mg\left(R+\dfrac{R}{4}\right)+mg\dfrac{R}{4}$, then it is correct.

 

[haruspex]

I guess because the particle is at A . $5R/4$ above reference line

Whoops! I was misled by the diagram, which makes it look at least R/2.

 

[Aurelius120]

Have evaluated the integral. It was correct. Adding it for future reference.
$$PE_{i_{ disc}}=\int^{R}_{-R}\left( 2g \sigma \left(y+\frac{R}{4}\right) \sqrt{R^2-y^2}\right)dy$$
$$=\int^{R}_{-R}\left( 2g \sigma

y \sqrt{R^2-y^2}\right)dy +\int^{R}_{-R}\left( 2g \sigma \frac{R}{4}\sqrt{R^2-y^2}\right)dy$$
$$=\int^{R}_{-R}\left(- g \sigma \sqrt{R^2-y^2}\right)d(R^2-y^2) +\int^{R}_{-R}\left( 2g \sigma \frac{R^2}{4}\sqrt{1-\frac{y^2}{R^2}}\right)dy$$
$$=\left[-\frac{ 2g \sigma}{3} {\sqrt{R^2-y^2}}^3\right]^{R}_{-R}+\int^{R}_{-R}\left( 2g \sigma \frac{R^2}{4}\sin \theta \right)dy$$

$$\frac{y}{R}=\cos\theta \implies \frac{dy}{d\theta}=-R\sin \theta$$
$$=0+\int^{R}_{-R}\left( 2g \sigma \frac{R^3}{4}(-\sin^2 \theta) \right)d\theta$$

$$=\int^{R}_{-R}\left( 2g \sigma \frac{R^3}{4}×\frac{\cos(2 \theta)-1}{2} \right)d\theta$$

$$=\frac{g \sigma R^3}{4}\left[ \sin \theta \cos \theta-\theta\right]^{R}_{-R}$$

$$=\frac{g \sigma R^3}{4}\left[ \frac{y}{R}\sqrt{1-\frac{y^2}{R^2}}-\cos^{-1}(y/R)\right]^{R}_{-R}$$

$$=\frac{g \sigma R^3}{4}\left[ \frac{y}{R}\sqrt{1-\frac{y^2}{R^2}}-\cos^{-1}(y/R)\right]^{R}_{-R}$$
$$=\frac{\sigma g R^3 \pi}{4}=\frac{mgR}{4}$$