Finding Vector C: Magnitude and Angle Calculation

[Koko23]

Homework Statement

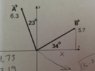
Vector C is given by C=B-A. The magnitude of vector A is 6.3 units and 23 degrees from the y axis in quadrant II. The magnitude of vector B is 5.7 units and 34 degrees from the x axis in quadrant I. What is the magnitude of vector C? What is the angle measured from the x axis to vector C in degrees?

Relevant Equations

Law of Cosine and Sine

Ax=6.3 cos 23; Ay=-6.3 sin 23; Bx= 5.7 cos 34; By=5.7 sin 34. Is this correct to calculate vector C magnitude which I got 7.7 units. Also is vector C in quadrant IV?
I am not sure how to calculate the angle part of this question.

 

[kuruman]

Hi Koko23 and welcome to PF.

How are the quadrants labeled I, II, III and IV, clockwise or counterclockwise? Which component(s) is (are) negative in quadrant II? Make a drawing to guide your thinking and try to draw it to scale as best as you can. Then you will see how to figure out the angle part with some simple trigonometry.

 

[Koko23]

This is the diagram for the problem. I drew the arrow pointing downward since it was vector B - vector A.

 

[neilparker62]

Determine the x and y components of the vectors you have drawn and add them up (as you've done - more or less). Then you can draw a single right angled triangle in which the Hypotenuse is the resultant and angle (from x-axis) can be determined with simple trig.

Alternatively if you want to use cosine and sine rules, I would suggest you draw your vectors tail to head.

 

[mjc123]

"Ax=6.3 cos 23; Ay=-6.3 sin 23;"

You have Ax and Ay the wrong way round.

 

[Koko23]

Ax=-6.3 sin 23 = -2.46; Ay=6.3 cos 23 = 5.8; Bx=5.7 cos 34 =4.73; By=5.7 sin 34 = 3.19
Sqrt((Bx-Ax)^2 + (By-Ay)^2) = 7.7 units for the magnitude of C.

For the angle measured from the x-axis to vector c, I got -20 which is the angle 20 degrees below the x-axis to vector c using tan.