Flywheel Leg Press Design - How is this being calculated?

[lucioc]

Hello guys,

I'm looking for some help. I'm reading a paper called "On Flywheel Leg Press Design and its Benefits". I'm interested in calculate the forces that a flywheel machine that I'm building (used for gym exercises) will require the user to use it. Following this paper, they start to calculate the flywheel mass, and they predetermine a range of forces and acceleration values in a table. This is what appears on the paper:

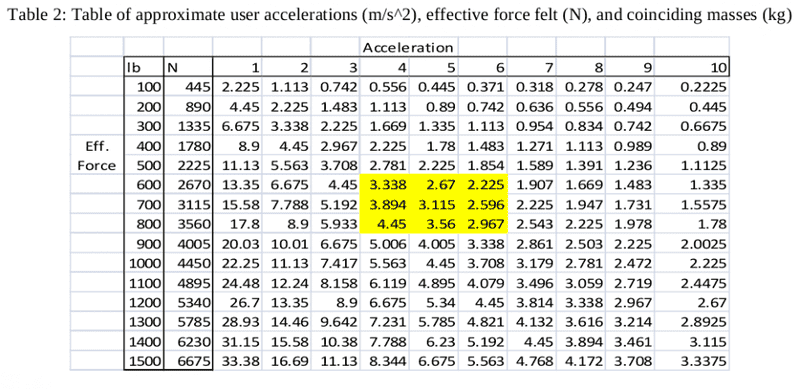
So, if I understood it correctly, when we have a force F = 2670N, and a acceleration a = 4 m/s² the mass of the flywheels are 3.338Kg. In this special case they use two flywheels, with 2 discs each (not sure how this influence). The problem is that when I try to use the functions they talk about using the values on the table, I'm not able to get the same result... like:

m = 2 * ( 2670N / 4m/s² ) * (0.3048² / 0.1524²) = 1335 * 4 Kg

That doesn't make sense. I'm probably getting some unit wrong or missing some equation in the middle. Can someone help to find out how they calculate the mass? Maybe the paper is not so clear in this. I want to understand it first so I can calculate the force giving I already have a mass and acceleration.

Thanks for any help,
Lucio

 

[lucioc]

Ok I find out one issue from the equation I was trying. Ro is the big radius, and not Rt. So:

m = 2 * ( 2670N / 4m/s² ) * (0.1524² / 0.3048² ) = 1335 * 0.25 Kg = 333.75

So this is basically 100times the amount of mass that is posted on the table 333.8 vs 3.338. Why is 100 times more? Why would I divide by 100?

 

[lucioc]

any help?

 

[bikengr]

Hi, I imagine I could help. Feel free to email me at bikengr AT netnet.net

 

[bikengr]

I can't tell if this forum is posting my input. Anyway, looks like they have typos in their use of R0, RT

 

[lucioc]

I can't tell if this forum is posting my input. Anyway, looks like they have typos in their use of R0, RT

What do you mean?

 

[billy_joule]

Ok I find out one issue from the equation I was trying. Ro is the big radius, and not Rt. So:

m = 2 * ( 2670N / 4m/s² ) * (0.1524² / 0.3048² ) = 1335 * 0.25 Kg = 333.75

So this is basically 100times the amount of mass that is posted on the table 333.8 vs 3.338. Why is 100 times more? Why would I divide by 100?

For starters, your R_t and R_o do not have the same ratio as they specify. Their applied force is close to the hub, 1/12 or 1/24 of the radius which is 1'' for both the 1' and 2' flywheels.

Although, increasing R_t is not a bad idea, it reduces the required strength of the belt and R_t changes less, proportionally, as the belt wraps on itself around the pulley (or is that a design feature?)

I wouldn't put too much stock in that source paper, It doesn't seem particularly rigorous.

http://www.worldacademicunion.com/journal/SSCI/SSCIvol07no02paper03.pdf

 

[lucioc]

For starters, your R_t and R_o do not have the same ratio as they specify. Their applied force is close to the hub, 1/12 or 1/24 of the radius which is 1'' for both the 1' and 2' flywheels.

Although, increasing R_t is not a bad idea, it reduces the required strength of the belt and R_t changes less, proportionally, as the belt wraps on itself around the pulley (or is that a design feature?)

I wouldn't put too much stock in that source paper, It doesn't seem particularly rigorous.

http://www.worldacademicunion.com/journal/SSCI/SSCIvol07no02paper03.pdf

Could you clarify a little more? Rt is supossed to be 1'' then, what means, 0.0254 meters ? And R0 is what, the radius of the disc? In this case, 0.3048 and 0.1524, or not?

At the end I just want to understand how I will apply the formula to my case, with only one single disc, giving I have the mass and just want to calculate the force to be applied by the user. In my case, what would be my Rt and R0? Giving a similar configuration besides the number of discs.

 

[billy_joule]

Could you clarify a little more? Rt is supossed to be 1'' then, what means, 0.0254 meters ? And R0 is what, the radius of the disc? In this case, 0.3048 and 0.1524, or not?

Yes R₀ is the disc radius. They use both 1' and 2' discs so it could be either. A quick skim of the paper and it's not clear which the table refers to.

At the end I just want to understand how I will apply the formula to my case, with only one single disc, giving I have the mass and just want to calculate the force to be applied by the user. In my case, what would be my Rt and R0? Giving a similar configuration besides the number of discs.

You know the mass of the flywheel? What is that based on?

The equation you used in #2 has five variables, you need to input four to find the unknown fifth. You can't find both R_t and R₀, but you can find their ratio. But you need to know m, F and a.
Remember F is the required force for a given state, whether the user can or will actually apply that force or not is a totally different question.

There are some common sense constraints eg
R_t < R₀ ≤ R_max (Where you've set R_max )
If you know your belt material you can determine an upper limit for F.
If you know your flywheel material you can determine a reasonable thickness range.
a and F probably depend on who'll be using the machine, Schwarzenegger or someone's great grandma. etc etc

Have you thought about how you'll use this thing? Will you have to manually wind the belt on? How will you ensure the belt is wound on and off reliably?
I can imagine it being much more difficult to use than regular weights.

 

[lucioc]

Yes R₀ is the disc radius. They use both 1' and 2' discs so it could be either. A quick skim of the paper and it's not clear which the table refers to.
You know the mass of the flywheel? What is that based on?

The equation you used in #2 has five variables, you need to input four to find the unknown fifth. You can't find both R_t and R₀, but you can find their ratio. But you need to know m, F and a.
Remember F is the required force for a given state, whether the user can or will actually apply that force or not is a totally different question.

There are some common sense constraints eg
R_t < R₀ ≤ R_max (Where you've set R_max )
If you know your belt material you can determine an upper limit for F.
If you know your flywheel material you can determine a reasonable thickness range.
a and F probably depend on who'll be using the machine, Schwarzenegger or someone's great grandma. etc etc

Have you thought about how you'll use this thing? Will you have to manually wind the belt on? How will you ensure the belt is wound on and off reliably?
I can imagine it being much more difficult to use than regular weights.

Let's say I have the mass m=2kg. I have the disc radius, let's say r0 = 0.15m. I want to find which would be the force needed by the user to perform the exercise. I will assume, like the paper did, accelerations ranging from 1 to 10m/s².

So now I need Rt, but giving I will build this thing, I imagine I should know / choose it right? So I though the Rt value was the radius of the second disc, but no. So what is Rt? Is Rt the radius of the axle where the belt is fixed on? If I choose it to be something like 2cm, can I do this?

a = 10m/s²
r0 = 0.15m
m = 2kg
Rt = 0.02m
F = (10 / 2)*2*(0.15² / 0.02²) = 562,5N

 

[billy_joule]

Let's say I have the mass m=2kg. I have the disc radius, let's say r0 = 0.15m. I want to find which would be the force needed by the user to perform the exercise. I will assume, like the paper did, accelerations ranging from 1 to 10m/s².

So now I need Rt, but giving I will build this thing, I imagine I should know / choose it right? So I though the Rt value was the radius of the second disc, but no. So what is Rt? Is Rt the radius of the axle where the belt is fixed on? If I choose it to be something like 2cm, can I do this?

Rt is the radius at which the force is applied from:
τ = Fr
Torque = Force * radius
This is the applied torque that causes the acceleration of the flywheel.
In your case the force is the belt tension and the radius is the belt drum/axle radius. Whether 2cm is a good value depends on the design, how will you attach the belt to the axle, does the change in Rt due to belt wrap matter, what is Fmax of the belt etc etc
Exercise equipment needs to be robust, you can't predict how and by who it will be used.
I'm sure many people and companies have gone bankrupt due to legal action over poor design leading to injury.
Remember that once the user pushes away and gets the flywheel spinning the foot rest is coming back towards them whether they are prepared or not, a significant quantity of energy can be stored in that flywheel. what if their feet slip off? What if they slip forward off the seat? etc

a = 10m/s²
r0 = 0.15m
m = 2kg
Rt = 0.02m
F = (10 / 2)*2*(0.15² / 0.02²) = 562,5N

That's right for your inputs.
Their calculations ignore all other rotating mass (axle, flywheel mount etc) so the actual force required will be greater. How much greater depends on the design.