- #1
Lambda96
- 158
- 59
- Homework Statement
- see post
- Relevant Equations
- none
Hi,
unfortunately I have several problems with the following task:
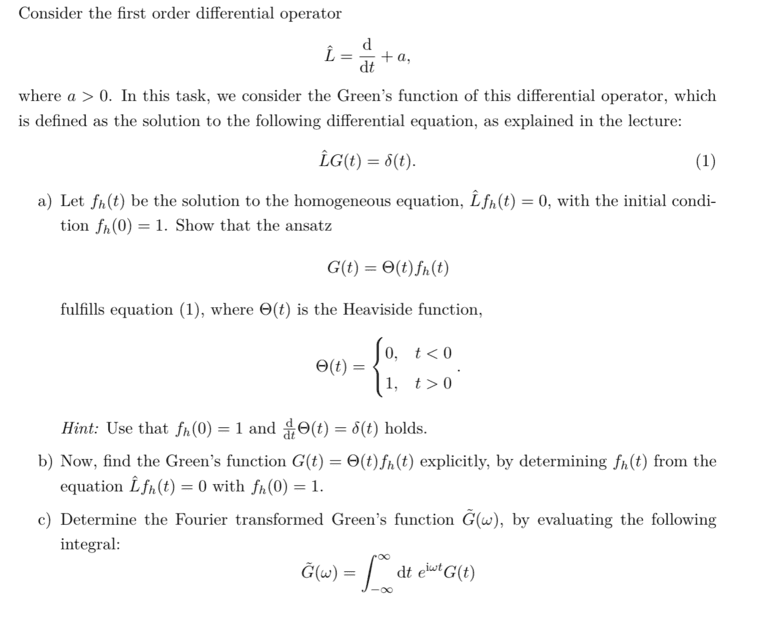
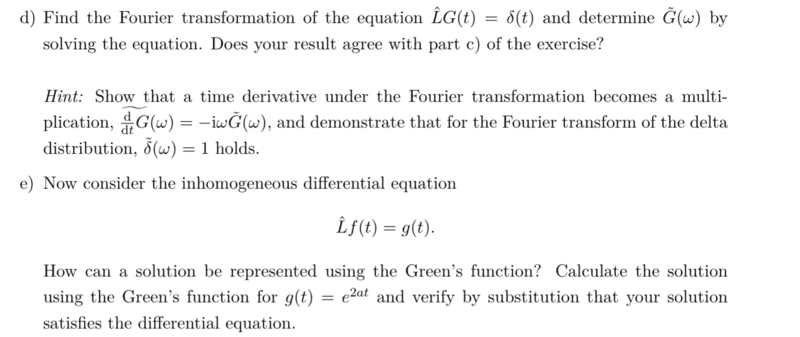
I have problems with the tasks a, d and e
Unfortunately, the Green function and solving differential equations with the Green function is completely new to me
In task b, I got the following for ##f_h(t)=e^{-at}##.Task a
$$\hat{L}G(t)=\Bigl( \frac{d}{dt} +a \Bigr) \Theta(t) f_h(t)$$
$$\hat{L}G(t)=\frac{d}{dt}\Theta(t) f_h(t) +a \Theta(t) f_h(t)$$
$$\hat{L}G(t)=\delta(t) f_h(t) + \Theta(t) f'_h(t) +a \Theta(t) f_h(t)$$
$$\hat{L}G(t)=\delta(t) f_h(t) -a \Theta(t) f_h(t) +a \Theta(t) f_h(t)$$
$$\hat{L}G(t)=\delta(t) f_h(t)$$
Can I now argue as follows that ##\hat{L}G(t)=\delta(t)## so when I multiply the operator by the Green function, I always get only one value. Then the following ##\delta(t)=\delta(0)## applies, so it follows that ##\delta(t) f_h(t)=\delta(0) f_h(0)## and since ##f_h(0)=1## only ##\delta(t)## remains on the left side of the equationTask d
I assumed that I should calculate the following integral.
$$\tilde{G}(\omega)= \int_{-\infty}^{\infty} dt \ e^{i \omega t} \hat{L} G(t) $$
$$ \tilde{G}(\omega)=\int_{-\infty}^{\infty} dt \ e^{i \omega t} \frac{d}{dt} G(t) +e^{i \omega t} a G(t) $$
$$ \tilde{G}(\omega)=\int_{-\infty}^{\infty} dt \ e^{i \omega t} \frac{d}{dt} G(t) +\int_{-\infty}^{\infty} dt \ e^{i \omega t} a G(t) $$
I then applied partial integration for the first integral
$$ \tilde{G}(\omega)=\biggl[ e^{i \omega t} G(t) \biggr]_{-\infty}^{\infty}-\int_{-\infty}^{\infty} dt \ i \omega e^{i \omega t} G(t) +\int_{-\infty}^{\infty} dt \ e^{i \omega t} a G(t) $$
Now, unfortunately, I don't get any further and I can't do anything with the hint from the task at the moment.
Task e
I thought that a solution may look like the following.
$$ f(t)= \int_{0}^{t} G(t)g(t) dt $$
I then calculated the following integral
$$ f(t)= \int_{0}^{t} G(t)g(t) dt $$
$$ f(t)= \int_{0}^{t} e^{-at} e^{2at} dt $$
$$ f(t)= \frac{e^{at} -1}{a}$$
If I substitute this ##f(t)## into ##\hat{L}f(t)##, I get ##2e^{at}-2## but I should get ##e^{2at}##.
unfortunately I have several problems with the following task:
I have problems with the tasks a, d and e
Unfortunately, the Green function and solving differential equations with the Green function is completely new to me
In task b, I got the following for ##f_h(t)=e^{-at}##.Task a
$$\hat{L}G(t)=\Bigl( \frac{d}{dt} +a \Bigr) \Theta(t) f_h(t)$$
$$\hat{L}G(t)=\frac{d}{dt}\Theta(t) f_h(t) +a \Theta(t) f_h(t)$$
$$\hat{L}G(t)=\delta(t) f_h(t) + \Theta(t) f'_h(t) +a \Theta(t) f_h(t)$$
$$\hat{L}G(t)=\delta(t) f_h(t) -a \Theta(t) f_h(t) +a \Theta(t) f_h(t)$$
$$\hat{L}G(t)=\delta(t) f_h(t)$$
Can I now argue as follows that ##\hat{L}G(t)=\delta(t)## so when I multiply the operator by the Green function, I always get only one value. Then the following ##\delta(t)=\delta(0)## applies, so it follows that ##\delta(t) f_h(t)=\delta(0) f_h(0)## and since ##f_h(0)=1## only ##\delta(t)## remains on the left side of the equationTask d
I assumed that I should calculate the following integral.
$$\tilde{G}(\omega)= \int_{-\infty}^{\infty} dt \ e^{i \omega t} \hat{L} G(t) $$
$$ \tilde{G}(\omega)=\int_{-\infty}^{\infty} dt \ e^{i \omega t} \frac{d}{dt} G(t) +e^{i \omega t} a G(t) $$
$$ \tilde{G}(\omega)=\int_{-\infty}^{\infty} dt \ e^{i \omega t} \frac{d}{dt} G(t) +\int_{-\infty}^{\infty} dt \ e^{i \omega t} a G(t) $$
I then applied partial integration for the first integral
$$ \tilde{G}(\omega)=\biggl[ e^{i \omega t} G(t) \biggr]_{-\infty}^{\infty}-\int_{-\infty}^{\infty} dt \ i \omega e^{i \omega t} G(t) +\int_{-\infty}^{\infty} dt \ e^{i \omega t} a G(t) $$
Now, unfortunately, I don't get any further and I can't do anything with the hint from the task at the moment.
Task e
I thought that a solution may look like the following.
$$ f(t)= \int_{0}^{t} G(t)g(t) dt $$
I then calculated the following integral
$$ f(t)= \int_{0}^{t} G(t)g(t) dt $$
$$ f(t)= \int_{0}^{t} e^{-at} e^{2at} dt $$
$$ f(t)= \frac{e^{at} -1}{a}$$
If I substitute this ##f(t)## into ##\hat{L}f(t)##, I get ##2e^{at}-2## but I should get ##e^{2at}##.