Interesting Thermodynamics Problem deleted in PhysicsStackExchange

[Chestermiller]

TL;DR Summary

Thermodynamics Learning Problem

I came across this very interesting Thermodynamics problem in PhysicsStackExchange. It was deleted by the OP because the moderators, in their infinite wisdom, gave him a hard time about its being a homework problem which was, in their opinion, a "check my work" post, rather than a "I'm having difficulty solving this" post. In the end, he became frustrated, received no help, and deleted his thread. Here is the problem statement. His difficulty was with part b, which should be our focus.

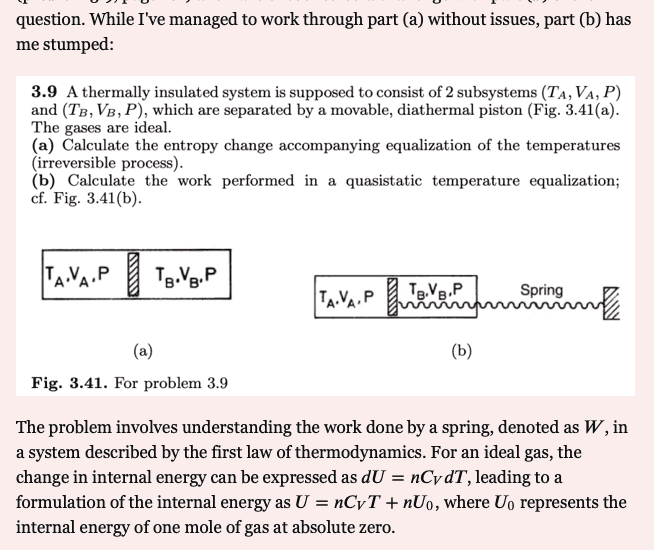
I personally had difficulty correctly analyzing this, and it took me about a day to finally reason it out. In my judgment, this problem would be worth considering by PF members studying Thermodynamics and others as well. Maybe even the guy who originally submitted it to StackExchange might come across this, and participate.

I am inviting PF members to participate in discussing and solving this problem. Again, the focus is part b.

 

[Andrew Mason]

I am confused by the P being the same on both sides.

If it starts with the frictionless piston at rest and the spring stretched from its equilibrium position, the pressure on the right side must be greater than the left by $\Delta P = kx/A$ (where x is the displacement of the piston from its equilibrium position and A is the area of the piston/cylinder) to make the force on each side of the piston equal. So:

$P_A=P_B-kx/A$

If it starts with the pressures on both sides being equal, the piston is moving initially to the right because there is a net force of $-kx/A$ on the piston. But that makes no sense because the spring is stretched and there is no mechanism for having that stretch unless it comes from higher pressure on the right.

AM

 

[Chestermiller]

I am confused by the P being the same on both sides.

If it starts with the frictionless piston at rest and the spring stretched from its equilibrium position, the pressure on the right side must be greater than the left by $\Delta P = kx/A$ (where x is the displacement of the piston from its equilibrium position and A is the area of the piston/cylinder) to make the force on each side of the piston equal. So:

$P_A=P_B-kx/A$

If it starts with the pressures on both sides being equal, the piston is moving initially to the right because there is a net force of $-kx/A$ on the piston. But that makes no sense because the spring is stretched and there is no mechanism for having that stretch unless it comes from higher pressure on the right.

AM

The spring is initially unstretched, so the two pressures are initially equal. The temperature difference causes the hotter cas to cool and the colder gas to heat up. This generates a pressure difference and causes the spring to stretch or compress.

 

[Charles Link]

Thank you @Chestermiller . It is certainly very interesting, and I think I am close to a solution of sorts. I need to check the algebra, and I got a cubic expression in $\Delta V_A$ when I set the temperatures the same for the final state. I used the initial internal energy is equal to the present internal energy plus the energy stored in the spring. I also used $P_B=P_A-k \Delta V_A /A^2$. I got expressions for $T_A$ and $T_B$ as functions of $\Delta V_A$ and then set $T_A=T_B$. I need to check my algebra though.

 

[Chestermiller]

Thank you @Chestermiller . It is certainly very interesting, and I think I am close to a solution of sorts. I need to check the algebra, and I got a cubic expression in $\Delta V_A$ when I set the temperatures the same for the final state. I used the initial internal energy is equal to the present internal energy plus the energy stored in the spring. I also used $P_B=P_A-k \Delta V_A /A^2$. I got expressions for $T_A$ and $T_B$ as functions of $\Delta V_A$ and then set $T_A=T_B$. I need to check my algebra, but I got the expression for $\Delta V_A$ is the solution of a quadratic equation in $\Delta V_A$ if $V_{Ao}=V_{B_o}$.

Hey Charles,

This approach sounds fundamentally correct. I set up the energy balances two ways. Method 1 treated the overall system, including both compartments, the partition, and the spring, as a an overall system. Method 2 treated the gases in the two compartments as separate systems. Adding the two energy balances obtained by Method 2 together then produced the equation from Method 1. It looks like you used Method 1. Please let us see you derivation.

Please also show us your derivations of the remainder of equations that can be used to establish the final state.

Also, the problem statement asks for the work, but it doesn't describe which work this is. If you derive the energy balance using Method 1, I contend that the overall system does no work. The spring energy comes into this energy balance based on the general form of the 1st law of thermodynamics on the left hand side of the equation, which includes not only kinetic energy change and potential energy change but also stored elastic energy change.

 

[Charles Link]

$U_o=3/2(P_A V_A+P_B V_B)+kx^2/2$ where $x=\Delta V_A/A$ which I will now start to abbreviate $\Delta V_A$ as $X$, with $\Delta V_A=-\Delta V_B$.

Now $P_B=P_A-kX/A^2$. With $V_A=V_{Ao}+X$ and $V_B=V_{Bo}-X$
we have

$3/2(P_A V_o)=U_o+(3/2)kV_{Bo}X/A^2-2kX^2/A^2$, where I did some algebra to get this result.

Here $V_o=V_{Ao}+V_{Bo}$.

Now $P_A V_A=N_A RT_A$ and $P_B V_B =N_B R T_B$.

We can then put in for $P_A$ to get $T_A$ in terms of $X$.
We can also get the $P_B$ expression to give a $T_B$ in terms of $X$.
We then set $T_A=T_B$, and solve for $X$. It turns out to be a cubic in $X$ with the constant term being $T_{Ao}-T_{Bo}$.

 

[Chestermiller]

$U_o=3/2(P_A V_A+P_B V_B)+kx^2/2$ where $x=\Delta V_A/A$ which I will now start to abbreviate $\Delta V_A$ as $X$, with $\Delta V_A=-\Delta V_B$.

Now $P_B=P_A-kX/A^2$. With $V_A=V_{Ao}+X$ and $V_B=V_{Bo}-X$
we have

$3/2(P_A V_o)=U_o+(3/2)kV_{Bo}X/A^2-2kX^2/A^2$, where I did some algebra to get this result.

Here $V_o=V_{Ao}+V_{Bo}$.

Now $P_A V_A=N_A RT_A$ and $P_B V_B =N_B R T_B$.

We can then put in for $P_A$ to get $T_A$ in terms of $X$.
We can also get the $P_B$ expression to give a $T_B$ in terms of $X$.
We then set $T_A=T_B$, and solve for $X$. It turns out to be a cubic in $X$ with the constant term being $T_{Ao}-T_{Bo}$.

This looks basically right to me. My approach was a little different. For the energy balance, I had $$n_AC_A(T_f-T_{A,0})+n_BC_B(T_f-T_{B,0})=-\frac{k}{2A^2}(\Delta V)^2$$where $$n_A=\frac{PV_{A,0}}{RT_{A,0}}$$ and $$n_B=\frac{PV_{B,0}}{RT_{B,0}}$$Solving for $T_f$ gives: $$T_f=\frac{n_AC_AT_{A,0}+n_BC_BT_{B.0}}{n_AC_A+n_BC_B}-\frac{k}{2A^2}\frac{(\Delta V)^2}{n_AC_A+n_BC_B}$$This equation applies only when the final state has been reached.

The other equation is $$P_{A,f}=P_{B,f}+\frac{k}{A^2}\Delta V$$or$$RT_f\left(\frac{n_A}{(V_A+\Delta V)}-\frac{n_B}{(V_B-\Delta V)}\right)=\frac{k}{A^2}\Delta V$$

 

[Charles Link]

@Chestermiller Your solution looks good to me. :) For the energy balance , you basically write the terms with $T_A-T_{Ao}$ and $T_B-T_{Bo}$ then set $T_A=T_B=T_f$.

Meanwhile my chemistry is a little rusty=I should be using small $n$ for the number of moles instead of capital $N$.

 

[Charles Link]

and a follow-on: I checked/compared the solutions for $\Delta V$ that we both got. Putting $C_A=C_B=(3/2)R$ for your molar heat capacities, and looking at the constant term and the cubic term in $\Delta V$ that is obtained from the equations, we are in agreement.

I didn't check the linear or quadratic terms, but no doubt those are also in agreement.

Both solutions with a little algebra give a constant term that is proportional to $T_{Bo}-T_{Ao}$ in the cubic equation for $\Delta V$.

and thank you @Chestermiller =that was a very good thermodynamics exercise. :)

Edit: For something additional that can be done with this, let $T_{bo}-T_{ao}=t$, and let $\Delta V_a=X$. We have a cubic equation in the form $cX^3+bX^2+aX=t$.
This makes for an iterative solution $X=\frac{t}{a+bX+cX^2}$ with the $X_o=t/a$, etc. We can readily generate something of the form $X=\frac{t}{a}+et^2+ft^3+...$.
(For the series expansion, note that $\frac{t}{a+u}=\frac{t}{a}(1-(u/a)+(u/a)^2+...)$).

 

[Andrew Mason]

The First law requirement
would be as you have it Ches:

$\Delta U_a+\Delta U_b=-\Delta U_{spring}=-\frac{1}{2}k(\Delta V_b/A)^2$
Where $\Delta U_a=n_aC_{V_a}(T_f-T_{a0})$



AM

 

[Andrew Mason]

This looks basically right to me. My approach was a little different. For the energy balance, I had $$n_AC_A(T_f-T_{A,0})+n_BC_B(T_f-T_{B,0})=-\frac{k}{2A^2}(\Delta V)^2$$where $$n_A=\frac{PV_{A,0}}{RT_{A,0}}$$ and $$n_B=\frac{PV_{B,0}}{RT_{B,0}}$$Solving for $T_f$ gives: $$T_f=\frac{n_AC_AT_{A,0}+n_BC_BT_{B.0}}{n_AC_A+n_BC_B}-\frac{k}{2A^2}\frac{(\Delta V)^2}{n_AC_A+n_BC_B}$$This equation applies only when the final state has been reached.

The other equation is $$P_{A,f}=P_{B,f}+\frac{k}{A^2}\Delta V$$or$$RT_f\left(\frac{n_A}{(V_A+\Delta V)}-\frac{n_B}{(V_B-\Delta V)}\right)=\frac{k}{A^2}\Delta V$$If you substitute for $n_a$ and $n_b$, you get:

I was trying to simplify it a bit further:

$T_f=\frac{(\frac{PV_{A,0}}{RT_{A,0}})C_AT_{A,0}+(\frac{PV_{B,0}}{RT_{B,0}})C_BT_{B.0}}{(\frac{PV_{A,0}}{RT_{A,0}})C_A+(\frac{PV_{B,0}}{RT_{B,0}})C_B}-\frac{k}{2A^2}\frac{(\Delta V)^2}{(\frac{PV_{A,0}}{RT_{A,0}})C_A+(\frac{PV_{B,0}}{RT_{B,0}})C_B}$

So the P and R cancels out in the first term:

$T_f=\frac{(V_{A,0})C_A+(V_{B,0})C_B}{(\frac{V_{A,0}}{T_{A,0}})C_A+(\frac{V_{B,0}}{T_{B,0}})C_B}-\frac{k}{2A^2}\frac{(\Delta V)^2}{(\frac{PV_{A,0}}{RT_{A,0}})C_A+(\frac{PV_{B,0}}{RT_{B,0}})C_B}$

If the heat capacities are the same for both gases:

$T_f=\frac{(V_{A,0})+(V_{B,0})}{(\frac{V_{A,0}}{T_{A,0}})+(\frac{V_{B,0}}{T_{B,0}})}-\frac{k}{2A^2}\frac{(\Delta V)^2}{(\frac{PV_{A,0}}{RT_{A,0}})C_v+(\frac{PV_{B,0}}{RT_{B,0}})C_v}$

Not sure that helps much....

AM

 

[Chestermiller]

I was trying to simplify it a bit further:

$T_f=\frac{(\frac{PV_{A,0}}{RT_{A,0}})C_AT_{A,0}+(\frac{PV_{B,0}}{RT_{B,0}})C_BT_{B.0}}{(\frac{PV_{A,0}}{RT_{A,0}})C_A+(\frac{PV_{B,0}}{RT_{B,0}})C_B}-\frac{k}{2A^2}\frac{(\Delta V)^2}{(\frac{PV_{A,0}}{RT_{A,0}})C_A+(\frac{PV_{B,0}}{RT_{B,0}})C_B}$

So the P and R cancels out in the first term:

$T_f=\frac{(V_{A,0})C_A+(V_{B,0})C_B}{(\frac{V_{A,0}}{T_{A,0}})C_A+(\frac{V_{B,0}}{T_{B,0}})C_B}-\frac{k}{2A^2}\frac{(\Delta V)^2}{(\frac{PV_{A,0}}{RT_{A,0}})C_A+(\frac{PV_{B,0}}{RT_{B,0}})C_B}$

If the heat capacities are the same for both gases:

$T_f=\frac{(V_{A,0})+(V_{B,0})}{(\frac{V_{A,0}}{T_{A,0}})+(\frac{V_{B,0}}{T_{B,0}})}-\frac{k}{2A^2}\frac{(\Delta V)^2}{(\frac{PV_{A,0}}{RT_{A,0}})C_v+(\frac{PV_{B,0}}{RT_{B,0}})C_v}$

Not sure that helps much....

AM

Do you want to take a shot at the linearized solution to this based on a small initial temperature difference and a small initial volume difference?

 

[Charles Link]

Just a comment or two for @Andrew Mason : You have two unknowns $T_f$ and $\Delta V$, but you also have two equations, so you should be able to solve for each of them, and not have both unknowns in your final expression(s).

 

[Charles Link]

I would like to give this one a "bump" and comment thank you @Chestermiller =this is one of the better problems we have had on here in the last month or two. I do encourage others to give it a try=it is a fun one. :)