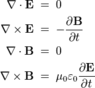- #1
varga
- 64
- 0
I find Maxwell's equations insufficient and superfluous having the Lorentz & Coulomb's force equations. As far as I see magnetic (Lorentz force) and electric (Coulomb's force) interaction is best defined by these two equations themselves, and although Maxwell's equations can describe quite a few electromagnetic interactions, by not having Lorentz force incorporated in any equation nor included as separate one, Maxwell's equations are automatically doomed to fail to describe any interaction due to this very, very important force. Did I miss something?
Why describe electric interaction with any other but with 'Electric force' equation?
Why describe magnetic interaction with any other but with 'Magnetic force' equation?*** Electric interaction
Step 1: Electric field potential given by Coulomb's law
Step 2: Electric force (acceleration vector) given by Coulomb's law
*** Magnetic interaction
Step 3: Magnetic field potential given by Biot-Savart law
Step 4: Magnetic force (acceleration vector) given by Lorentz force
====================================================
As far as I see that's all what is necessary to solve any em interaction, no?
1. Gauss's law
- This is obviously about Coulomb's law/electric potential, so why would this equation be "more suitable"?2. Gauss's law for magnetism
- divB = 0, what in the world? Instead of to describe magnetic potentials or force, to put here Biot-Savart law, or Lorentz force equation or Ampere's force law, they included some equation that has result already calculated in advance? No monopoles? That's as useful as stating "there is no other intelligent life in the Universe". 3. Faraday's law of induction
- Is there anything here we can not calculate with the time integral of four steps given above? How is this induction supposed to accurately describe complete interaction if it is oblivious to Lorentz force?4. Ampère's circuital law
- Is there anything here we can not calculate with the time integral of four steps given above? This is the best candidate to be saying something about Lorentz force, if it only included "Ampere's force law" too.
I suppose the explanation why these equations are in this form is because that is the most suitable for practical application and experimental setups, but still, my greatest concern is how any of that can accurately work without incorporating Lorentz force and Biot-Savart law in the same fashion as Coulomb's law and electric potential/force.
Why describe electric interaction with any other but with 'Electric force' equation?
Why describe magnetic interaction with any other but with 'Magnetic force' equation?*** Electric interaction
Step 1: Electric field potential given by Coulomb's law
Step 2: Electric force (acceleration vector) given by Coulomb's law
*** Magnetic interaction
Step 3: Magnetic field potential given by Biot-Savart law
Step 4: Magnetic force (acceleration vector) given by Lorentz force
====================================================
As far as I see that's all what is necessary to solve any em interaction, no?
1. Gauss's law
- This is obviously about Coulomb's law/electric potential, so why would this equation be "more suitable"?2. Gauss's law for magnetism
- divB = 0, what in the world? Instead of to describe magnetic potentials or force, to put here Biot-Savart law, or Lorentz force equation or Ampere's force law, they included some equation that has result already calculated in advance? No monopoles? That's as useful as stating "there is no other intelligent life in the Universe". 3. Faraday's law of induction
- Is there anything here we can not calculate with the time integral of four steps given above? How is this induction supposed to accurately describe complete interaction if it is oblivious to Lorentz force?4. Ampère's circuital law
- Is there anything here we can not calculate with the time integral of four steps given above? This is the best candidate to be saying something about Lorentz force, if it only included "Ampere's force law" too.
I suppose the explanation why these equations are in this form is because that is the most suitable for practical application and experimental setups, but still, my greatest concern is how any of that can accurately work without incorporating Lorentz force and Biot-Savart law in the same fashion as Coulomb's law and electric potential/force.
Last edited: