- #1
the-brammo
- 6
- 0
The question reads:
A fat wire, radius a, carries a constant current I, uniformly distributed over its cross section. A narrow gap in the wire, of width w << a, forms a parallel-plate capacitor.
I have drawn a red box at the bottom of the page where the Poynting theorem is supposedly verified - however it seems to be a factor of 2 out. I am happy that the derivation for uem is correct, it must be something to do with the very last line. Could someone please point me in the right direction, excuse the pun.
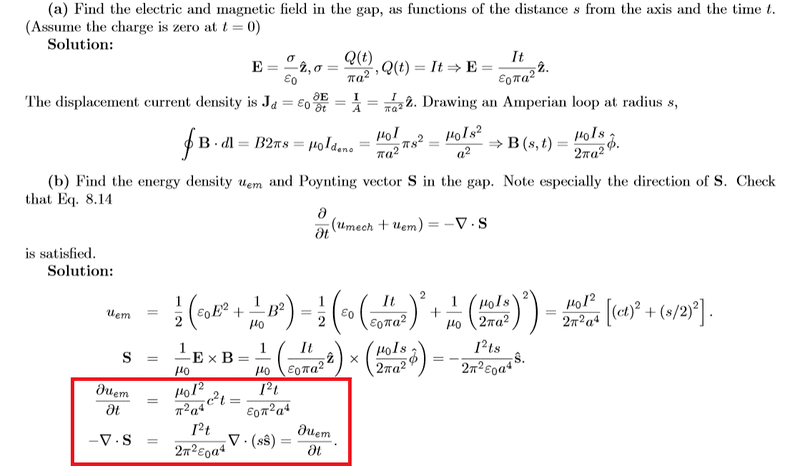
A fat wire, radius a, carries a constant current I, uniformly distributed over its cross section. A narrow gap in the wire, of width w << a, forms a parallel-plate capacitor.
I have drawn a red box at the bottom of the page where the Poynting theorem is supposedly verified - however it seems to be a factor of 2 out. I am happy that the derivation for uem is correct, it must be something to do with the very last line. Could someone please point me in the right direction, excuse the pun.
Last edited: