P=ρ*g*h when h is large (density significantly changes)

[flyboy_1234]

TL;DR Summary

Search for method of how to solve for pressure in a column of vapor when the height is large given the state at the top of the column and the height.

Background:
I am seeking the method of solving the following problem. I’m trying to work through a real-world problem and am stumped on P=ρ *g*h when h is large (density changes significantly)(vapor/gas). I tried to isolate the issue from my bigger problem to help find a formulaic way to solve this. I hope I have formed my question correctly and have not contradicted myself in the process. Below I will show why straight P=ρ *g*h does not work and then explain my approach… for your appraisal.

Problem:

Q: What is the pressure at the bottom of a U-Tube?

In the land of Jack and the Bean Stock, we have a U-Tube that is ridged and well-insulated attached to the bean stock with the top at 1,000 m above the ground (bottom at 0 m). The tops of the U-Tube is connected together with a pump moving 1 kg/minute of CO2 vapor. The pressure at the inlet side is 1 bar (100,000 Pa) and the entropy is 2.5 kJ/kg-K. What is the pressure at the bottom? Consider the system as frictionless. The flow is laminar and steady-state.Diagram 1 - Shows State 1, 2, 3

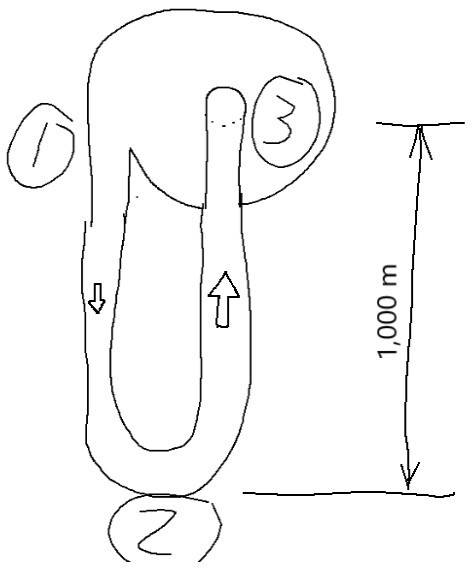
Standard approach (that does not work) of P=ρ*g*h

Substance: CO2
Flow rate: 1 kg/minute
Entropy: 2.5 kJ/kg-K
Height: 1,000 m
P1=1 bar = 1,000 Pa
State 1:
P₁ = 1,000 Pa
s₁=s₂=s₃=2.5 kJ/kg-K

From NIST webbook:
ρ₁=2.4147 kg/m³

State 2:
Solve for P₂

P₂=P₁ + (ρ₁*g*h)
P₂=123,664.06 Pa

From NIST webbook:
ρ₂=2.8349 kg/m3

State 3:
Note: if we use the same method we should get State 2 = State 3 since we are frictionless and insulated given that adiabatic process is reversible

Solve for P₃

P₃=P₂ - (ρ₂*g*h)
P₃=95,882.04 Pa <=== Not equal to P₁

My approach:

Ideally, I would like to start by using the pressure in the middle then find the pressure at the top and bottom by

P₁=P_middle - (ρ_middle*g*(1/2)h)
P₃=P_middle + (ρ_middle*g*(1/2)h)

Since I don't know the middle pressure (or if this is a valid approach) I then have to just go by trial and error until I am close... not exactly an elegant approach.

 

[BvU]

you are searching for the barometric formula, which google

 

[flyboy_1234]

For the record, this is not a student trying to get others to do their homework for them, rather it is just a hic trying to do math beyond his training, one that is not scared to learn whatever it takes to get his head wrapped around it. My problem is easy if I was just dealing with air barometric formula as there are lots of things that are published for atmosphere (air), with very little that is comprehendible for other substances.

I had hoped I would get a more substantial answer that would show you read my attempt at solving the problem and comment if you thought my approach would yield correct results.

 

[Chestermiller]

Why don't you try it first for the case where there is no flow? The equation to use is $$\frac{dp}{dh}=\rho g$$ where the density $\rho$ is calculated from the ideal gas law, such the $$\rho=\frac{pM}{RT}$$with M being the molecular weight of the CO2, R being the universal gas constant, and T being the absolute temperature.

 

[flyboy_1234]

Thanks for the reply Chestermiller. I thought I had started with your formula $\frac{dp}{dh}=\rho g$ but ran into difficulties. I tried to formulate this problem using a U-Tube to illustrate my confusion. (It is very likely I am just confused in my lack of understanding, and the rest of you can't see what I am getting stuck on.)

State 1 (top of U-Tube)
I know Pressure $P_1=1 [bar]=100,000[Pa]$ and Entropy $s=2.5 [kJ/kg-K]$ and am able to look up from a table $\rho_1=2.4147 [kg/m3]$

Using your formula:
$$\begin{align*} \frac{\Delta p}{\Delta h}&=\rho g \\
\Delta p &= \rho g \Delta h \\
\Delta p &=2.4147 [\frac{kg}{m^3}]*9.8[\frac{m}{s^2}]*1000[m] \\
\Delta p &=23,664 [Pa] \\
\\
P_2 &= P_1 + \Delta p \\
P_2 &= 100,000 [Pa] + 23,664 [Pa] \\
P_2 &= 123,664 [Pa] \\ \end{align*}
$$
Now that I have the pressure at the bottom of the U-Tube I should be able to reverse the process and get $P_3$. Since the process was adiabatic (reversable) it should equal $P_1$.

Since the pressure at final state does not equal the pressure at the initial state (see original post) I am at a loss how to proceed.

 

[Chestermiller]

Thanks for the reply Chestermiller. I thought I had started with your formula $\frac{dp}{dh}=\rho g$ but ran into difficulties. I tried to formulate this problem using a U-Tube to illustrate my confusion. (It is very likely I am just confused in my lack of understanding, and the rest of you can't see what I am getting stuck on.)

State 1 (top of U-Tube)
I know Pressure $P_1=1 [bar]=100,000[Pa]$ and Entropy $s=2.5 [kJ/kg-K]$ and am able to look up from a table $\rho_1=2.4147 [kg/m3]$

Using your formula:
$$\begin{align*} \frac{\Delta p}{\Delta h}&=\rho g \\
\Delta p &= \rho g \Delta h \\
\Delta p &=2.4147 [\frac{kg}{m^3}]*9.8[\frac{m}{s^2}]*1000[m] \\
\Delta p &=23,664 [Pa] \\
\\
P_2 &= P_1 + \Delta p \\
P_2 &= 100,000 [Pa] + 23,664 [Pa] \\
P_2 &= 123,664 [Pa] \\ \end{align*}
$$
Now that I have the pressure at the bottom of the U-Tube I should be able to reverse the process and get $P_3$. Since the process was adiabatic (reversable) it should equal $P_1$.

Since the pressure at final state does not equal the pressure at the initial state (see original post) I am at a loss how to proceed.

The equation for the density of CO2, treated as an ideal gas is: $$\rho=\frac{pM}{RT}$$At a pressure of 1 bar and a temperature of 298 K, this yields a value of 1.776 kg/m^3 for CO2 (rather than 2.4147).

My two formulas reduce to $$\frac{dp}{dh}=\frac{pM}{RT}g$$ or, equivalently $$\frac{dp}{dh}=1.776g\frac{p}{p_0}$$where $p_0=100000\ Pa$ and p is the pressure at depth h. If I integrate this equation from 0 to h=1000 m, I get $$p=p_0\exp{\left(\frac{1.776gh}{p_0}\right)}=100000\exp{\left(\frac{17400}{100000}\right)}=119000\ Pa$$

 

[jack action]

Shouldn't we assume an isentropic process?
$$ \begin{align*}
dp &=\rho gdh \\
dp &= \frac{pM}{RT}gdh \\
dp &= \frac{pM}{RT_0\left(\frac{p}{p_0}\right)^{\frac{\lambda - 1}{\lambda}}}gdh \\
dp &= \frac{p^\frac{1}{\lambda}Mp_0^\frac{\lambda-1}{\lambda}}{RT_0}gdh \\
\int\frac{dp}{p^\frac{1}{\lambda}} &= \int\frac{gMp_0^\frac{\lambda-1}{\lambda}}{RT_0}dh \\
\frac{\lambda}{\lambda - 1}\left(p^\frac{\lambda-1}{\lambda} - p_0^\frac{\lambda-1}{\lambda}\right) &= \frac{gMp_0^\frac{\lambda-1}{\lambda}}{RT_0}(h_0 - h) \\
\\
\\
p &= \sqrt[\frac{\lambda-1}{\lambda}]{\frac{\lambda - 1}{\lambda}\frac{gMp_0^\frac{\lambda-1}{\lambda}}{RT_0}(h_0 - h) + p_0^\frac{\lambda-1}{\lambda}}
\end{align*}$$

 

[Chestermiller]

Shouldn't we assume an isentropic process?
$$ \begin{align*}
dp &=\rho gdh \\
dp &= \frac{pM}{RT}gdh \\
dp &= \frac{pM}{RT_0\left(\frac{p}{p_0}\right)^{\frac{\lambda - 1}{\lambda}}}gdh \\
dp &= \frac{p^\frac{1}{\lambda}Mp_0^\frac{\lambda-1}{\lambda}}{RT_0}gdh \\
\int\frac{dp}{p^\frac{1}{\lambda}} &= \int\frac{gMp_0^\frac{\lambda-1}{\lambda}}{RT_0}dh \\
\frac{\lambda}{\lambda - 1}\left(p^\frac{\lambda-1}{\lambda} - p_0^\frac{\lambda-1}{\lambda}\right) &= \frac{gMp_0^\frac{\lambda-1}{\lambda}}{RT_0}(h_0 - h) \\
\\
\\
p &= \sqrt[\frac{\lambda-1}{\lambda}]{\frac{\lambda - 1}{\lambda}\frac{gMp_0^\frac{\lambda-1}{\lambda}}{RT_0}(h_0 - h) + p_0^\frac{\lambda-1}{\lambda}}
\end{align*}$$

If there is no flow, why would we assume an isentropic process? Even if there was flow, it would depend on the flow rate and heat transfer characteristics of the system.

 

[flyboy_1234]

I tried to set up the problem with enough flow in a steady state to force it to be an isentropic process with ample insulation and ridged.

 

[vanhees71]

If there is no flow, why would we assume an isentropic process? Even if there was flow, it would depend on the flow rate and heat transfer characteristics of the system.

It's experimentally easy to demonstrate that you have an adiabatic process with good accuracy by simply measuring the sound velocity in air. You get the adiabatic not the isothermal value.

 

[Chestermiller]

It's experimentally easy to demonstrate that you have an adiabatic process with good accuracy by simply measuring the sound velocity in air. You get the adiabatic not the isothermal value.

If there is no flow in the tube, the system will eventually be isothermal.

 

[Chestermiller]

I tried to set up the problem with enough flow in a steady state to force it to be an isentropic process with ample insulation and ridged.

Then the thing to do is to use @jack action's results to get the downhole pressure. But, understand that you are also assuming that there is negligible viscous friction affecting the pressure variation. If there were viscous friction, the pressure variation would be different, and the assumption of isentropic flow would also be invalid. Also, you would have to employ a significant pressure difference between the inlet and outlet of the U-tube.

So, try the calculation over again with jack action's equation. It should also be possible to use his equation to show that the pressure variation in the up-leg of the U-tube is such that, at the top, the pressure is again 1 bar.

 

[Chestermiller]

Shouldn't we assume an isentropic process?
$$ \begin{align*}
dp &=\rho gdh \\
dp &= \frac{pM}{RT}gdh \\
dp &= \frac{pM}{RT_0\left(\frac{p}{p_0}\right)^{\frac{\lambda - 1}{\lambda}}}gdh \\
dp &= \frac{p^\frac{1}{\lambda}Mp_0^\frac{\lambda-1}{\lambda}}{RT_0}gdh \\
\int\frac{dp}{p^\frac{1}{\lambda}} &= \int\frac{gMp_0^\frac{\lambda-1}{\lambda}}{RT_0}dh \\
\frac{\lambda}{\lambda - 1}\left(p^\frac{\lambda-1}{\lambda} - p_0^\frac{\lambda-1}{\lambda}\right) &= \frac{gMp_0^\frac{\lambda-1}{\lambda}}{RT_0}(h_0 - h) \\
\\
\\
p &= \sqrt[\frac{\lambda-1}{\lambda}]{\frac{\lambda - 1}{\lambda}\frac{gMp_0^\frac{\lambda-1}{\lambda}}{RT_0}(h_0 - h) + p_0^\frac{\lambda-1}{\lambda}}
\end{align*}$$

Simplifying

$$p = p_0\left(\frac{\lambda - 1}{\lambda}\frac{gM}{RT_0}(h_0 - h) + 1\right)^{\lambda/(\lambda-1)}$$

 

[vanhees71]

Sure, I thought you are discussing the barometric formula, and there one usually assumes adiabacy or uses a phenomenological polytropic equation of state,
$$p=p_0 \left (\frac{\rho}{\rho_0} \right)^n.$$
(according to Sommerfeld using $n=1.2$ rather than $n=1.4$, which latter is the adiabatic case for gas molecules, which is to good approximation the case for air). For $n=1$ you get the isothermal equation of state (all for an ideal gas).

Here the barometric formula is easy to derive. It follows from
$$\vec{\nabla} p=\rho \vec{g}=C p^(1/n) \vec{g}.$$
For $\vec{g}=-g \vec{e}_3$ you get $p=p(x_3)$ and thus
$$p'=-C g p^{1/n}$$
with the solution
$$p(x_3)=p_0 \left (1-\frac{n-1}{n} \frac{p_0}{\rho_0} g x_3 \right)^{n/(n-1)}.$$
For $n \rightarrow 1$ it follows
$$p(x_3)=p_0 \exp \left (-\frac{\rho_0}{p_0} g x_3 \right),$$
and since for an ideal gas
$$p=\frac{\rho R T}{\mu} \; \Rightarrow \; \frac{\rho}{p}=\frac{\rho_0}{p_0} = \frac{\mu}{R T}$$
you the barometric formula
$$p=p_0 \exp \left (-\frac{\mu g x_3}{R T} \right).$$

 

[Chestermiller]

Sure, I thought you are discussing the barometric formula, and there one usually assumes adiabacy or uses a phenomenological polytropic equation of state,
$$p=p_0 \left (\frac{\rho}{\rho_0} \right)^n.$$
(according to Sommerfeld using $n=1.2$ rather than $n=1.4$, which latter is the adiabatic case for gas molecules, which is to good approximation the case for air). For $n=1$ you get the isothermal equation of state (all for an ideal gas).

Here the barometric formula is easy to derive. It follows from
$$\vec{\nabla} p=\rho \vec{g}=C p^(1/n) \vec{g}.$$
For $\vec{g}=-g \vec{e}_3$ you get $p=p(x_3)$ and thus
$$p'=-C g p^{1/n}$$
with the solution
$$p(x_3)=p_0 \left (1-\frac{n-1}{n} \frac{p_0}{\rho_0} g x_3 \right)^{n/(n-1)}.$$
For $n \rightarrow 1$ it follows
$$p(x_3)=p_0 \exp \left (-\frac{\rho_0}{p_0} g x_3 \right),$$
and since for an ideal gas
$$p=\frac{\rho R T}{\mu} \; \Rightarrow \; \frac{\rho}{p}=\frac{\rho_0}{p_0} = \frac{\mu}{R T}$$
you the barometric formula
$$p=p_0 \exp \left (-\frac{\mu g x_3}{R T} \right).$$

No. This is a U-tube where, with no flow, parcels of gas can't move upward and downward by circulations like in the atmosphere.

 

[vanhees71]

Hm, but the above calculation still applies. The only question is, whether you have an adiabatic, isothermal, or more general situation. I'm not too sure about that question here. Anyway, you find the solution for any polytropic EoS above ;-)).

 

[Chestermiller]

Using the simplified form of @jack action's formula I presented in post #13, at a depth of 1000 M, using $\lambda = 1.28$ for CO2, we get:
$$p=100000\left(1+\frac{0.28}{1.28}\frac{174000}{100000}\right)^{\frac{1.28}{0.28}}=118620\ Pa$$

 

[vanhees71]

Just to clarify: After all you use a polytropic equation of state (my $n$ is obviously your $\lambda$). Since you use $\lambda=c_p/c_v$ for $\text{CO}_2$ it's the adiabatic equation of state after all. So the assumption is that in the considered static equilibrium no heat-exchange occurs between the "layers" of the gas.

 

[Chestermiller]

Just to clarify: After all you use a polytropic equation of state (my $n$ is obviously your $\lambda$). Since you use $\lambda=c_p/c_v$ for $\text{CO}_2$ it's the adiabatic equation of state after all. So the assumption is that in the considered static equilibrium no heat-exchange occurs between the "layers" of the gas.

Like I said, from a fluid dynamics and heat transfer perspective, if the gas flow rate is high enough for heat transfer between the medium surrounding the U-tube and the flowing gas is negligible, yet low enough or inviscid enough for viscous frictional drag between the walls of the U-tube and the flowing gas to be negligible, the flow can be regarded as isentropic. However, if the gas is not flowing (i.e., static), there will be sufficient heat transfer between the medium surrounding the U-tube and the gas for the gas to come to equilibrium with the medium, and the gas will be isothermal.

 

[vanhees71]

But then why are you giving the solution with the adiabatic equation of state as solution? Isn't this a contradiction?

 

[Chestermiller]

But then why are you giving the solution with the adiabatic equation of state as solution? Isn't this a contradiction?

To simplify things to begin with, I originally suggested the the OP first consider the isothermal case without flow. Why? If he couldn’t solve that case, he probably wouldn’t be able to analyze the case with flow. If you go back through the history of the thread, you will see that, in the beginning, he didn’t even realize how to include the density variation with pressure.

 

[vanhees71]

It's of course also clear that for ideal hydrodynamics, which is nothing else than the local-equilibrium limit of the Boltzmann transport equation, the right equation of state must be the adiabatic one.

It should be clear to every student of hydrodynamics that in addition to the local conservation laws, which are in non-relativistic hydro the conservation of mass (continuity equation), momentum (equivalent to Euler's equation of motion), you always need an equation of state, relating pressure and (mass) density. That's easy to count: You have 3 components for the flow field, $\vec{v}(t,\vec{x})$ and pressure, $P$, and (mass) density $\rho$ to solve for, and thus you need 5 equations. So in addition to the above mentioned field equations of motion you also need an equation of state like $P=P(\rho)$.

In relativistic hydrodynamics it's the same. The only difference is that instead of the mass-conservation law you have energy conservation, i.e., the local conservation law for the energy-momentum tensor $\partial_{\mu} T^{\mu \nu}=0$. If there are conserved charges (like baryon number, strangeness, electric charge) of the particles making up the flow you have in addition a continuity equation for the corresponding current $\partial_{\mu} j^{\mu}=0$. Also here you need an equation of state.

In my field of research, relativistic nuclear physics (heavy-ion collisions, neutron stars), the question about the equation of state is among the most interesting ones. Here the problem is that we don't now, how the equation of state really looks like, and one has to draw conclusions from observations (like the investigation of the momentum spectra of produced particles in heavy-ion collisions, the mass-radius relation for neutron stars, or the gravitational-wave-signal shape in double-neutron-star mergers, as available from LIGO/VIRGO).