- #1
Calabi_Yau
- 35
- 1
Homework Statement
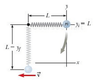
A system consists of a spring with force constant k = 1250 N/m, length L = 1.50m, and an object of mass m = 5.00kg attached to the end. The object is placed at the level of the point of attachment with the spring unstretched, at position yi= L, an then is released so that it swings like a pendulum.
a) Find the y position of the object at the lowest point.
b) Will the pendulum's period be greater or less than the period of a simple pendulum with same mass m and length L?
The Attempt at a Solution
b) As the spring will stretch out during the swing, its period will be greater than that of a simple pendulum, as the greater the l, the greater the period.
a) This is where I'm having trouble. I imagine the spring going down and stretching out to the max at the lowest point, and the shrinking again in the ascent.
I used an xy axis, with y = L being the plane of the mass before moving. The - Y would be the y coordinate at the lowest point.
I am not even sure if that is correct, but I took it and went on to tackle the problem with an energy approach.
Its maximum potential energy was at the top. U = mgL.
At the lowest point, Elastic potential energy is at its maximum Uelastic = 1/2 * k( Y)^2. And so is kinetic energy. This is where I get stuck. I don't now what to use for velocity. Because it has tangential and normal direction and has the length of the spring is not constant I don't know what to take into account for the normal direction.
This all should work if and only if my first assumptions were right, that the maximum length happens at the lowest point. But I'm not even sure about it.
Any help would be appreciated :)