- #1
tracker890 Source h
- 90
- 11
- Homework Statement
- Determine flow rate per unite width in the source flow
- Relevant Equations
- flow rate equation
Please help me to understand what wrong with method 2.
ref.Flowrate Between Streamlines
(Thank you for your time and consideration.)
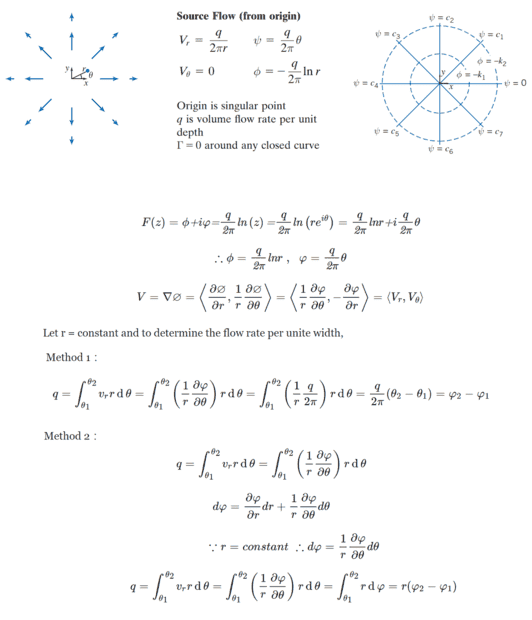
ref.Flowrate Between Streamlines
(Thank you for your time and consideration.)
Last edited: