[Semiclassical physics] 1D box trace formula

[carlosbgois]

Hey there. While studying the Single-particle Level Density, I encountered the example in the image below, referring to the One-dimensional Box problem. However, I do not understand what is it that he call's F(E), neither how does one go from that, to the density of states in Equation (3.64).

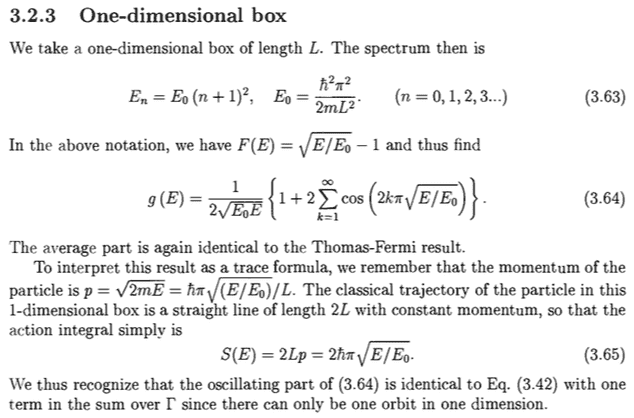
http://imgur.com/cBRbFXz

Can somebody hint me on how to reproduce this result?
Thank you for your time.

 

[gtoguy97]

F(E) is the total number of energy levels in the one-dimensional box problem that have an energy less than or equal to E. To calculate it, you can use the fact that the total number of energy levels for a one-dimensional box problem is equal to its quantum number n^2. So, you can calculate F(E) by summing up the energy levels with energies below E for all values of n from 1 to sqrt(E).Once you have calculated F(E), you can use it to calculate the density of states (DOS). This is because the DOS is simply the derivative of F(E) with respect to E. So, if you take the derivative of F(E) with respect to E, you will get the DOS in Equation (3.64).