How can incorrect torque calculations lead to contradictory solutions?

[smr101]

Hi,

Struggling with calculating the angle of twist, using TL/GJ.

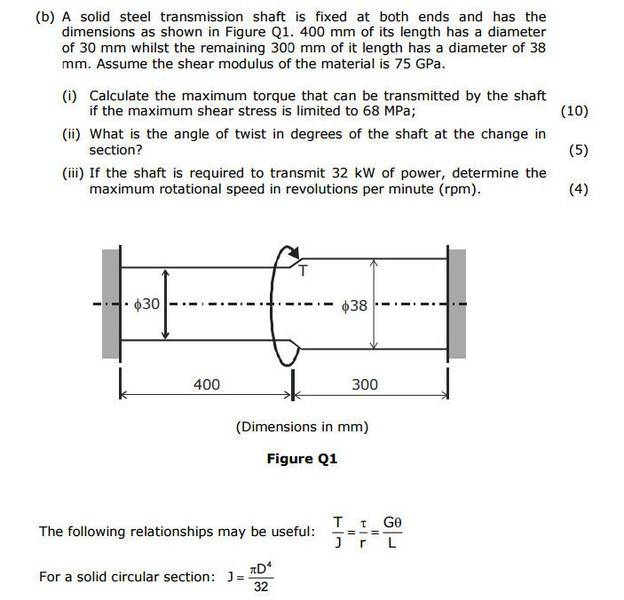
The answer to (b)(i) is 946.1 Nm.

Using 0.3 m length section.

946.1 * 0.3 / 75 x 10^9 * 2.047 x 10^-7

= 0.0185 * 180/pi

= 1.06 rads

Answer is 0.273.

Question below (b)(ii):

 

[billy_joule]

Show your working so we can see where you went wrong.

 

[smr101]

Just realized I was treating it as a series shaft and not parallel as it is.

 

[smr101]

Just realized I was treating it as a series shaft and not parallel as it is.

Have edited OP, (b)(ii) now not making sense, thanks.

 

[billy_joule]

I've never heard of the terms series and parallel applied to a shaft but I would call each portion of the shaft in series with each other.

Which shaft section (long and thin or short and thick) will twist more under torque? What is the unit of angles in the equation you used? Ie was the conversion necessary?

 

[smr101]

I've never heard of the terms series and parallel applied to a shaft but I would call each portion of the shaft in series with each other.

Which shaft section (long and thin or short and thick) will twist more under torque? What is the unit of angles in the equation you used? Ie was the conversion necessary?

In parallel both ends are rigidly connected, in series only one is and torque is applied to the other end, so in this case it is parallel. In parallel total torque = T1 + T2 and angle of twist is equal for each section, so they will both have the same angle of twist.

Yes the conversion was necessary, the answer is converted from rads to degrees.

Still not getting the correct answer though!

 

[billy_joule]

Oh i see, I didn't notice the torque is applied in the centre.
You need to consider both sections simultaneously and with the appropriate constraints, in your attempt you found the twist if the thin section didn't exist.
Note that each section won't support the same torque load.

 

[smr101]

Oh i see, I didn't notice the torque is applied in the centre.
You need to consider both sections simultaneously and with the appropriate constraints, in your attempt you found the twist if the thin section didn't exist.
Note that each section won't support the same torque load.

Ok, so I should use the total length?

I am under the impression the angle of twist of one section is the same as the other, so why do they need to both be taken into account? Should one sections not be enough?

 

[billy_joule]

How did you solve the first question? The approach should be similar to that.

Ok, so I should use the total length?

I am under the impression the angle of twist of one section is the same as the other, so why do they need to both be taken into account? Should one sections not be enough?

Imagine a weight hanging from two different ropes in parallel, the max weight is more than either of the two ropes can take alone. But how much more? All we know is both ropes must be stretched the same distance when one (and only one) breaks. To find the max stretch (or breaking load) you need to consider both ropes together, they share the load but not equally.
That's analogous to your shaft. You'll need to form an equation for each section of the shaft and solve them simultaneously.

 

[smr101]

How did you solve the first question? The approach should be similar to that.Imagine a weight hanging from two different ropes in parallel, the max weight is more than either of the two ropes can take alone. But how much more? All we know is both ropes must be stretched the same distance when one (and only one) breaks. To find the max stretch (or breaking load) you need to consider both ropes together, they share the load but not equally.
That's analogous to your shaft. You'll need to form an equation for each section of the shaft and solve them simultaneously.

Have you done this question and got the correct solution yourself?

I've individually got the angle of twist for each using TL/JG.

The 400 mm section = 0.0242 rads using T = 360.5 Nm and 300 mm section = 0.01431 rads. Not sure where to go from here.

Worked out T and J individually for each part using the equations given in the question.

 

[billy_joule]

Have you done this question and got the correct solution yourself?

No, not yet. I'm hoping it won't need to come to that

I've individually got the angle of twist for each using TL/JG.

The 400 mm section = 0.0242 rads using T = 360.5 Nm and 300 mm section = 0.01431 rads. Not sure where to go from here.

Worked out T and J individually for each part using the equations given in the question.

That doesn't make sense. In post #6, you already correctly recognised that the angle of twist is the same for each section, and now you're saying they're different?

Lets go back to what you said in #6, we know:
θ_L = θ_R =θ (let L be the left section and R be the right)

And we know:

θ_L = T_LL_L / GJ_L

θ_R = T_RL_R / GJ_R

and so:
θ = T_LL_L / GJ_L = T_RL_R / GJ_R

If you've done Q i) correctly you know all the variables* except θ so just plug and chug.

*You may not know T_R and T_L individually but you do know their sum as you mentioned in #6.

 

[smr101]

No, not yet. I'm hoping it won't need to come to that

That doesn't make sense. In post #6, you already correctly recognised that the angle of twist is the same for each section, and now you're saying they're different?

Lets go back to what you said in #6, we know:
θ_L = θ_R =θ (let L be the left section and R be the right)

And we know:

θ_L = T_LL_L / GJ_L

θ_R = T_RL_R / GJ_R

and so:
θ = T_LL_L / GJ_L = T_RL_R / GJ_R

If you've done Q i) correctly you know all the variables* except θ so just plug and chug.

*You may not know T_R and T_L individually but you do know their sum as you mentioned in #6.

So without the individual torques how do I incorporate the sum into the equation?

 

[billy_joule]

That's why I asked this:

How did you solve the first question?

Once you've solve Q i) it should be trivial to find the torque in each section.

 

[smr101]

That's why I asked this:

Once you've solve Q i) it should be trivial to find the torque in each section.

I do have the torque for each section, yes.

Still not getting the correct solution though. I'll take you through what I've done:

I have JL = 7.952 x 10^-8 and JR = 2.047 x 10^-7.

I have TR = shear stress * JR/ radius of right hand side = 732.6 Nm

I then calculated TL = (LR*JL / LL*JR) * TR
TL = 213.4 Nm

TL + TR = 946 Nm which is the correct solution for (i). So I know all number up to this point are correct as they fit with the solution for part (i).

Those are all the figures needed for the angle of twist plus the given value G = 75GPa and LL = 0.4 m and LR = 0.3 m.

Using those figures I'm not getting the correct solution.

 

[billy_joule]

I do have the torque for each section, yes.

Still not getting the correct solution though. I'll take you through what I've done:

I have JL = 7.952 x 10^-8 and JR = 2.047 x 10^-7.

I have TR = shear stress * JR/ radius of right hand side = 732.6 Nm

I then calculated TL = (LR*JL / LL*JR) * TR
TL = 213.4 Nm

TL + TR = 946 Nm which is the correct solution for (i). So I know all number up to this point are correct as they fit with the solution for part (i).

The given solution is wrong. You've found the max torque for each shaft section in isolation without considering the constraint that they must both twist the same angle. It would be a rare coincidence if both shaft sections happened to reach their max torque at the same twist angle. This is what I was trying to illustrate with my parallel rope analogy-one shaft will break before the other (except for two special cases: a certain ratio for L & r for each shaft and of course, identical shafts)

You can show the first answer is wrong by calculating the torque required to reach the twist angle in the 2nd given answer, you will find they do not match the torque value in the previous answer - the answers are contradictory.