Tricky problem: Falling plank originally leaning against a wall

[Order]

Homework Statement

A plank of lengt 2l leans against a wall. It starts to slip downward without friction. Show that the top of the plank loses contact with the wall when it is at two-thirds of its initial height.
Hint: Only a single variable is needed to describe the system. Note the motion of the center of mass.

Homework Equations

\tau=I\alpha
Energy conservation

The Attempt at a Solution

I don't get very far at all.

First of all I evaluate the torque of the system: \tau=lMg\sin \theta=I\ddot{\theta}=\frac{Ml^{2}}{3}\ddot{\theta}, which leads to \ddot{\theta}=\frac{3g}{l}\sin \theta. This is not possible to solve exactly and therefore is of no help.

Next, I try energy conservation to evaluate the angular velocity at a height of two-thirds of the original: Mgl\cos\theta=\frac{1}{2}\frac{Ml^{2}}{3}\omega^{2}+\frac{2}{3}Mgl\cos\theta, which leads to the result \omega^{2}=\frac{2g}{l}\cos\theta. Now this is of no use either since it cannot be combined with the first equation. It is a relation relative to the original angle, whereas the first equation above is a general differential equation.

Now if neither torque evalutations nor energy conservation leads anywhere, I don't know how to go on. Does anyone have a hint?

 

[SammyS]

Draw a free body diagram.

You are finding the torque about which point?

 

[Doc Al]

It's a tricky problem. Use the hint: What path does the CM follow as the plank falls?

 

[Order]

Draw a free body diagram.

You are finding the torque about which point?

Here is a FBD as I think it should be. In the diagram I also think that the normal forces are N_{1}=Mg\sin\theta and N_{2}=Mg\cos\theta where the latter might be most interesting. See my post below for further thoughts.

 

[Doc Al]

In the diagram I also think that the normal forces are N_{1}=Mg\sin\theta and N_{2}=Mg\cos\theta

How did you determine this?

 

[Order]

It's a tricky problem. Use the hint: What path does the CM follow as the plank falls?

Sure, I can try that. First of all I can evaluate the center of mass in the x direction. x_{cm}=l\cos\theta and differentiating this gives \frac{dx_{cm}}{dt}=-l\sin\theta\omega Now since theta is evaluated in the positive direction, whereas I am interested in the negative direction, I revise this to \frac{dx_{cm}}{dt}=l\sin\theta\omega Differntiating once again yields \frac{dx^{2}_{cm}}{dt^{2}}=l\cos\theta\omega^{2}+l\sin\theta\alpha Now putting in relations for alpha and omega in my original post, slightly changed, I get \frac{dx^{2}_{cm}}{dt^{2}}=6g\cos\theta(\cos\theta-cos\phi)-3g\sin^{2}\theta, where phi is the starting angle.

At first I thought Í could find where the acceleration is zero to find where the plank loses contact, but I find that the acceleration is always positive. Instead I might use the results from my free body diagram in post above to solve the equation M\frac{dx^{2}_{cm}}{dt^{2}}=N_{1}=Mg\cos\theta which leads to the equation 6\cos\theta(\cos\theta-\cos\phi)-3\sin^{2}\theta=\cos\theta to get a relation between theta and phi. Unfortunately it seems like a very difficult equation to solve.

I can do the same thing with the center of mass in the y direction. Now the result is y_{cm}=l\sin\theta \frac{dy_{cm}}{dt}=l\cos\theta\omega \frac{dy^{2}_{cm}}{dt^{2}}=l\sin\theta\omega^{2}-l\cos\theta\alpha But unless my equation I found above is correct, I am stuck once again.

 

[Doc Al]

Describe in words the path of the CM. It's surprisingly simple. (The path, not the problem.)

 

[Order]

How did you determine this?

On closer thought I need to revise this to N_{2}=\frac{\sin\theta\cos\theta}{2} where the trignonometric part is due to the force being applied perpendicular to the gravity force through the plank. The factor 2 is due to the normal force being at twice the length. I think this should be correct.

 

[Order]

Describe in words the path of the CM. It's surprisingly simple. (The path, not the problem.)

I don't know how this helps. Will think about it. But as for know I can readily see that it is a circle.

 

[Doc Al]

But as for know I can readily see that it is a circle.

Excellent! Now apply conservation of energy to find the velocity of the CM as a function of angle.

 

[Order]

Excellent! Now apply conservation of energy to find the velocity of the CM as a function of angle.

I still have no clue why this is good. I try to think it through and although it seems like something good I can't see the end of the string.

I can express it as a function of a fraction of the original height, or, as I guess you wanted me too, express it as a difference of the original and the final angle: v^{2}_{CM}=2lg(\sin\phi-\sin\theta) or if a is a fraction of the original height v^{2}_{CM}=2lg\sin\phi(1-a)

 

[Doc Al]

There's only one angle here: The angle the plank makes with the wall. (Assume it starts out perfectly flat against the wall, and is then given a nudge.)

To apply conservation of energy, you must include both rotational and translational KE terms.

 

[Order]

There's only one angle here: The angle the plank makes with the wall. (Assume it starts out perfectly flat against the wall, and is then given a nudge.)

To apply conservation of energy, you must include both rotational and translational KE terms.

Ooops, sorry about that. In that case i san write v_{cm}^{2}=2gl(1-\sin\theta)-\frac{1}{3}l^{2}\omega^{2} or (\omega l)^{2}=\frac{3}{2}gl(1-\sin\theta)

By the way, I'm off tomorrow, so maybe I need to wait a week to solve this problem if I don't today.

 

[Doc Al]

Good. Hint: Consider the horizontal component of this velocity.

 

[Order]

Good. Hint: Consider the horizontal component of this velocity.

Ok, now I'm back from my little vacation. I still don't know how velocities can help, though. I want to deal with accelerations. So I need to differentiate the equation above.

Firstly I separate the horizontal and vertical velocities.

\omega^{2}(\sin^{2}\theta+\cos^{2}\theta)=\frac{3}{2}gl(1-\cos\theta) I can then differntiate this to -2\omega\sin\theta a_{x_{CM}}l+2\omega\cos\theta a_{y_{CM}}l=-\frac{3}{2}gl\cos\theta\omega Continuing this way, first dividing by omega, then substituting for a_xcm=N₂ (where it loses contact), omega² (angular velocity) and alpha (angular acceleration), I get the equation \sin^{2}\theta+2\sin\theta\cos\theta-\frac{3}{2}=0 This leads to the result that the plank loses contact at about 5% of its initial height (analyzing the equation numerically).

Am I on the right track or am I supposed to do something completely different?

 

[Doc Al]

I still don't know how velocities can help, though. I want to deal with accelerations. So I need to differentiate the equation above.

Yes, you'll need to differentiate.

Start by giving an expression for the horizontal component of the velocity. Then differentiate that.

 

[Order]

Yes, you'll need to differentiate.

Start by giving an expression for the horizontal component of the velocity. Then differentiate that.

Yes, I needed the free body diagram to reevaluate the angular acceleration, and using that the horizontal acceleration should be zero I arrived at the equation -2\sin\theta+2\sin^{2}\theta-\cos^{2}\theta=-1 with the non-trivial solution \sin\theta=\frac{2}{3} Thanks for help Doc!

 

[Doc Al]

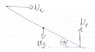
Excellent! (A tricky problem indeed.)