- #1
Happiness
- 679
- 30
How do we get (5.381)?
The term involving ##V## in (5.378) is ##V(r' + vt, t)\ \Psi(r' + vt, t)##. After dividing on both sides of (5.378) by the exponential term ##e^{[i(mv.r' + mv^2t/2)/\hbar]}## [which appears in (5.379)], the term becomes ##V(r' + vt, t)\ \Psi(r', t)##. But the term as given in (5.380) is ##V'(r', t)\ \Psi(r', t)##. This means that we must have ##V'(r', t) = V(r' + vt, t) = V(r, t)##, which contradicts (5.381).
I've worked out the other terms and they are correct.
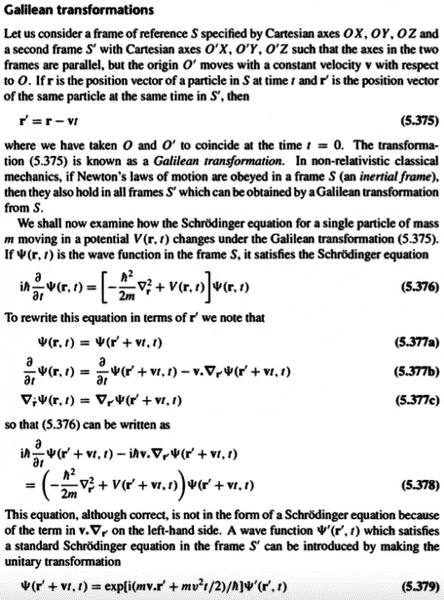
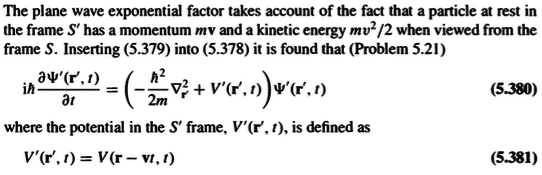
The term involving ##V## in (5.378) is ##V(r' + vt, t)\ \Psi(r' + vt, t)##. After dividing on both sides of (5.378) by the exponential term ##e^{[i(mv.r' + mv^2t/2)/\hbar]}## [which appears in (5.379)], the term becomes ##V(r' + vt, t)\ \Psi(r', t)##. But the term as given in (5.380) is ##V'(r', t)\ \Psi(r', t)##. This means that we must have ##V'(r', t) = V(r' + vt, t) = V(r, t)##, which contradicts (5.381).
I've worked out the other terms and they are correct.