Why is (5.302) an Approximation of Exponential Operators?

[Happiness]

Why is (5.302) an approximation instead of an equality?

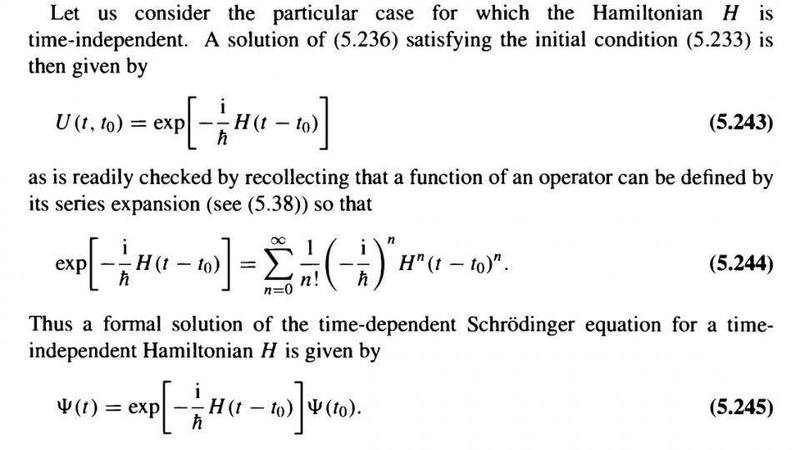
Let $T$ be the operator $\frac{p_x^2}{2m}$.

By the law of indices, we should have $e^{-\frac{i}{\hbar}(T+V)\Delta t}=e^{-\frac{i}{\hbar}T\Delta t} e^{-\frac{i}{\hbar}V\Delta t}$ exactly. Is it because $T$ and $V$ do not commute? So the correct equation should be $e^{-\frac{i}{\hbar}(T+V)\Delta t}=\frac{1}{2}[e^{-\frac{i}{\hbar}T\Delta t} e^{-\frac{i}{\hbar}V\Delta t}+e^{-\frac{i}{\hbar}V\Delta t} e^{-\frac{i}{\hbar}T\Delta t}]$?

But if this is so, shouldn't (5.302) be correct up to terms of order $\Delta t$ instead of order $(\Delta t)^2$ as claimed by the book?

 

[atyy]

Use the definition of the exponential operator as a series. https://en.wikipedia.org/wiki/Matrix_exponential

 

[strangerep]

[...] Is it because $T$ and $V$ do not commute?

Yes.

So the correct equation should be $e^{-\frac{i}{\hbar}(T+V)\Delta t}=\frac{1}{2}[e^{-\frac{i}{\hbar}T\Delta t} e^{-\frac{i}{\hbar}V\Delta t}+e^{-\frac{i}{\hbar}V\Delta t} e^{-\frac{i}{\hbar}T\Delta t}]$?

Not in general. Check out the Baker-Campbell-Hausdorff formula(s).

 

[Happiness]

When I work out the term of order $(\Delta t)^2$, the left hand side gives $\frac{1}{2}(-\frac{i}{\hbar})^2(T^2+TV+VT+V^2)(\Delta t)^2$ but the right hand side gives $\frac{1}{2}(-\frac{i}{\hbar})^2(T^2+2TV+V^2)(\Delta t)^2$. They are not equal. So is the book wrong?

 

[strangerep]

Which book is it?

 

[Happiness]

Which book is it?

It's Quantum Mechanics 2nd ed. by Bransden & Joachain, page 243.

 

[samalkhaiat]

When I work out the term of order $(\Delta t)^2$, the left hand side gives $\frac{1}{2}(-\frac{i}{\hbar})^2(T^2+TV+VT+V^2)(\Delta t)^2$ but the right hand side gives $\frac{1}{2}(-\frac{i}{\hbar})^2(T^2+2TV+V^2)(\Delta t)^2$. They are not equal. So is the book wrong?

To 2nd order, the BCH identity reads
$$e^{\lambda (T+V)/N} = e^{\lambda T/N}\ e^{\lambda V/N} - \frac{1}{2}(\frac{\lambda}{N})^{2} [ T , V ]$$
However, one can prove that, as $N \to \infty$,
$$\left( e^{\lambda (T+V)/N} \right)^{N} = \left( e^{\lambda T/N}\ e^{\lambda V/N} \right)^{N}$$
This helps you to rewrite the Green's function as
$$\langle x | e^{\lambda H} | y \rangle = \langle x | ( e^{\lambda H /N} )^{N}| y \rangle = \langle x | ( e^{\lambda T /N} e^{\lambda V /N} )^{N}| y \rangle ,$$
which leads to the Path Integral when you insert the identity operators
$$\int dx_{j} |x_{j}\rangle \langle x_{j}| = I, \ \ \ \int dp |p \rangle \langle p| = I.$$

 

[atyy]

When I work out the term of order $(\Delta t)^2$, the left hand side gives $\frac{1}{2}(-\frac{i}{\hbar})^2(T^2+TV+VT+V^2)(\Delta t)^2$ but the right hand side gives $\frac{1}{2}(-\frac{i}{\hbar})^2(T^2+2TV+V^2)(\Delta t)^2$. They are not equal. So is the book wrong?

I think you are right and the book is wrong.

 

[Happiness]

However, one can prove that, as $N \to \infty$,
$$\left( e^{\lambda (T+V)/N} \right)^{N} = \left( e^{\lambda T/N}\ e^{\lambda V/N} \right)^{N}$$

But this equation can be simplified to one independent of $N$:

$$e^{\lambda (T+V)} = e^{\lambda T}\ e^{\lambda V}$$

So if it is true as $N \to \infty$, then shouldn't it be true for all values of $N$? But this is not true, so the equation should not be true for any value of $N$?

 

[blue_leaf77]

But this equation can be simplified to one independent of $N$:

$$e^{\lambda (T+V)} = e^{\lambda T}\ e^{\lambda V}$$

So if it is true as $N \to \infty$, then shouldn't it be true for all values of $N$? But this is not true, so the equation should not be true for any value of $N$?

If $T$ and $V$ do not commute, you can't interchange $e^{\lambda T}$ and $\ e^{\lambda V}$.

 

[Happiness]

If $T$ and $V$ do not commute, you can't interchange $e^{\lambda T}$ and $\ e^{\lambda V}$.

So that means
$$\left( e^{\lambda (T+V)/N} \right)^{N} = \left( e^{\lambda T/N}\ e^{\lambda V/N} \right)^{N}$$
is false even as $N \to \infty$?

I think the correct statement should instead be as $N \to \infty$,

$$e^{\lambda (T+V)/N} = e^{\lambda T/N}\ e^{\lambda V/N}$$

Is this correct?

 

[blue_leaf77]

What I implied is that, I don't think you can reduce the RHS of $\left( e^{\lambda (T+V)/N} \right)^{N} = \left( e^{\lambda T/N}\ e^{\lambda V/N} \right)^{N}$ to $\left( e^{\lambda T}\ e^{\lambda V} \right)$ because in doing so, you must have brought $N$ in the outer most to inside, which means you are interchanging some pairs of the two exponential operators in the process.

 

[Happiness]

To 2nd order, the BCH identity reads
$$e^{\lambda (T+V)/N} = e^{\lambda T/N}\ e^{\lambda V/N} - \frac{1}{2}(\frac{\lambda}{N})^{2} [ T , V ]$$

Since (https://en.wikipedia.org/wiki/Baker–Campbell–Hausdorff_formula)

then to the second order, $\ e^Xe^Y=e^{X+Y+\frac{1}{2}[ X , Y ]}$.

So shouldn't it be

$e^{\lambda (T+V)/N} = e^{\lambda T/N}\ e^{\lambda V/N}\ e^{\frac{1}{2}(\frac{\lambda}{N})^{2} [ T , V ]}$?

 

[strangerep]

@Happiness,

Sam has (almost) given you the crucial missing piece in post #7. I'm reasonably sure Sam meant "in the limit as $N\to\infty$". In general, some properties might be true only in the sense of limits, though untrue for finite values of $N$.

I should have also given you the Wiki link to the Lie-Trotter product formula. I.e., $$e^{A+B} ~=~ \lim_{N\to\infty} \Big( e^{A/N} e^{B/N} \Big)^N ~.$$The way your textbook expresses it seems a bit misleading, imho, but the proper Lie-Trotter formula can be applied more successfully in the path integral material.

 

[samalkhaiat]

But this equation can be simplified to one independent of $N$:

$$e^{\lambda (T+V)} = e^{\lambda T}\ e^{\lambda V}$$

So if it is true as $N \to \infty$, then shouldn't it be true for all values of $N$? But this is not true, so the equation should not be true for any value of $N$?

No. You can write
$$e^{H} = (e^{H/N})^{N} ,$$
But
$$(e^{T/N}e^{V/N})^{N} = (e^{T/N}e^{V/N}) \cdots N \mbox{-factors} \cdots (e^{T/N}e^{V/N}) .$$

 

[samalkhaiat]

So that means
$$\left( e^{\lambda (T+V)/N} \right)^{N} = \left( e^{\lambda T/N}\ e^{\lambda V/N} \right)^{N}$$
is false even as $N \to \infty$?

No. It is true and has a name. It is called the Lie-Trotter product formula. You should not jump to a wrong conclusion just because you can not prove it. Do you think I lied to you when I said “we can prove that”?

I think the correct statement should instead be as $N \to \infty$,

$$e^{\lambda (T+V)/N} = e^{\lambda T/N}\ e^{\lambda V/N}$$

Is this correct?

Yes, it is correct because as I said
$$e^{T/N}e^{V/N} - e^{(T+V)/N} = \frac{1}{2N^{2}} [ T , V ] \sim \mathcal{O}(\frac{1}{N^{2}}) . \ \ \ \ (1)$$
So,
$$\lim_{N \to \infty} \left( e^{T/N}e^{V/N} - e^{(T+V)/N} \right) = 0 .$$
But this does not help you in setting up the Path integral representation of the propagator. In fact, we can use this result to prove the Lie-Trotter formula which is essential in deriving the path integral. Let me show you how. Let $A$ and $B$ be two operators, and consider the difference $A^{N} - B^{N}$. By adding and subtracting equal terms, I can write the following identity
$$\begin{equation*} \begin{split} A^{N} - B^{N} =& (AB^{N-1} - B^{N}) + (A^{2}B^{N-2} - AB^{N-1}) \\ & + (A^{3}B^{N-3} - A^{2}B^{N-2}) + (A^{4}B^{N-4} - A^{3}B^{N-3}) \\ & + \cdots + (A^{N} – A^{N-1}B) . \end{split} \end{equation*}$$
This can be rewritten as
$$\begin{equation*} \begin{split} A^{N} - B^{N} =& (A - B ) B^{N-1} + A (A - B ) B^{N-2} \\ & + A^{2} (A - B ) B^{N-3} + A^{3} (A - B ) B^{N-4} \\ & + \cdots + A^{N-1} ( A - B ) . \end{split} \end{equation*}$$
Okay, now take
$$A = e^{T/N}e^{V/N} , \ \ B = e^{(T+V)/N} .$$
But, we know that
$$A - B = \frac{1}{2N^{2}} [ T , V ] \sim \mathcal{O}(N^{-2}) .$$
So, $(A-B) \to 0$ as $N \to \infty$. Now, the above identity consists of $N$ terms each of which has the factor $(A-B)$ which is of order $(1/N^{2})$. Hence, in the limit $N \to \infty$, the difference $A^{N} - B^{N}$ is zero. Thus, we obtain the Lie-Trotter formula
$$\lim_{N \to \infty} \left( e^{T/N}e^{V/N} \right)^{N} = \lim_{N \to \infty} \left( e^{(T+V)/N} \right)^{N} = e^{(T+V)} .$$

 

[samalkhaiat]

...
then to the second order, $\ e^Xe^Y=e^{X+Y+\frac{1}{2}[ X , Y ]}$.

This equation is correct to all orders provided that
$$[ X , [ X , Y ] ] = [ Y , [ X , Y ] ] = 0 .$$
We speak of “orders” when we expand the exponentials.

So shouldn't it be

$e^{\lambda (T+V)/N} = e^{\lambda T/N}\ e^{\lambda V/N}\ e^{\frac{1}{2}(\frac{\lambda}{N})^{2} [ T , V ]}$?

No. Again, if $[ T , V ]$ commutes with both $T$ and $V$, you can write
$$e^{\lambda (T + V)/N } = e^{\lambda T/N} e^{\lambda V/N} e^{- \frac{1}{2}(\frac{\lambda}{N})^{2} [ T , V ] } .$$
Notice the minus sign on the right hand side.
But, when I wrote
$$e^{X+Y} = e^{X}e^{Y} - \frac{1}{2} [ X , Y ], \ \ \ \ (2)$$
I did not assume that $[ X , Y ]$ commutes with both $X$ and $Y$. Equation (2) is an identity up to quadratic terms, i.e., when you expand and keep only the quadratic terms $X^{2},Y^{2},XY$ and $YX$.

 

[Happiness]

No. You can write
$$e^{H} = (e^{H/N})^{N} ,$$
But
$$(e^{T/N}e^{V/N})^{N} = (e^{T/N}e^{V/N}) \cdots N \mbox{-factors} \cdots (e^{T/N}e^{V/N}) .$$

Yes, I realized my mistake when @blue_leaf77 pointed it out earlier in post #12, sorry!