- #1
emilyb1995
- 8
- 0
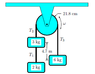
A pulley is massless and frictionless. The
masses 2 kg, 3 kg, and 6 kg are suspended as
in the figure.
(Figure Attached)
What is the tension T1 in the string be-
tween the two blocks on the left-hand side
of the pulley? The acceleration of gravity is
9.8 m/s2 .
Answer in units of N
I'm completely stupped and have no clue where to begin. Just a hint on where to begin would be appreciated.
masses 2 kg, 3 kg, and 6 kg are suspended as
in the figure.
(Figure Attached)
What is the tension T1 in the string be-
tween the two blocks on the left-hand side
of the pulley? The acceleration of gravity is
9.8 m/s2 .
Answer in units of N
I'm completely stupped and have no clue where to begin. Just a hint on where to begin would be appreciated.