High School Why is the use of absolute value in vector norms a matter of preference?

[mech-eng]

I would like to ask you why the author does not use absolute value of y instead of y?

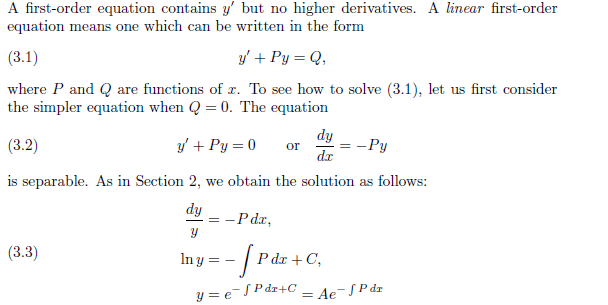
Source: Mathematical Methods in the Physical Sciences by Mary L. Boas

Thank you.

 

[fresh_42]

$y=\pm \exp(-\int P dx +C)=\pm A \exp(-\int P dx)=A' \exp(-\int P dx)$ and the sign can be put into the value of $A$.

 

[mech-eng]

$y=\pm \exp(-\int P dx +C)=\pm A \exp(-\int P dx)=A' \exp(-\int P dx)$ and the sign can be put into the value of $A$.

But in this example in the same source In calculating I, the integrating factor, the minus sign cannot be put into any value. Would you like to examine this?

So shouldn't it be as "3ln|1+ x^2|"

Thank you.

 

[fresh_42]

When is $1+x^2 < 0\,$?

 

[mech-eng]

When is $1+x^2 < 0\,$?

Sorry for the mistake, this expression can be 0 for maximum value.

Thank you.

 

[fresh_42]

I simply assumed real numbers. Over complex numbers, things become a bit more complicated. What is $\ln|x|$, e.g? Usually $x$ refers to a real variable and a complex would be denoted by $z$. At least it explains the examples.

 

[mech-eng]

I simply assumed real numbers. Over complex numbers, things become a bit more complicated. What is $\ln|x|$, e.g? Usually $x$ refers to a real variable and a complex would be denoted by $z$. At least it explains the examples.

But even for complex numbers, cannot be it maximum 0 because i^2=-1 ?

Thank you.

 

[fresh_42]

It could be $1+(2i)^2=-3$ but the complex numbers aren't ordered anymore, so $"<"$ only makes sense for it's real absolute values, which are usually written by double lines $||z||$. Differentiation and integration of complex valued functions must be handled more carefully, because a lot of formulas we're used to, don't apply anymore. E.g. the exponential function in the complex number plane behaves very differently compared to the real version. Here we have $e^{2n \pi i}=1$ for all $n \in \mathbb{N}$ which doesn't have anything near over the reals.

 

[pwsnafu]

so $"<"$ only makes sense for it's real absolute values, which are usually written by double lines $||z||$.

I have never seen double lines for the absolute value of complex numbers. That's reserved for norm.

 

[fresh_42]

What's the difference?

 

[pwsnafu]

Norms are defined on vectors. Absolute values are defined on fields. When you have complex vector spaces, you need the notation separate because the norm will in general be different to the absolute value of vectors.

As an aside, absolute values are related to valuation theory, but as far as I'm aware of norms don't have an analogue.

 

[fresh_42]

$|x+iy|^2=x^2+y^2$ is a norm, the Euclidean norm of $(x,y)$.

 

[pwsnafu]

$|x+iy|^2=x^2+y^2$ is a norm, the Euclidean norm of $(x,y)$.

There is a difference between the field $\mathbb{C}$, the one dimensional vector space of the complex numbers over itself $\mathbb{C}^1$, which in turn is a different thing to $\mathbb{R}^2$. They are equivalent as vector spaces, but his thread is not about complex vector spaces.
The reason why we notationally separate the two, is that there is no requirement that the vector space's norm be the Euclidean one.

 

[fresh_42]

Yes, I know, but it's how the "absolute value" of a complex number is defined, by a vector norm. Therefore the double lines make sense here. That's all I wanted to say. Do you define $|x+iy|$ differently?

 

[pwsnafu]

Yes, I know, but it's how the "absolute value" of a complex number is defined, by a vector norm. Therefore the double lines make sense here. That's all I wanted to say. Do you define $|x+iy|$ differently?

The part in bold is what I disagree with.

 

[fresh_42]

A matter of taste. But as it is a vector norm you somehow contradict yourself. Anyway, I'm sure that both notations are actually used, so there's no reason to argue about it.